Question
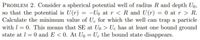
Transcribed Image Text:PROBLEM 2. Consider a spherical potential well of radius R and depth Uo,
so that the potential is U(r) = -Uo at r < R and U(r) = 0 at r > R.
Calculate the minimum value of Uc for which the well can trap a particle
with l = 0. This means that SE at Uo > Uc has at least one bound ground
state at l = 0 and E < 0. At Ug = Uc the bound state disappears.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 5 steps

Knowledge Booster
Similar questions
- A one-dimensional infinite potential well has a length of 2L. i) What are the energy eigenvalues? ii) Calculate the ground state energy if ten protons are confined in the box. Assume that the protons don't interact with each other. iii) If the ten protons are replaced by ten neutral hydrogen atoms, what is the total ground state energy resulting from the confinement? Again, assume that the hydrogen atoms do not interact with each other. You can treat the mass of proton and hydrogen atom to be identical.arrow_forwardWhat is the ground-state energy of (a) an electron and (b) a proton if each is trapped in a one-dimensional infinite potential well that is 273 pm wide? (a) Number 8.083824566 Units eV (b) Number 4.401408127 Units eVarrow_forward6QM Please answer question throughly and detailed.arrow_forward
- a question of quantum mechanics: Consider a particle in a two-dimensional potential as shown in the picture Suppose the particle is in the ground state. If we measure the position of the particle, what isthe probability of detecting it in region 0<=x,y<=L/2 ?arrow_forwardProblem 1 An electron is described by the wave function with the following x dependence: (x) = 0 for a 0, where C is a constant. (a) Determine the value of C is the wave-function is normalised. (b) Where is the electron most likely to be found? (c) Calculate the expectation value of position for the electron. (d) What is the uncertainty in finding the position of the electron?arrow_forwardA particle with mass m is in the state .2 mx +iat 2h Y(x,t) = Ae where A and a are positive real constants. Calculate the expectation values of (x).arrow_forward
- a) Show that if the total energy ε of a single particle state can be written as the sum of independent energies EiA, εiB, εic... then its partition function will factorise into a product of partition functions ZAZBZC. b) Given the factorisation, show how the free energy F and quantities such as S and Cy can be expressed as a sum of terms dependent on the sources A, B, C.arrow_forwardConsider an atomic nucleus to be equivalent to a one dimensional infinite potential well with L = 1.4 x1014 m, a typical nuclear diameter .What would be the ground-state energy of an electron if it were trapped in such a potential well? (Note: Nuclei do not contain electrons.)arrow_forward
arrow_back_ios
arrow_forward_ios