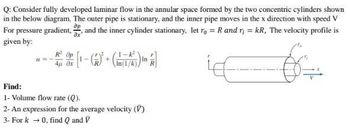
Q: Consider fully developed laminar flow in the annular space formed by the two concentric cylinders shown in the below diagram. The outer pipe is stationary, and the inner pipe moves in the x direction with speed V For pressure gradient, and the inner cylinder stationary, let ro = R and r = KR, The velocity profile is given by: 4p x Find: 1-k In(1/k) 1- Volume flow rate (Q). 2- An expression for the average velocity (V) 3- For k 0, find Q and V

4) A constriction in a pipe will cause the velocity to rise and the pressure to fall at section 2 in the throat. The pressure difference is a measure of the flow rate through the pipe. The smoothly necked-down system shown in Figure is called a venturi tube. Find an expression for the mass flux in the tube as a function of the pressure change. (1) HGL 4²² (2)

a. a long rectangle of constant area A containing an irregu- larly shaped obstacle (figure 2). The upstream flow into the tube is u1 = (U, 0). Determine the flow at point 2, far downstream of the obstacle. b. now write down steady conservation of momentum in integral form, taking a simple control volume including points (1) and (2). Include pressure forces and horizontal drag from the particle but ignore viscosity. c. thus, determine the value of the drag force for an arbitrary irregular shaped obstacle in an inviscid, incompressible, irrotational fluid flow.

A Newtonian fluid is flowing in the narrow gap between two infinite parallel horizontal plates due to the movement of the top plate (see the figure below). The top plate is moving at a speed U from left to right, and the bottom plate is stationary. Consider the flow is steady, incompressible, and laminar. The distance between two plate is H, and the gravity acts in negative z-direction (which is perpendicular into the page). There is an applied constant pressure gradient of apJəx in the x-direction. Calculate the velocity and pressure fields. Plot typical velocity profiles in dimensionless form for a pressure gradient value of 0 and -15. In your solution show the conceptual diagram of the problem; write all relevant assumptions and boundary conditions; show the detailed steps of the problem solutions including equations and detailed processing of those equations. Moving plate Fluid: p.H Fixed plate P2-P1

yes it is hard questoin that is why I am looking for help

= = Air flows radially outward between the two parallel circular plates shown in the figure below. The pressure at the outer radius, Ro 5 cm, is atmospheric. Find the pressure at the inner radius R¿ 0.5 cm, if the air density is constant, the air flow is inviscid, the volume flow rate is 4.0 L/s, and the plate spacing is h = 0.125 cm. h Po= Patm = 101.3 kPa T = 20°C 777 R₁ Ra

Using the figure depicted below, perform the following: (a) Calculate the minimum height h at which cavitation occurs. Indicate on the figure where the cavitation occurs. (b) Calculate the volume flowrate (in m³/s) if h = 25 cm. Be sure to state all assumptions. Note that section 2 opens to atmosphere. D1 = 5 cm h D2 = 10 cm Free Jet (1) 2 Water at 20°C

A hydraulic pump is shown in the following Figure, flow rate is 25 L/min, the diameter of suction pipe is 25mm, and the suction port of pump is higher than the oil surface of tank, the height is h, Kinematic viscosity of oil is 30×10“m²/s, density of oil is 900kg/m³. Only the pressure loss along the straight suction pipe with length h is 75 considered, if the vacuum degree at the inlet of pump is not bigger than 4500P ( 2 = R. a = 2, g=9.8) (1) Calculate the flow velocity in the suction pipe; (2) Judge the flow state in suction pipe; (3) Calculate the pressure loss along the straight suction pipe; (4) Calculate the oil absorption height h.