
Concept explainers
Part a and part d are both complete, see attached images.
Problem 10: Consider standing waves in the column of air contained in a pipe of length L = 6.5 m. The speed of sound in the column is vs = 348 m/s.
Each of the standing wave images provided may represent a case for which one or both ends are open.
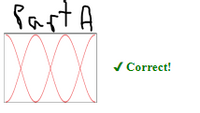
Part (a) Select the image from the options provided showing the displacement of the air molecules in the third harmonic of a pipe with two open ends.
Part (b) Calculate the wavelength λ3, in meters, for the third harmonic in the pipe with two open ends.
Part (c) Calculate the frequency f1, in hertz, for the fundamental harmonic in the pipe with two open ends.
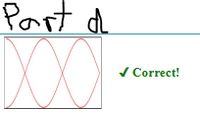
Part (d) Select the image from the options provided showing the displacement of the air molecules in the third mode of a pipe with one open end and one closed end. (The third mode is the second excitation above the fundamental.)
Part (e) Calculate the frequency f1, in hertz, for the fundamental harmonic in the pipe with one open and one closed end.
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps

- Problem 1: The figure below shows four identical point sources of sound that are uniformly spaced on an x-axis. Sq d- d S3 d S4 P The sources emit sound at the same wavelength X and same amplitude A, and they emit in phase. A point P is shown on the x axis. Assume that as the sound waves travel to P, the decrease in their amplitude is negligible. What multiple of A is the amplitude of the net wave at P if distance d in the figure is (a) X/4, (b) A/2, and (c) X?arrow_forwardQuestion in attachmentsarrow_forwardDo the following on paper, scan or photograph your work, and submit it. You have a room that is 4 m long, 7 m wide, and has a ceiling height of 3 m. The floor is hardwood, = .15 at 500 Hz. The walls are gypsum wallboard (GWB), = .30 at 500 Hz. The ceiling is acoustic ceiling tile (ACT), = .95 at 500 Hz. What is the mean free path in this room?arrow_forward
- 0.0058 kg/m is fixed at both ends. A 5.0-kg mass is hung from A string with a linear mass density of the string, as shown. If a pulse is sent along section A, what is the wave speed in section A and the wave speed in section B? %D B. A FA 35° 5 kg Image Description VA m/s VB = m/s B.arrow_forwardConsider standing waves in a pipe of length L = 3.5 m containing a column of air. The speed of sound in the columns is vs = 334 m/s. Each of the standing wave images provided may represent a case for which one or both ends are open. Select the image from the options provided showing the displacement of air particles for the third harmonic of a pipe with two open ends. Calculate the wavelength λ3 in m for the third harmonic in the pipe with two open ends. Calculate the frequency f1 in Hz for the fundamental harmonic in the pipe with two open ends.arrow_forwardWe will assume that a sound wave, once generated and emitted, will propagate uniformly in all directions of 3-dimensional space. Find an expression for the displacement s(r, t) of the air particles from equilibrium, where r is the radial distance from the source, and t is time. Introduce physical constants/coefficients, with appropriate physical dimensions, when necessary.arrow_forward
- Consider a sound wave in air that has displacement amplitude 2.40X10-² mm. For related problemsolving tips and strategies, you may want to view a Video Tutor Solution of Amplitude of a sound wave in air. Part A Calculate the pressure amplitude for frequencies of 158 Hz . Take the bulk modulus of the air to be 1.42 × 105 Pa and the speed of sound to be 344 m/s Express your answer in pascals. pmaz = |98.30 Part B Submit Previous Answers Request Answer Be x Incorrect; Try Again; 4 attempts remaining pmaz = |983 Calculate the pressure amplitude for frequencies of 1580 Hz. Take the bulk modulus of the air to be 1.42 × 105 Pa and the speed of sound to be 344 m/s. Express your answer in pascals. Part C ? Be pmax = |9830 Pa Submit Previous Answers Request Answer (3 ? x Incorrect; Try Again; 5 attempts remaining Re 6 Calculate the pressure amplitude for frequencies of 1.58x104 Hz . Take the bulk modulus of the air to be 1.42 × 105 Pa Express your answer in pascals. Pa #3 ? Pa Submit Previous…arrow_forwardThe speaker depicted below (See slide 10 of chapter 16 for a detailed explanation) emits a sound of frequency 779 Hz. Due to the path difference (r2 - r1) the receiver detects a constructive interference. Find the distance by which r2 needs to be adjusted to detect the very next destructive interference. (Take speed of sound as 343 m/s).arrow_forward
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON





