Let G be a finite group of order 56. a) Determine the possible number of Sylow 7-subgroups in G. Provide a detailed justification using Sylow's theorems. b) Assume that G has exactly one Sylow 7-subgroup. Prove that this Sylow 7-subgroup is normal in G, and subsequently show that G contains a normal subgroup of order 8. c) Using the results from parts (a) and (b), classify all possible group structures for G. Discuss whether G is necessarily abelian or non-abelian based on your classification. 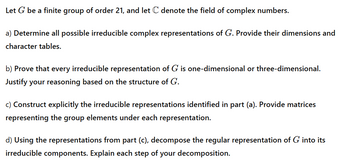Let G be a finite group of order 21, and let C denote the field of complex numbers. a) Determine all possible irreducible complex representations of G. Provide their dimensions and character tables. b) Prove that every irreducible representation of G is one-dimensional or three-dimensional. Justify your reasoning based on the structure of G. c) Construct explicitly the irreducible representations identified in part (a). Provide matrices representing the group elements under each representation. d) Using the representations from part (c), decompose the regular representation of G into its irreducible components. Explain each step of your decomposition.

Prove that the set of all complex numbers that have absolute value forms a group with respect to multiplication.

Need detailed solutions to each parts as ap

under multiplication and let G be the group of all real 2x 2 matrices of -- Let G be the group of all non-zero complex numbers under multiplication and let G be the eroup of all real 2× 2 matrices of a b -b a the form where a and b are not both zero under matrix nlir plication. Show that G and G are isomorphic by exhibiting an isomorphism of G onto G.