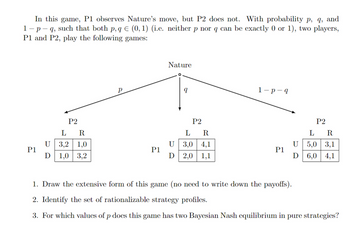
In this game, P1 observes Nature's move, but P2 does not. With probability p, q, and 1-p-q, such that both p, q = (0, 1) (i.e. neither p nor q can be exactly 0 or 1), two players, P1 and P2, play the following games: p Nature 1-p-q P2 P2 P2 L R L R L R U 3,2 1,0 U 3,0 4,1 U 5,0 3,1 P1 P1 P1 Ꭰ 1,0 3,2 D 2,0 1,1 D 6,0 4,1 1. Draw the extensive form of this game (no need to write down the payoffs). 2. Identify the set of rationalizable strategy profiles. 3. For which values of p does this game has two Bayesian Nash equilibrium in pure strategies?

A small community has 10 people, each of whom has a wealth of $3,000. Each individual must choose whether to contribute $300 or $0 to the support of public entertainment for their community. The money value of the benefit that a person gets from this public entertainment is one half of the total amount of money contributed by all individuals in the community. This game has a Nash equilibrium in which 5 people contribute $300 and for public entertainment and 5 people contribute nothing. This game has a dominant strategy equilibrium in which nobody contributes anything for public entertainment. This game has no Nash equilibrium in pure strategies, but has a Nash equilibrium in mixed strategies. This game has a dominant strategy equilibrium in which all 10 citizens contribute $300 to support public entertainment. This game has two Nash equilibria, one in which everybody contributes $300 and one in which no- body contributes $300.

help please answer in text form with proper workings and explanation for each and every part and steps with concept and introduction no AI no copy paste remember answer must be in proper format with all working

If the players play pure strategies, the game has no Nash equilibrium. But what if they choose their moves randomly? Let each player instead opt for a mixed strategy instead of a pure strategy. The first will play action Z with probability p, and the second will play action L with probability q. At which pair (p, q) are the mixed strategies of the players in equilibrium? At which pair (p, q) does neither player want to change strategy? When are both strategies simultaneously the best response?

Based on the following game, what are the secure strategies for player 1 and player 2? Player 1 S1 and 12 S1 and t1 S2 and 12 S2 and 11 s1 S2 Player 2 tl 10,15 -10,7 t2 15,8 10,20

Player 1 and Player 2 will play one round of the Rock-Paper-Scissors game. The winner receives a payoff +1, the loser receives a payoff -1. If it is a tie, then each player receives a payoff zero. Suppose Player 2 is using a non-optimal strategy: he/she plays Rock with probability 35%; Paper with probability 45%; and Scissors with probability 20%. Given that Player 1 knows Player 2 is using this strategy, the best response of Player 1 is to play O a mixed strategy with probability 1/3 each (Rock, Paper and Scissors) O Scissors with probability 100% O any strategy (any strategy is a best response for Player 1) O Rock with probability 100% O Paper with probability 100%

Q

Consider the following game. Firm 1 can implement one of two actions, A or B. Firm 2 observes the action chosen by Firm 1 and then decides whether to fight it or not. (-10, 20) F2 Fight A Don't fight -(30, 10) Firm 1 (-10, 0) Fight B Don't fight -(20, 15) (a) Consider the following strategy profile: Firm 1 chooses A; Firm 2 chooses fight if A, and fight if B. • this strategy profile is [Select] (b) Consider the following strategy profile: Firm 1 chooses B; Firm 2 chooses fight if A, and don't fight if B. • this strategy profile is [Select] O (c) Consider the following strategy profile: Firm 1 chooses B; Firm 2 chooses don't fight if A and don't fight if B. • this strategy profile is [Select] 00 F2 ()

A game involves two players: player A and player B. Player A has three strategies a1, a2 and a3 while player B has three strategies b1, b2 and b3.       Player B         b1 b2 b3     a1 -40,30 70,20 -10,120   Player A a2 40,60 80,80 60,20     a3 -30,40 -50,110 150, -70               Assuming that this is a one-time game, answer the following questions: Is there any dominant strategy for each player? What is the secure strategy of each player. What is the Nash equilibrium of the game?

8) Find the mixed strategy Nash equilibrium of the following normal form game. Player 2 T1 T2 T3 2, 3 3, 5 1, 1 Player 1 S2 1, 4 4, 3 0, 5 Player 1 attaches probability (S1, S2) = () and Player 2 attaches probability (T1, T2, T3) = ( ) Player 1 attaches probability (S1, S2) = (.) and Player 2 attaches probability (T1, T2, T3) = (qi, 42, 1 – q1 – 92) where q1 , and 0 < q2 S %3D Player 1 attaches probability (S1, S2) = (G,;) and Player 2 attaches probability (T1, T2, T1) = (qı.42, 1 – q1 – 42) where 0 < qi <, and q2 = 3. Player 1 attaches probability (S1, S) = (;, -) and player 2 attaches probability (T1, T2, T3) = (1.42, 1- q1- 42) where 0 s qı s and q2 =