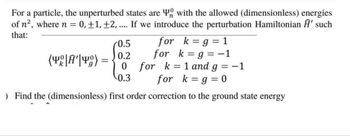
For a particle, the unperturbed states are with the allowed (dimensionless) energies of n², where n = 0, +1, +2, .... If we introduce the perturbation Hamiltonian Ã' such that: (YA'YO) = 0.5 0.2 0 0.3 for k=g = 1 for k =g = −1 for k = 1 and g = -1 for k=g=0 ) Find the (dimensionless) first order correction to the ground state energy

Consider the 2D harmonic oscillator Hamiltonian: 87 - 12/24 (13² + 8² ) + 2 ²³² (1² + 8² ) Ĥ mw² 2m Unless otherwise specified, we will work in the eigenstates that satisfy: Ĥ|nz, ny) = Enz,ny |nx, ny) x, with Eng,ny = ħw(nx + Ny + 1). (a.) Some energy levels are degenerate. For example, E 2ħw can be achieve with (nx, ny) = (1, 0); (0, 1). This energy level has a degeneracy D(2ħw) = 2. What is the degeneracy of energy level E (where N is a positive integer)? = Nhw (b.) Consider the state (0)) = √ (12,0) + 2 |1, 1) + (0,2)). (c.) Calculate (Ĥ), (px), (py), and (âŷ) for the state above. = What is (t)) at a later time t > 0?

2) ( Consider an electron trapped in a one-dimensional anharmonic potential. The Hamiltonian for this system is given as: H mw?£? + 2m + B&3 . Regarding the cubic term as being small, apply non- degenerate perturbation theory by letting the perturbation be: A, = ß2³. а) Calculate to first order the ground state energy of this anharmonic oscillator. b) ( | Calculate to second order the ground state energy of this anharmonic oscillator. c) ( Find the lowest order correction to the ground state wave function.

The three matrix operators for spin one satisfy sz Sy – Sy 8z = isz and cyclic permutations. Show that s = sz, (sz tisy )³ = 0. For the sane in, m, can have the values im, m – 1, .., -m, while A12 has eigenvalue m(m + 1). Thus M? = m(m + 1) ±2 5 x 1 = 5 times each once 6 15 4 x 8 = 32 times +3/2 ±1/2 3/2 each 8 times 4 ±1 3 x 27 = 81 times 1 each 27 times 3 2 x 18 = 96 times 1/2 ±1/2 each 48 times 1 × 12 = 42 times 0, each 42 times Total 256 eigenvalues A certain state | 4) is an eigenstate of L? and L,: L'|v) = 1(l+ 1) h² |»), mh|v) . For this state calculate (La) and (L²).