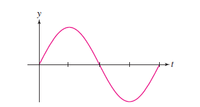
Figure shows the height y of a mass oscillating at the end of a spring, through one cycle of the oscillation. Sketch the graph of velocity as a function of time.

The spring is 5 cm at its natural length. When at equilibrium (e.g. see figure) its length becomes 50 cm. The cart’s mass is 273 gr and the hanging weight is 300 gr. The cart and weight are set into oscillation. Derive the theoretical period as a function of the two masses and the spring constant (no air resistance or friction). Show methodology and discrete steps.

The oscillatory movement of a simple pendulum is a characteristic of the regular repetition of displacements around an equilibrium position. The pendulum swings due to the restored force of gravity, which seeks to bring the mass back to the equilibrium point. The oscillatory behavior is described by a trigonometric solution, which relates the pendulum's position to time, considering its amplitude, frequency and initial phase. Statement: A simple pendulum moves according to the following question:y(t) = A.sin(ωt + φ)In this question, y(t) is the horizontal position of the pendulum, A is its amplitude, ω is its angular velocity, given by ω = 2πf, and φ is the initial phase of the movement, in radians. Since the initial phase of the movement is equal to 0 and its angular velocity is π/2 rad/s, the oscillation frequency of this pendulum is correctly given by the alternative: a) 2.0Hz b) 1.5Hz c) 1.0Hz d) 0.5Hz e) 0.25Hz

Determine the conditions under which mechanical energy is conserved in a pendulum. Consider a situation in which a pendulum made from a wooden sphere. The mass of the pendulum is 100 g and the length 1 m. If it is hanging on the ceiling of a room, it is made to oscillate. What, if anything, is the mechanical energy of the pendulum conserved? Make the argument grounded in physics.

In the figure provided, two boxes oscillate on a frictionless surface. The coefficient of static friction between the two boxes is 0.440 and the spring constant in the spring is 54.5 N/m. If the smaller box has a mass of 3.51 kg and the larger box has a mass of 8.47 kg, what is the maximum oscillation amplitude for which the 3.51 kg box does not slip?

Determine the theoretical equation for the dependence of Period of the Physical Pendulum to location of the pivot point for a solid bar of length L. This will be T(x) - Period as a function of pivot point position. Mathematically determine minima of the function T(x).

The figure shows the top view of an object of mass m sitting on a horizontal frictionless surface, connected to two walls by two stretched rubber bands of length L. The tension in each rubber band is T when the object is stationary. Find an expression for the frequency of oscillations perpendicular to the rubber bands. Assume that the amplitude of oscilla- tions is sufficiently small such that the tension in the rubber bands is essentially unchanged as the object oscillates. Use small angle approximations to express your answer only in terms of the variables m, L and T. Rubber bands

a particle of mass m makes simple harmonic motion with force constant k around the equilibrium position x = 0. Calculate the valu of the amplitude and the phase constant if the initial conditions are x(0) = 0 and v(0) = -Vi (vi > 0). Draw x-t, v - t and a - t figures for one complete cycle of oscillations.

A spring is attached to the ceiling and pulled 7 cm down from equilibrium and released. The amplitude decreases by 12% each second. The spring oscillates 17 times each second. Find an equation for the distance, D the end of the spring is below equilibrium in terms of seconds, t. D(t) =

A pendulum is formed by placing a horizontal axis at various positions of a measuring stick of length 108 cm. What is the period in seconds of the pendulum when the axis is placed at the 81.0-cm mark?