What is simple harmonic motion (SHM)?
Simple harmonic motion is a type of periodic motion in which an object undergoes oscillatory motion. The restoring force exerted by the object exhibiting SHM is proportional to the displacement from the equilibrium position. The force is directed towards the mean position. We see many examples of SHM around us, common ones are the motion of a pendulum, spring and vibration of strings in musical instruments, and so on.
Introduction to simple harmonic motion
Simple harmonic motion is a special type of periodic motion in which an object oscillates about an equilibrium position. Consider a simple pendulum in which the bob is hung using a string. When the mass is displaced from the rest position, the mass undergoes oscillation. This is an example of simple harmonic motion.
It is important to understand that not all periodic motions are simple harmonic but all simple harmonic motions are periodic motion. Consider the example of motion of the earth around the sun, since earth takes eliptical orbit around the sun there is no equilibrium position. Hence, the revolution of earth is not simple harmonic motion though it is periodic.
Periodic motion
A type of motion that is repeated after a certain amount of time is called periodic motion. e.g. The motion of the earth around the sun and the motion of a pendulum.
When an object undergoes periodic motion, it takes some time to reach the initial state. This time interval is called the period denoted by T. Period is measured in seconds. The reciprocal of the period is called frequency which is denoted by ν.
T=1/ν( in seconds)
The SI unit of frequency is hertz (Hz).
Spring-mass oscillator
In this module, we study the dynamics of spring-mass oscillators using Hooke’s law. Consider a block of mass m which is attached to the one end of spring. As shown below, let x be the displacement, x=0 be the initial position.
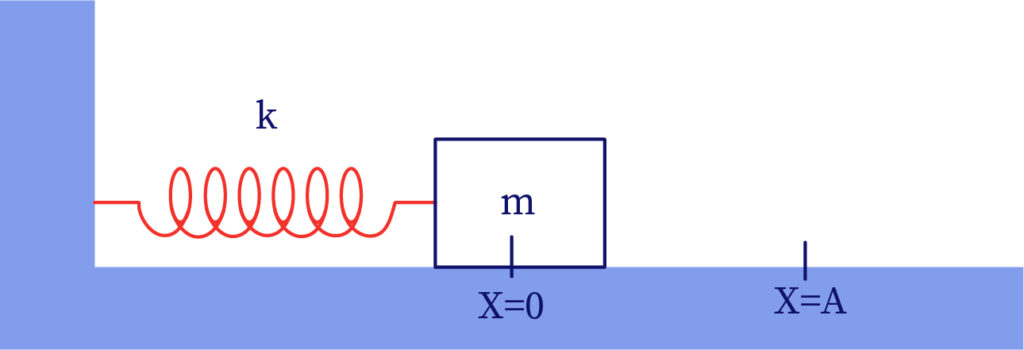
When the block is moved to a certain position say x=A if the block is released from position A, the block is accelerated towards the initial position as the spring is subjected to a restoring force. Hooke’s law states that stress is directly proportional to strain. The expression for force is as follows:
(1)
Where k is the spring constant.
The right-hand side of equation (1) is negative. Because, if we move the block to some position say +x direction, the restoring force acts in the -x direction.
According to Newton’s second law, the force is,
Where a is the acceleration.
The above equation can be written in the form,
(2)
Using equations (1) and (2),
Since k and m are constants, we can put,
(4)
Therefore, equation (3) becomes,
(5)
The solution of equation (5) is given as follows,
(6)
Where B and C are constants.
To find B and C using initial conditions
Let us find the physical significance of constant B using the initial condition t=0.
The equation (6) becomes,
[since and ]
Put,
Here, is nothing but the starting position.
Let us differentiate the equation (6),
[since ]
Now put t=0, we get,
put
or
Where is the initial velocity.
Hence, the solution becomes,
(7)
Where ω is the angular frequency. It is given by,
Now we can further simplify our equation using,
Where A and φ are constants.
Therefore, equation (7) becomes,
or (8)
The following graph shows the variation of displacement as time flows,
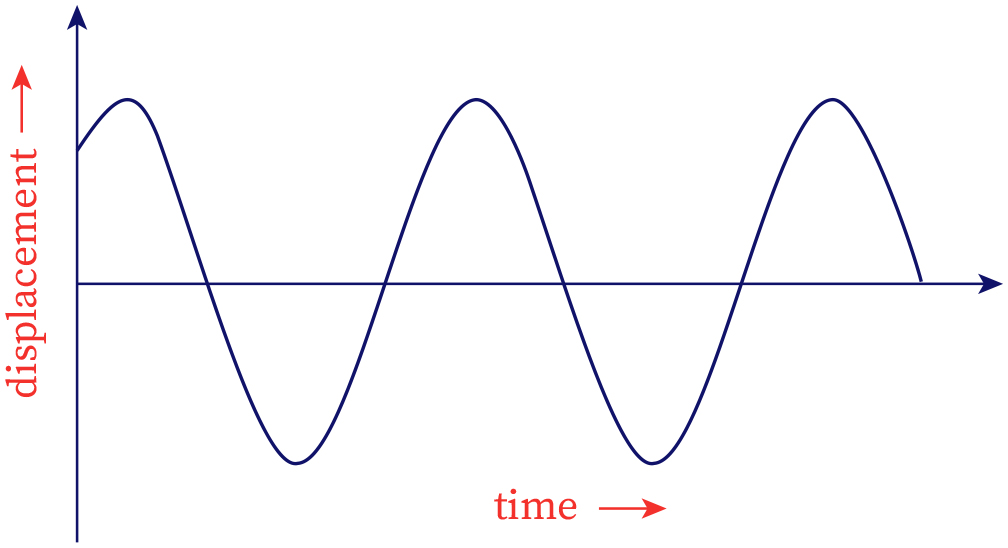
The quantity A is the amplitude i.e. the maximum displacement and φ is the initial phase.
Expression for angular frequency
Assume, that the block was at rest initially i.e. x=0 at t=0.
After time T, the block returns to the original position i.e. x=0 at t=T.
Similarly, at any time t=nT , x=0 where n is any positive integer i.e. n=0,1,2,…
Equation (8) becomes,
Where v is the frequency (measured in Hz).
Note: In our spring-mass oscillator example, the mechanical energy remains constant as the system oscillates which means neither energy is lost nor gained. These types of oscillations are called undamped oscillations. In practice, the mechanical energy does not remain constant. There may be a loss of energy due to friction or any other parameter. As the amplitude of block undergoing oscillation decreases as mechanical energy decreases hence, they are called damped oscillations. The damped oscillations are shown graphically as below.
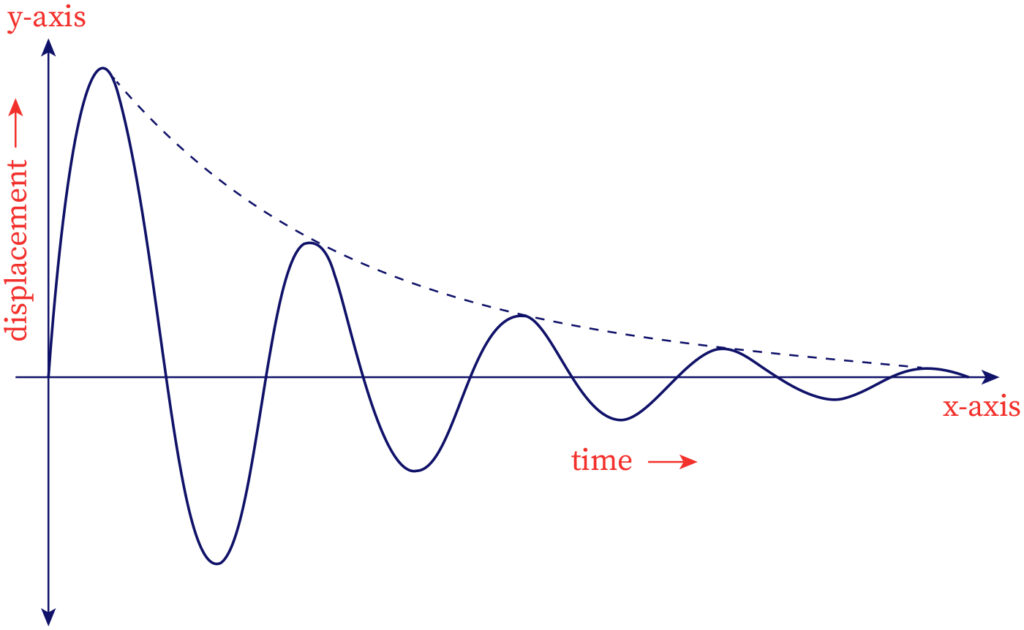
Important observations
- The period for a given spring-mass system is constant. The magnitude of displacement does not alter the value of the period.
- The period depends only upon two factors. The nature of the spring and the mass of the block used are the two factors that affect the period of oscillations.
- The restoring force depends upon the displacement. As the net displacement is zero at the equilibrium position, the force at this position is also null. The force and acceleration are maximum when the displacement is maximum.
- In contrast, the velocity at extreme position is zero, at equilibrium position the velocity attains its maximum value.
- The net mechanical energy remains constant if there is no frictional force.
Simple pendulum
A simple pendulum is another example that exhibits simple harmonic motion. The simple pendulum consists of a bob of certain mass suspended using a massless string. In a simple pendulum, a gravitational force acts as restoring force. The bob undergoes angular displacement.
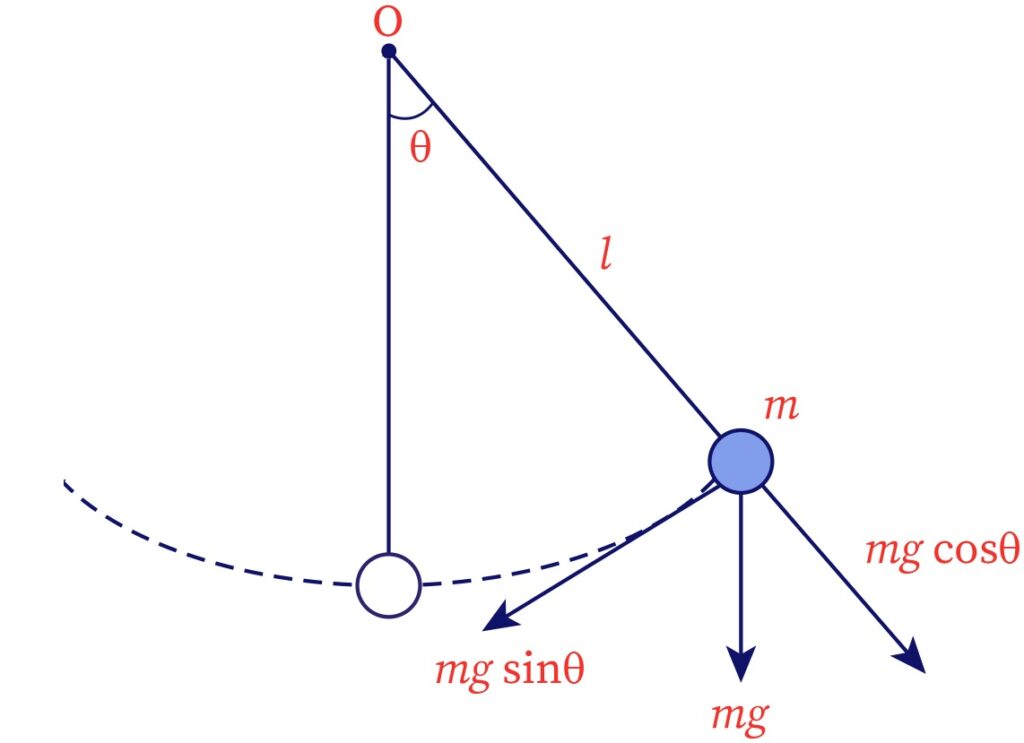
Consider a bob that is suspended using a string with almost zero mass and of length l. Let m be the mass of the bob. When the bob is displaced to a certain angle θ, the bob undergoes simple harmonic motion. The tangential component of gravitational force acts as restoring force.
The gravitational force on mass m is,
Where g is the acceleration due to gravity.
The force can be resolved into tangential and radial components.
The tangential component is,
(9)
Let us use newton’s law to obtain the dynamics of a simple pendulum.
We have,
or (10)
Comparing equations (9) and (10) we get,
[ since for small angles] (11)
Equation (12) is similar to equation (5).
Hence the solution can be given as,
Where is called amplitude, ω is the angular frequency and φ is the initial phase.
Observations
- Unlike the spring-mass system, the mass of the bob does not affect the frequency of oscillation. The period depends upon the length of the string only.
- if friction is absent, the mechanical energy remains constant.
- Other parameters like force, acceleration, and velocity changes are similar to the spring-mass system.
Formulas
The displacement of the pendulum is,
Where A is the amplitude.
Angular frequency of the spring-mass system is given by,
or
Where k is the spring constant (in N/m).
The angular displacement is expressed as,
Where is the amplitude of the simple pendulum.
The angular frequency of the simple pendulum is given as,
(in radians per second),
Where g is the acceleration due to gravity.
Context and applications
This topic is significant in physics for both undergraduate and graduate courses, especially for
Bachelors of science (Physics)
Masters of science (Physics)
Bachelors of technology (Electrical engineering).
Practice problems
Question 1: What is the reciprocal of the period?
(a) Angular frequency
(b) Frequency
(c) Amplitude
(d) Velocity
Answer: Option (b) is correct.
Explanation: Frequency is the measure of repetition of motion per unit time. Hence the reciprocal of the period is frequency so the correct answer is (b) frequency.
Question 2: Which of the following is an example of simple harmonic motion?
(a) Simple pendulum
(b) Revolution of the earth around the sun
(c) Rotation of windmills
(d) Car moving in a straight line
Answer: Option (a) is correct.
Explanation: For any motion to be simple harmonic the two conditions are the motion should repeat after some time and there will be an equilibrium position. The motion of the simple pendulum is about an equilibrium position and the motion is repeated; hence the correct option is (a) simple pendulum.
Question 3: For a damped simple harmonic motion, the quantity that decreases over time is _____.
(a) Frequency
(b) Phase
(c) Amplitude
(d) Velocity
Answer: Option (c) is correct.
Explanation: The amplitude of the damped oscillation reduces with time as the energy is lost to the surroundings. The energy is the only parameter that affects the oscillatory motion.
Hence, option (c) is the correct answer.
Question 4: At the equilibrium position, the following quantity is maximum in ____.
(a) Potential energy
(b) Phase
(c) Acceleration
(d) Velocity
Answer: Option (d) is correct.
Explanation: The velocity is maximum at the equilibrium position. The acceleration is zero at the equilibrium position but the kinetic energy is maximum at this position, hence the velocity is maximum. Thus, option (d) is the correct answer.
Question 5: Which of the quantity affects the frequency of oscillation of the pendulum?
(a) Mass of the bob
(b) Length of the string
(c) Initial displacement
(d) Nature of string used
Answer: Option (b) is correct.
Explanation: The angular frequency is given by,
From this equation, it is clear that the only length of the string affects the frequency.
Hence, option (b) is the correct answer.
Want more help with your physics homework?
*Response times may vary by subject and question complexity. Median response time is 34 minutes for paid subscribers and may be longer for promotional offers.
Simple harmonic motion Homework Questions from Fellow Students
Browse our recently answered Simple harmonic motion homework questions.