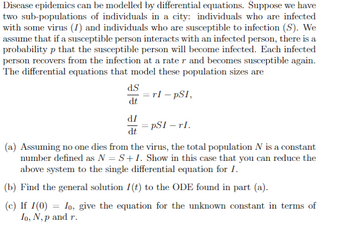
Disease epidemics can be modelled by differential equations. Suppose we have two sub-populations of individuals in a city: individuals who are infected with some virus (I) and individuals who are susceptible to infection (S). We assume that if a susceptible person interacts with an infected person, there is a probability p that the susceptible person will become infected. Each infected person recovers from the infection at a rate r and becomes susceptible again. The differential equations that model these population sizes are TI - PSI, dS dt dI dt pSI - TI. (a) Assuming no one dies from the virus, the total population N is a constant number defined as N = S+I. Show in this case that you can reduce the above system to the single differential equation for I. (b) Find the general solution I(t) to the ODE found in part (a). = (c) If I(0) Io, give the equation for the unknown constant in terms of Io, N, p and r.

Consider the following linear system of differential equations. Y - 324y2 Which of the following is the general solution to this system? e108x, -108x (C) c1 e108 -108x (A) c1 (B) c1 +c2 e$4x. -54x (F)c e108r (D) c (E) c1 -108x (G) c1 e108x -108x (H) c1 -54x + C2

You are given a set of data (xj, Yi) for linear regression analysis and find out these data are not linearly related by plotting (x¡, Yi) with graphical analysis. After using log transformation of data from (x¡ , yi) to (X¡ = log X¡ , Y¡ = log yi ) and a linear fitting line were derived, Y = A + BX, for the power-law relationship y = k x". You tried to find the power-law equation's kand n by transforming back from A and B in that linear relation equation Y = A + BX. Which one of the below can be the correct result? There might be more than one correct answer; please select all of the correct answers. O logA=k O n= logB O 10A=K O en=B n = B O A=logK O eK=A O eB=n

Can you find all equilibria of each system of differential equations and use the analytical approach to determine the stability of each equilibrium? dx1/dt= 4x1(1-x1)-2x1x2 dx2/dt= x2(2-x2)-x2

2 Problem 2. Sketch a proof of these formulas for linear regression, described on the following page. P;(x1, y1) y = mx + b P2(x2, y2) w = (mx + b – y)² + ... + (mxr, + b – (1) (See the accompanying figure.) Show that the values of m and b that do this are (E»)(E») - »>»» (E.) - »>«? т (2) b= Σχ (3) - m

Clean Fossil Fuels. In the article, “Squeaky Clean Fossil Fuels” (New Scientist, Vol. 186, No. 2497, p. 26), F. Pearce reported on the benefits of using clean fossil fuels that release no carbon dioxide (CO2), helping to reduce the threat of global warming. One technique of slowing down global warming caused by CO2 is to bury the CO2 underground in old oil or gas wells, coal mines, or porous rocks filled with salt water. Global estimates are that 11,000 billion tonnes of CO2 could be disposed of underground, several times more than the likely emissions of CO2 from burning fossil fuels in the coming century. This could give the world extra time to give up its reliance on fossil fuels. The following bar chart shows the distribution of space available to bury CO2 gas underground. a. Explain why the break is found in the third bar. b. Why was the graph constructed with a broken bar?

a) A Multiple Linear Regression Model in the form provided in Equation 1 is required to predict the amount of trips likely to be generated in the Bortianor vicinity in 2040. Y=a+ajX1+æXz+æX3 (Equation 1) where Y= trips/household to work X1= number of cars owned by household X2 = income of household X3 = household size ao, ai, æ and a3 =constants Using the corelation matrix obtained from a base year survey in Table 5, detemine all the possible equations that can be fomulated in the fom of Equation 1 out of which the best predictive model will be selected. Give reasons for your choice of equations. Assume all the independent variables are linearly related to the dependent variable and eachindependent variable is easily projected. Table 5: Comrelation matrix Trips Household size Car Income ownership Trips Car ownership Income Household size 1 0.65 1 0.90 0.89 1 0.97 0.25 0.18 1

The indicated function y1(x) is a solution of the given differential equation. Use reduction of order or formula (5) in Section 4.2, as instructed, to find a second solution y2(x).

A population of fish is living in an environment with limited resources. This environment can only support the population if it contains no more than M fish (otherwise some fish would starve due to an inadequate supply of food, etc.). There is considerable evidence to support the theory that, for some fish species, there is a minimum population m such that the species will become extinct if the size of the population falls below m. Such a population can be modelled using a modified logistic equation: dP =(1-)(-) m dt M

1. (True/False) A Simple Linear Regression Equation is found y = 1.456 + 5.33x based on a sample of 36 observations, then there is sufficient evident to conclude that x is positively related to y. True False