400 500 600 700 800 900 1,000 1,100 Sample Midrange (thousands of dollars) 34 38 95 137 140 155 155 207 110 169 209 217 314 314 323 343 347 349 363 274 369 388 389 397 416 448 483 487 373 450 488 516 571 595 600 738 762 769 863 1,084
To estimate a population mean μ, the sample mean x¯ is often used as an estimator. However, a different estimator is called the sample midrange, given by the formula sample minimum + sample maximum/ 2.
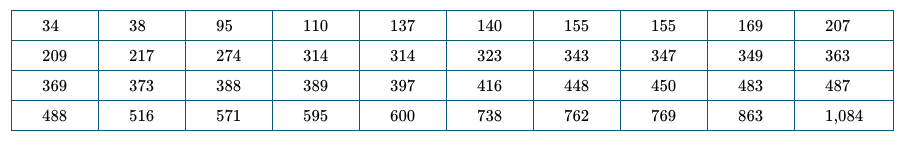
(a) The following table shows the values, in thousands of dollars, of 40 randomly selected houses in the city.
(i) Calculate the sample midrange for the data.
(ii) Explain why the sample midrange might be preferred to the sample mean as an estimator of the population mean.
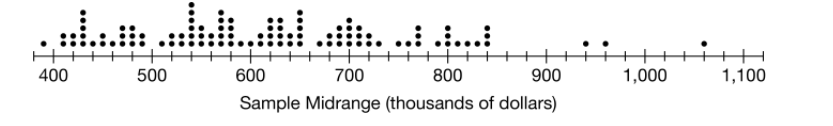
(b) To investigate the sampling distribution of the sample midrange, a simulation is performed in which 100 random samples of size n=40 were selected from the population of house values. For each sample, the sample midrange was calculated and recorded on the following dotplot. The mean of the distribution of sample midranges is $617,000 with standard deviation $136,000. Based on the results of the simulation, explain why the sample mean might be preferred to the sample midrange as an estimator of the population mean.
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images
