Elements Of Electromagnetics
7th Edition
ISBN: 9780190698614
Author: Sadiku, Matthew N. O.
Publisher: Oxford University Press
expand_more
expand_more
format_list_bulleted
Related questions
Question
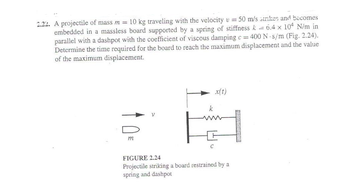
Transcribed Image Text:2.22. A projectile of mass m = 10 kg traveling with the velocity v =
=
.
50 m/s strikes and becomes
6.4 x 104 N/m in
embedded in a massless board supported by a spring of stiffness k
parallel with a dashpot with the coefficient of viscous damping c = 400 N s/m (Fig. 2.24).
Determine the time required for the board to reach the maximum displacement and the value
of the maximum displacement.
m
k
C
x(t)
FIGURE 2.24
Projectile striking a board restrained by a
spring and dashpot
PARA?
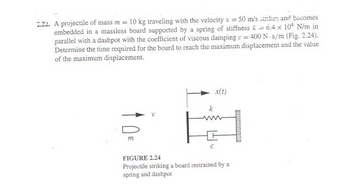
Transcribed Image Text:2.22. A projectile of mass m = 10 kg traveling with the velocity v =
=
.
50 m/s strikes and becomes
6.4 x 104 N/m in
embedded in a massless board supported by a spring of stiffness k
parallel with a dashpot with the coefficient of viscous damping c = 400 N s/m (Fig. 2.24).
Determine the time required for the board to reach the maximum displacement and the value
of the maximum displacement.
m
k
C
x(t)
FIGURE 2.24
Projectile striking a board restrained by a
spring and dashpot
PARA?
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A rigid block of mass M is mounted on four elastic supports, as shown in the figure below. A small mass m drops from a height h and adheres to the rigid block without rebounding, and the spring constant of each elastic support is k. Determine the equation of motion of the system after the small mass strikes the block. Given the following values, if the equation of motion is in the form + az = 0, determine the value of a. M = 55 kg; m = 6 kg; k=181 N/m; h= 20 cm a = Hence, determine the natural frequency of vibration of the system in units, Hz. f= The springs are modified, so that the equation of motion is now +14x=0. If x = 0 at the static equilibrium position when both masses are included, the initial position is given by mg 20. Hence, determine the initial velocity of the system at the point of impact, ż (t = 0) = V₁ (units m/s). Vo = If the resulting motion of the system after the impact is of the form r(t) = A cos wnt + B sin wnt, determine the values, A = B= wn (rad/s) = k k M m h…arrow_forward1. The mechanical system shown in Figure 1 is initially at rest. The displacement x of mass m is measured from the rest position. With m = 10 kg, b = 20 N-s/m, and k = 500 N/m, the system is set into motion at t=0 by an impulsive force whose strength is 20 N. Obtain the initial velocity *(0,) of the mass due to the impulsive force.arrow_forwardProblem 2. A projectile of mass m = 10 kg travelling with a velocity of 50m/s strikes and becomes embedded in a massless board supported by a spring of stiffness k-6.4x 104 N/m and a dashpot with damping coefficient, c. a) Determine the damping coefficient, c, for the fastest decay of the vibrations. b) For the value of c calculated above, determine the time required for the board to reach the maximum displacement. What is the value of the maximum displacement? (Hint: Velocity will be zero at the point of maximum displacement) c) [Bonus] What percentage of energy has been dissipated at the point of maximum displacement?arrow_forward
- PART OF MECHANICAL VIBRATIONS A viscously damped single degree of freedom mass–spring system has a massm of 2 kg, a spring coefficient k of 2000 N/m, and a damping constant c of5 N · s/m. Determine (a) the damping factor ξ , (b) the natural frequency ω,(c) the damped natural frequency ωd, and (d) the spring coefficient needed toobtain critically damped system.arrow_forwarddamping, c Sliding Crate with Bumper Stop A crate of mass m enters a lubricated slide at a loading dock with a velocity v, to the right and an initial position x(0) = x, in the diagram shown above. The sliding motion is lubricated, characterized by a linear damping coefficient C. At a distance x = L the crate contacts a bumper stop characterized by a spring constant k and a damping coefficient c. Numerical values for the system parameters are: C = 8 N-s/m k = 1000 N/m m = 50 kg X = 0 Vo = 5 m/s L= 20 m Ca is to be experimentally determined in completing this assignment Bumper: Assignment 1) Given these parameters, determine the governing dynamic equation for the crate position. 2) Convert the governing equation to state variable form. This requires two first-order equations: a. dv/dt f:(x,v) b. dx/dt = f.(x,v) where f, is a function of the states, x and v. where f, is a different function of the states, x and v. Make two sets of the above equations, one each depending on whether there…arrow_forward32 kg k- 12 kN/m Figure: Question 2 2. A 32-kg block is attached to a spring and can move without friction in a slot as shown. The block is in its equilibrium position when it is struck by a hammer, which imparts to the block an initial velocity of 250 mm/s. Determine a) the period and frequency of the resulting motion, b) the amplitude of the motion and the maximum acceleration of the block.arrow_forward
- An undamped free vibration system consisting of mass m = 5 kg and stiffnessa spring of 400 N/m, is given an initial displacement of 0.02 m and is kicked with an initial velocity of 0.1 m/s.Determine the equation of motion for the system.arrow_forwardQ.22 The natural frequencies corresponding to the spring-mass systems I and II are o, and O, respectively. The ratio is M k ww System I System II (a) 2 (b) (d) 4 1/4arrow_forwardA loaded railroad car weighing 30,000 lb is rolling at a constant velocity v0 when it couples with a spring and dashpot bumper system (Fig. 1). The recorded displacement–time curve of the loaded railroad car after coupling is as shown (Fig. 2). Determine (a) the damping constant, (b) the spring constant. (Hint: Use the definition of logarithmic decrement given in 19.129.)arrow_forward
- 3: A 24 lb weight stretches a spring 6 feet. The weight hangs vertically from the spring and a damping force numerically equal to 2√√3 times the instantaneous velocity acts on the system. The weight is released from 3 feet above the equilibrium position with a downward velocity of 14 ft/s. (a) Determine the time (in seconds) at which the mass passes through the equilibrium position. (b) Find the time (in seconds) at which the mass attains its extreme displacement from the equilibrium position.arrow_forward2. A railroad car of mass 2,000 kg traveling at a velocity v = 10 m/s is stopped at the end of the tracks by a spring- damper system. If the stiffness of the spring is k =180 N/mm and the damping constant is c = 20 N-s/mm, determine (a) the maximum displacement of the car after engaging the springs and damper and (b) the time taken to reach the maximum displacement. k/2 k/2 A. 1.0048 m, 0.0736 s C. 1.1048 m, 1.0736 s B. 1.0548 m, 0.5736 s D. 1.1548 m, 1.5736 sarrow_forwardFig. P4.6 4.22. Derive the differential equation of motion of the simple vehicle model shown in Fig. P4.7. The vehicle is assumed to travel over the rough surface with a constant vehicle speed v. Obtain also the steady state response of the vehicle. For this system, let m = 10kg, k = 4 x 103 N/m, c = 150N · s/m, i = 2 m, and the amplitude Yo = 0.1 m. Determine the maximum vertical displacement of the mass and the corresponding vehicle speed. Determine also the maximum dynamic force transmitted to the mass at the resonant speed. m 2πη y = Y, sin y Fig. P4.7arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education 
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY