Chapter 9.6, Problem 9P

An object is dropped from the top of a building. At first the object falls slowly. but as it continues to fall, it falls faster and faster. The speed at which the object falls increases at a steady rate. One function that arises from this scenatio is the height function, whose input is the time elapsed since the object was dropped and whose output is the height of the object above the ground at that time. Another function that arises from this scenario is the speed function, whose input is the time elapsed since the object was dropped and whose output is the speed at which the object is falling at that time. Identify graphs of these two functions from among the graphs shown in Figure 9.62 and explain your choices.

 

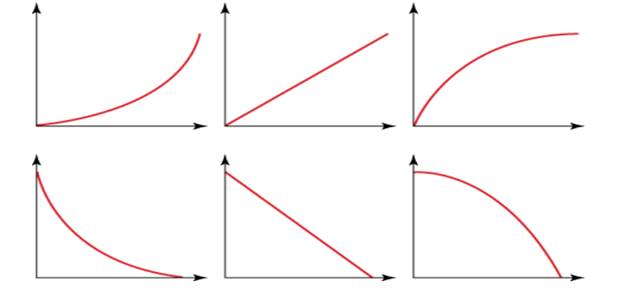
Figure 9.62 Which are graphs of the height and speed functions ?