Chapter 8, Problem 1P

A model rocket is fired horn the roof of a 50 ft tall building as shown in Fig. P8.1.

The height of the rocket is given by

$y\left(t\right)=y\left(0\right)+v\left(0\right)t-\frac{1}{2}g{t}^2\text{ft}$

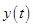
where $y\left(t\right)$ the height of the rocket at time t, $y\left(0\right)=H=50\text{ft}$ is the initial height of the rocket, $v\left(0\right)=150\text{ft/s}$ is the initial velocity of the rocket, and $g=32.2{\text{ft/s}}^2$ is the acceleration due to gravity and the following:

(a) Write the quadratic equation for the height $y\left(t\right)$ of the rocket.

(b) The velocity $v\left(t\right)=\frac{dy\left(t\right)}{dt}$.

(c) The acceleration $a\left(t\right)=\frac{dv\left(t\right)}{dt}=\frac{d^{2}y\left(t\right)}{d{t}^2}$.

(d) The time required to reach the maximum height as well as the corresponding height $y_{\max }$. Use your results to sketch $y\left(t\right)$.

To determine

(a)

The quadratic equation for the height 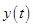of the rocket.

Answer to Problem 1P

The quadratic equation for the height 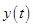is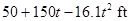

Explanation of Solution

Given:

The height of the rocket is

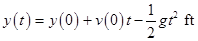  .......(1)

The initial height of the rocket 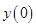is

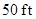
The initial velocity of the rocket 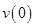is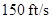

The value of acceleration due to gravity is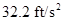

Calculation:

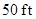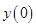
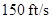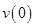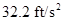Substitute for for and for 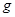in equation (1).

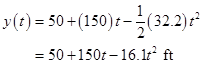
Conclusion:

Thus, the quadratic equation for the height 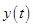is

To determine

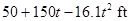
(b)

The velocity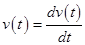

Answer to Problem 1P

The velocity 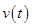is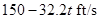

Explanation of Solution

Concept used:

Write the expression for the velocity

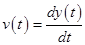  .......(2)

Here, 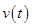is the velocity and 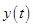is the height of the rocket at time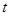

Calculation:

Substitute 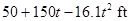for 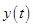in equation (2).

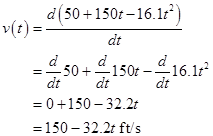
Conclusion:

Thus, the velocity 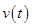is

To determine

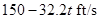
(c)

The acceleration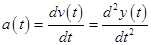

Answer to Problem 1P

The acceleration 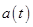is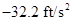

Explanation of Solution

Concept used:

Write the expression for the acceleration.

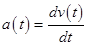  .......(3)

Here, the 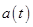is the acceleration and 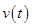is the velocity of the rocket at time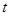

Calculation:

Substitute 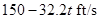for 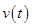in equation (3).

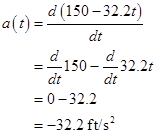
Conclusion:

Thus, the acceleration 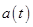is

To determine

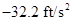
(d)

The time required to reach the maximum height, as well as, corresponding maximum height and sketch the result and use the result to sketch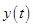

Answer to Problem 1P

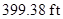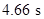The maximum height of the rocket is at and sketch for the height

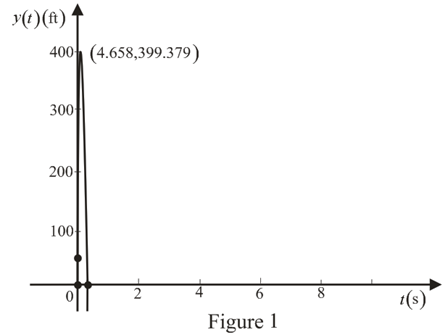
is drawn as shown in Figure 1.

Explanation of Solution

Concept used:

Write the expression for the maximum height.

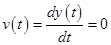  .......(4)

Calculation:

Equate the derivative of 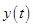to zero

Substitute 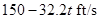for 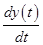in equation (4).

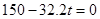
Rearrange for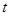

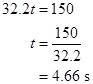
Therefore, the time at maximum height is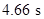

Write the expression for maximum height of the rocket.

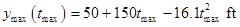  .......(5)

Substitute 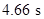for 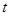in equation (5).

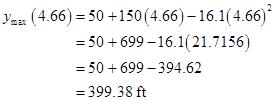
Therefore, the maximum height of the rocket reached is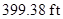

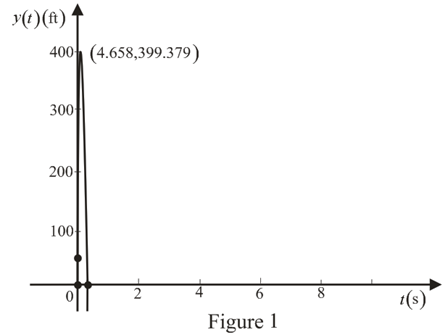
The sketch for the height is drawn as shown in Figure 1.

Conclusion:

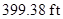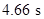Thus, the maximum height of the rocket is at and sketch for the height

is drawn as shown in Figure 1.