Videos
(a)
The sketch and label of a rectangular solid and the pyramid formed by the angular surface edges.
Answer to Problem 12A
The sketch and label of a rectangular solid and the pyramid formed by the angular surface edges is
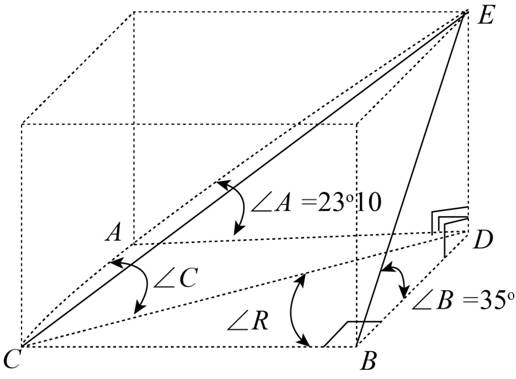
Explanation of Solution
Consider the angle ∠A=23°10′ of triangle ADE, the angle ∠B=35°00′ of triangle BDE.
There are mainly three types of views such as top view, front view and right side view. The rectangular solid and pyramid is formed using the trihedral method. In this method all the planes are perpendicular to each other and eight right triangles are formed. The object is placed in these right angles to take the projection.
The steps of the sketching of the rectangular solid and the pyramid formed by the surface to be cut and the extended sides of the block are following:
1.Draw a rectangular solid surface block.
2.Draw triangles on the extended surface of the rectangular solid surface block.
3.Cut the extended surface of the rectangular solid surface block as per the drawn triangle on the surface of the block to form a pyramid on the extended sides of the block.
4.The sketch of a pyramid formed on surface of the extended sides of the rectangular solid surface block.
5.Identify the angle ∠R and ∠C and the given angles in the sketch of a pyramid formed on surface of the extended sides of the rectangular solid surface block.
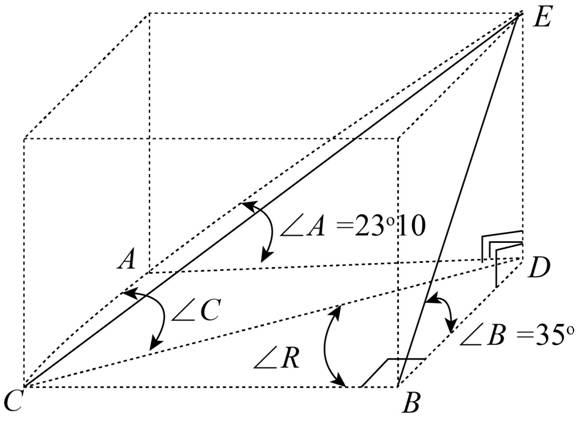
Figure-(1)
(b)
The angle ∠R.
Answer to Problem 12A
The angle ∠R is 31.37°.
Explanation of Solution
Write the expression for the angle ∠B using the triangle BDE.
tanB=DEBD ...... (I)
Here, the length of the side DE is DE and the length of the side BD is BD.
Write the expression for the angle ∠A using triangle ADE.
tanA=DEAD ...... (II)
Here, the length of the side AD is AD.
Write the expression for the angle ∠R using triangle CBD.
tanR=BDBC ...... (III)
Here, the length of the side AD is AD.
Calculation:
Consider the length of the side DE=1 unit.
Substitute 1 unit for DE and 35°00′ for B in Equation (I).
tan35°00′=1 unitBDBD=1 unittan35°00′BD=1 unit0.70020BD=1.4281 unit
Substitute 1 unit for DE and 23°10′ for A in Equation (II).
tan23°10′=1 unitADAD=1 unittan23°10′AD=1 unit0.4271AD=2.3413 unit
The length of the side AD and BC are same. Therefore, the length of the side BC is 2.3413 unit.
Substitute 2.3413 unit for BC and 1.4281 unit for BD in Equation (III).
tanR=1.4281 unit2.3413 unittanR=0.6099R=tan−1(0.6099)R=31.37°
Conclusion:
The angle ∠R is 31.37°.
(c)
The angle ∠C.
Answer to Problem 12A
The angle ∠C is 20.16°.
Explanation of Solution
Write the expression for the angle ∠R using the triangle CBD.
sinR=BDCD ...... (IV)
Write the expression for the angle ∠C using triangle CDE.
tanC=DECD ...... (V)
Calculation:
Consider the length of the side DE=1 unit.
Substitute 1.4281 unit for BD and 31.37° for R in Equation (IV).
sin31.37°=1.4182 unitCDCD=1.4182 unitsin31.37°CD=1.4182 unit0.5205CD=2.7246 unit
Substitute 2.7246 unit for CD and 1 unit for DE in Equation (V).
tanC=1 unit2.7246 unittanC=0.3672C=tan−1(0.3672)C=20.16°
Conclusion:
The angle ∠C is 20.16°.
Want to see more full solutions like this?
Chapter 76 Solutions
Mathematics for Machine Technology
- Let U = {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10} be the universal set. Let A = {0, 1, 2, 3, 9} and B = {2, 3, 4, 5, 6}. Select all elements in An B. 1 2 ✓ 3 + 5 10 7 > 00 ☐ 10arrow_forwardComplete the missing components of the know-show table to prove the statement be- low. Alternatively, you may construct your own table to prove the statement using the strategy that comes to your mind. Statement: For all integers n, if n is odd, then n³ + 4n+5 is even. Step Know P P1 n³ is odd P2 P3 5 is odd 0 Step Reason Hypothesis Product of even and odd is even 5 = 2(2)+1 Show Reasonarrow_forwardConsider the following false statement: For all integers a and b, if ab = 1 (mod 8), then a = 1 (mod 8) or b = 1 (mod 8). (a) Which of the following could be used as a counterexample. Select all that apply. a = -7 and b = −7 a = 1 and b = 23 ☐ a = 3 and b: = −5 ☐ a = 4 and b = 6 □ a = −1 and b = −9arrow_forward
- 1. Given X' = X 3 e2t (a) Verify that X₁(t) = (e) and X2(t) = (et) - are solutions to the given system. (b) Verify that X₁(t) and X2(t) form a fundamental set on the interval (-∞, ∞). (c) Write the general solution to the given system. (d) Find the solution that satisfies the initial condition X(0) = ( 2 ).arrow_forwardProve that a relation X defined on a set A that is reflexive, symmetric and antisymmetric is an equivalence relation and determine the equivalence classes.arrow_forwardLet X be the relation defined on the power set of the set integers P(Z) by AXB whenever A U B is a finite set of integers. Prove whether or not X is reflexive, symmetric, antisymmetirc or transitivearrow_forward
- Page < 1 of 2 - ZOOM + 1) Answer the following questions by circling TRUE or FALSE (No explanation or work required). −1 0 01 i) If A = 0 0 2 0, then its eigenvalues are ₁ = 1,λ₂ = 2, and 13 0 0 = : 0. (TRUE FALSE) ii) A linear transformation is operation preserving because the same result occurs whether you perform the operations of addition and scalar multiplication before or after applying the linear transformation. ( TRUE FALSE) iii) A linear transformation that is one-to-one and onto is called an isomorphism. (TRUE FALSE) iv) If the standard matrix A for the linear transformation T: R³ → R³ is -1 0 01 A = 2 00, then T is invertible. (TRUE FALSE) 0 1 1. v) Let A, B, and C be square matrices of order n. If A is similar to B and B is similar to C, then A is similar to C. ( TRUE FALSE) 2) a) i) Find the matrix that produces the counterclockwise rotation of 30° about the z-axis. ii) Find the image of the vector (1,1,1) for the rotation described in i). b) Give a geometric description…arrow_forwardPls help ASAParrow_forward1. Except for the door and floor, a shed is built entirely out of plywood. How many square meters of plywood are needed to build the shed? (1 foot 0.3048 m) 10 ft. 7 ft. 3 ft. 18 ft. 17 ft. 15 ft.arrow_forward
- 1. Leigh plans to invest $2500 for 4 years. She is considering two options: • A compound interest Guaranteed Investment Certificate (GIC) earning 5.6% per year for 4 years • A simple interest government bond earning 6.1% per year for 4 years a) Determine the total interest earned for each option at the end of each year. GIC Year Principal Interest earned (5.6%) Total interest 1 2 3 4 Government Bond [2 Marks] Interest earned (6.1%) Year Principal 1 2 3 4 b) Which is the better option? Explain. Total interest c) If Leigh can only invest for 3 years, which is the better option? Explain.arrow_forwardPls help ASAParrow_forwardPls help ASAParrow_forward
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Algebra for College StudentsAlgebraISBN:9781285195780Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning