Chapter 7.4, Problem 58PPS

(a).

To determine

Make tables for f(x) and g(x) using n = 3 and n = 4.

Explanation of Solution

Given:

  $\begin{array}{l}f(x)=x^n\\ g(x)=\sqrt[n]{x}\end{array}$

Calculation:

	n =3		n=4
x	$y=x^3$	$y=\sqrt[3]{x}$	$y=x^4$	$y=\sqrt[4]{x}$
-3	-27	-1.442	81	No real solution
-2	-8	-1.260	16	No real solution
-1	-1	-1	1	No real solution
0	0	0	0	0
1	1	1	1	1
2	8	1.260	16	1.189
3	27	1.442	81	1.316

Since the radicand of a radical with even index has to be non-negative.

  $y=\sqrt[4]{x}$ has index 4 , so it has no real solution if x is negative.

(b).

To determine

Graph the equations.

Explanation of Solution

Calculation:

From part (a),

	n =3		n=4
x	$y=x^3$	$y=\sqrt[3]{x}$	$y=x^4$	$y=\sqrt[4]{x}$
-3	-27	-1.442	81	No real solution
-2	-8	-1.260	16	No real solution
-1	-1	-1	1	No real solution
0	0	0	0	0
1	1	1	1	1
2	8	1.260	16	1.189
3	27	1.442	81	1.316

Plot the points on the coordinate plane and graph the equations:

  

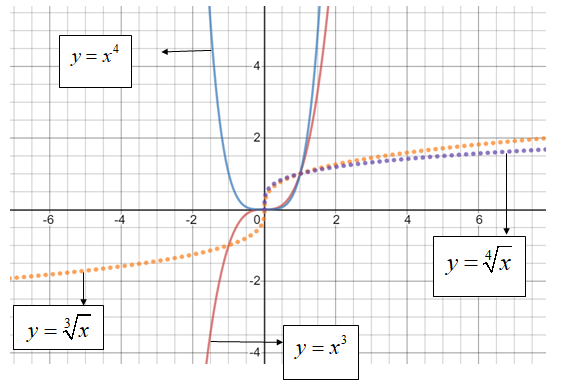
(c).

To determine

Find the equations that are functions and the functions that are one-to-one.

Answer to Problem 58PPS

All are functions and all except $f(x)=x^4$ are one-to-one.

Explanation of Solution

Given:

  $\begin{array}{l}f(x)=x^n\\ g(x)=\sqrt[n]{x}\end{array}$

Calculation:

From part (b) ,

Since the graph of all the equations pass the vertical line test , that is any vertical line intersects the graph at only one point. So, all the equations are functions.

Since the graph of the function $f(x)=x^4$ does not pass the horizontal line test , as the line $y=1$ intersects the graph of the function at 2 points. So, $f(x)=x^4$ is not one-to-one .All other functions pass the hortizontal line test since any horizontal line intersects the graphs of the functions at only one point. So, all the other functions are one-to-one .

(d).

To determine

Find the values of n for which f(x) and g(x) are inverses of each other.

Answer to Problem 58PPS

  $f(x)=x^3\text{ and }g(x)=\sqrt[3]{x}$ are inverse of each other.

Explanation of Solution

Given:

  $\begin{array}{l}f(x)=x^n\\ g(x)=\sqrt[n]{x}\end{array}$

Calculation:

From part (c) ,

Since $f(x)=x^4$ is not one-to-one , so it does not have inverse. As we know the rule that :

A function has an inverse if and only if it is one-to-one.

From the same rule, since $f(x)=x^3$ is one-to-one , so it has an inverse.

Also , we can observe from the graph :

If two functions are inverse of each other , their graph is symmetric along the line $y=x$ .

Since the graphs of the functions $f(x)=x^4\text{ and }g(x)=\sqrt[4]{x}$ are not symmetric along the line $y=x$ , since the range of $g(x)=\sqrt[4]{x}$ is $\left\{y\right|y\ge 0\}$ but domain of $f(x)=x^4$ is $\left\{x\right|x\in ℝ\}$ , which are not same. So, they are not inverses.

But , the graphs of the functions $f(x)=x^3\text{ and }g(x)=\sqrt[3]{x}$ are symmetric along the line $y=x$ , so they are inverse of each other.

(e).

To determine

Find the conclusions that you can make about $g(x)=\sqrt[n]{x}\text{ and }f(x)=x^n$ for all positive even values of n and for odd values of n.

Answer to Problem 58PPS

For positive even values of n , the pair of functions are not inverses.

For positive odd values of n, the pair of functions are inverses.

Explanation of Solution

Given:

  $\begin{array}{l}f(x)=x^n\\ g(x)=\sqrt[n]{x}\end{array}$

Calculation:

For positive even values of n , the pair of functions are not one-to −one . Hence are not inverses of each other. Here , the domain of $f(x)=x^{2m}$ is $\left\{x\right|x\in ℝ\}$ , while the range of $g(x)=\sqrt[2m]{x}$ is $\left\{y\right|y\ge 0\}$ , and they are not equal .

While for postitive odd values of n , the pair of functions are one-to-one .Hence they are inverses of each other.