College Algebra (Collegiate Math)
College Algebra (Collegiate Math)
2nd Edition
ISBN: 9780077836344
Author: Julie Miller, Donna Gerken
Publisher: McGraw-Hill Education
bartleby

Videos

Textbook Question
Book Icon
Chapter 7.2, Problem 66PE

The cross section of a cooling tower of a nuclear power plant is in the shape of a hyperbola, and can be modeled by the equation

x 2 625 − ( y − 80 ) 2 2500 = 1 where x and y are measured in meters. The top of the tower is 120 m above the base.

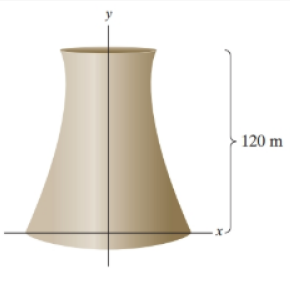
Chapter 7.2, Problem 66PE, 66. The cross section of a cooling tower of a nuclear power plant is in the shape of a hyperbola,

a. Determine the diameter of the tower at the base. Round to the nearest meter.

b. Determine the diameter of the tower at the top. Round to the nearest meter.

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 7.2, Problem 65PE
Next
Chapter 7.2, Problem 67PE
Students have asked these similar questions
I need a little help with this exercise. I'm confused about how to solve it. Thanks.

Chapter 7 Solutions

College Algebra (Collegiate Math)

Ch. 7.1 - Skill Practice 1. Graph the ellipse, and identify...Ch. 7.1 - Prob. 2SPCh. 7.1 - Prob. 3SPCh. 7.1 - Prob. 4SPCh. 7.1 - Prob. 5SPCh. 7.1 - Prob. 6SPCh. 7.1 - Prob. 7SPCh. 7.1 - 1. The circle, the ellipse, the hyperbola, and the...Ch. 7.1 - Prob. 2PECh. 7.1 - Prob. 3PE
Ch. 7.1 - Prob. 4PECh. 7.1 - Prob. 5PECh. 7.1 - Given x2a2+y2b2=1,whereab0, the ordered pairs...Ch. 7.1 - Prob. 7PECh. 7.1 - Prob. 8PECh. 7.1 - Prob. 9PECh. 7.1 - Prob. 10PECh. 7.1 - For Exercises 1112, from the equation of the...Ch. 7.1 - Prob. 12PECh. 7.1 - For Exercises 1322, a. Identify the center of the...Ch. 7.1 - Prob. 14PECh. 7.1 - For Exercises 13–22, a. Identify the center of...Ch. 7.1 - Prob. 16PECh. 7.1 - For Exercises 1322, a. Identify the center of the...Ch. 7.1 - For Exercises 1322, a. Identify the center of the...Ch. 7.1 - For Exercises 1322, a. Identify the center of the...Ch. 7.1 - For Exercises 13–22, a. Identify the center of...Ch. 7.1 - For Exercises 1322, a. Identify the center of the...Ch. 7.1 - For Exercises 13–22, a. Identify the center of...Ch. 7.1 - For Exercises 2332, a. Identify the center of the...Ch. 7.1 - Prob. 24PECh. 7.1 - For Exercises 2332, a. Identify the center of the...Ch. 7.1 - Prob. 26PECh. 7.1 - For Exercises 2332, a. Identify the center of the...Ch. 7.1 - Prob. 28PECh. 7.1 - For Exercises 2332, a. Identify the center of the...Ch. 7.1 - Prob. 30PECh. 7.1 - For Exercises 23–32, a. Identify the center of...Ch. 7.1 - Prob. 32PECh. 7.1 - For Exercises 33–42, a. Write the equation of the...Ch. 7.1 - For Exercises 33–42, a. Write the equation of the...Ch. 7.1 - For Exercises 3342, a. Write the equation of the...Ch. 7.1 - Prob. 36PECh. 7.1 - For Exercises 33–42, a. Write the equation of the...Ch. 7.1 - Prob. 38PECh. 7.1 - For Exercises 3342, a. Write the equation of the...Ch. 7.1 - Prob. 40PECh. 7.1 - For Exercises 3342, a. Write the equation of the...Ch. 7.1 - Prob. 42PECh. 7.1 - For Exercises 43–56, write the standard form of an...Ch. 7.1 - For Exercises 43–56, write the standard form of an...Ch. 7.1 - For Exercises 4356, write the standard form of an...Ch. 7.1 - Prob. 46PECh. 7.1 - For Exercises 4356, write the standard form of an...Ch. 7.1 - For Exercises 4356, write the standard form of an...Ch. 7.1 - For Exercises 4356, write the standard form of an...Ch. 7.1 - Prob. 50PECh. 7.1 - For Exercises 4356, write the standard form of an...Ch. 7.1 - Prob. 52PECh. 7.1 - For Exercises 43–56, write the standard form of an...Ch. 7.1 - For Exercises 4356, write the standard form of an...Ch. 7.1 - For Exercises 4356, write the standard form of an...Ch. 7.1 - Prob. 56PECh. 7.1 - Prob. 57PECh. 7.1 - 58. The reflective property of an ellipse is the...Ch. 7.1 - 59. A homeowner wants to make an elliptical rug...Ch. 7.1 - Coordinate axes are superimposed on a photo of an...Ch. 7.1 - Prob. 61PECh. 7.1 - A window above a door is to be made in the shape...Ch. 7.1 - Prob. 63PECh. 7.1 - Prob. 64PECh. 7.1 - Prob. 65PECh. 7.1 - Prob. 66PECh. 7.1 - Prob. 67PECh. 7.1 - Prob. 68PECh. 7.1 - Prob. 69PECh. 7.1 - Prob. 70PECh. 7.1 - Prob. 71PECh. 7.1 - Prob. 72PECh. 7.1 - Prob. 73PECh. 7.1 - 74. Halley’s Comet and the comet Hale-Bopp both...Ch. 7.1 - Prob. 75PECh. 7.1 - The planet Saturn orbits the Sun in an elliptical...Ch. 7.1 - Prob. 77PECh. 7.1 - 78. The Ellipse, also called President’s Park...Ch. 7.1 - For Exercises 7982, write the standard form of an...Ch. 7.1 - Prob. 80PECh. 7.1 - Prob. 81PECh. 7.1 - Prob. 82PECh. 7.1 - A circular vent pipe is placed on a plat roof. a....Ch. 7.1 - A cylindrical glass of water with diameter 3.5 in....Ch. 7.1 - Prob. 85PECh. 7.1 - Prob. 86PECh. 7.1 - Prob. 87PECh. 7.1 - Prob. 88PECh. 7.1 - Prob. 89PECh. 7.1 - Prob. 90PECh. 7.1 - Prob. 91PECh. 7.1 - Prob. 92PECh. 7.1 - Prob. 93PECh. 7.1 - Prob. 94PECh. 7.1 - 95. An elliptical pool table is in the shape of an...Ch. 7.1 - Prob. 96PECh. 7.1 - Prob. 97PECh. 7.1 - Prob. 98PECh. 7.1 - Prob. 99PECh. 7.1 - 100. Find the pints on the ellipse that are twice...Ch. 7.1 - Prob. 101PECh. 7.1 - Prob. 102PECh. 7.1 - Prob. 103PECh. 7.1 - Prob. 104PECh. 7.1 - Prob. 105PECh. 7.2 - Skill Practice Repeat Example 1 with the equation:...Ch. 7.2 - Prob. 2SPCh. 7.2 - Prob. 3SPCh. 7.2 - Prob. 4SPCh. 7.2 - Prob. 5SPCh. 7.2 - Prob. 6SPCh. 7.2 - Prob. 7SPCh. 7.2 - Prob. 1PECh. 7.2 - Prob. 2PECh. 7.2 - Prob. 3PECh. 7.2 - Prob. 4PECh. 7.2 - Prob. 5PECh. 7.2 - Prob. 6PECh. 7.2 - Prob. 7PECh. 7.2 - For Exercises 7–8, a. Use the distance formula to...Ch. 7.2 - For Exercises 9–12, determine whether the...Ch. 7.2 - Prob. 10PECh. 7.2 - For Exercises 912, determine whether the...Ch. 7.2 - Prob. 12PECh. 7.2 - For Exercises 13–22, a. Identify the center....Ch. 7.2 - Prob. 14PECh. 7.2 - For Exercises 13–22, a. Identify the center....Ch. 7.2 - Prob. 16PECh. 7.2 - For Exercises 1322, a. Identify the center. b....Ch. 7.2 - Prob. 18PECh. 7.2 - For Exercises 13–22, a. Identify the center....Ch. 7.2 - Prob. 20PECh. 7.2 - For Exercises 13–22, a. Identify the center....Ch. 7.2 - Prob. 22PECh. 7.2 - For Exercises 2332, a. Identify the center. b....Ch. 7.2 - Prob. 24PECh. 7.2 - For Exercises 2332, a. Identify the center. b....Ch. 7.2 - Prob. 26PECh. 7.2 - For Exercises 23–32, a. Identify the center....Ch. 7.2 - Prob. 28PECh. 7.2 - For Exercises 2332, a. Identify the center. b....Ch. 7.2 - Prob. 30PECh. 7.2 - For Exercises 23–32, a. Identify the center....Ch. 7.2 - Prob. 32PECh. 7.2 - For Exercises 3340, a. Write the equation of the...Ch. 7.2 - Prob. 34PECh. 7.2 - For Exercises 3340, a. Write the equation of the...Ch. 7.2 - For Exercises 33–40, a. Write the equation of...Ch. 7.2 - For Exercises 33–40, a. Write the equation of...Ch. 7.2 - Prob. 38PECh. 7.2 - For Exercises 33–40, a. Write the equation of...Ch. 7.2 - Prob. 40PECh. 7.2 - For Exercises 41–50, write the standard form of...Ch. 7.2 - Prob. 42PECh. 7.2 - For Exercises 4150, write the standard form of the...Ch. 7.2 - Prob. 44PECh. 7.2 - For Exercises 4150, write the standard form of the...Ch. 7.2 - Prob. 46PECh. 7.2 - For Exercises 41–50, write the standard form of...Ch. 7.2 - For Exercises 4150, write the standard form of the...Ch. 7.2 - For Exercises 41–50, write the standard form of...Ch. 7.2 - For Exercises 41–50, write the standard form of...Ch. 7.2 - Prob. 51PECh. 7.2 - Prob. 52PECh. 7.2 - Prob. 53PECh. 7.2 - Prob. 54PECh. 7.2 - Prob. 55PECh. 7.2 - Determine the eccentricity of a hyperbola with a...Ch. 7.2 - Prob. 57PECh. 7.2 - Prob. 58PECh. 7.2 - Two radio transmitters are 1000 mi apart at points...Ch. 7.2 - Prob. 60PECh. 7.2 - 61. In some designs of eyeglasses, the surface is...Ch. 7.2 - Prob. 62PECh. 7.2 - Prob. 63PECh. 7.2 - Prob. 64PECh. 7.2 - 65. In September 2009, Australian astronomer...Ch. 7.2 - 66. The cross section of a cooling tower of a...Ch. 7.2 - Prob. 67PECh. 7.2 - Prob. 68PECh. 7.2 - Prob. 69PECh. 7.2 - Prob. 70PECh. 7.2 - Prob. 71PECh. 7.2 - Prob. 72PECh. 7.2 - Prob. 73PECh. 7.2 - Prob. 74PECh. 7.2 - Prob. 75PECh. 7.2 - Prob. 76PECh. 7.2 - Prob. 77PECh. 7.2 - Prob. 78PECh. 7.2 - Prob. 79PECh. 7.2 - Prob. 80PECh. 7.2 - Prob. 81PECh. 7.2 - Prob. 82PECh. 7.2 - A hyperboloid of one sheet is a three-dimensional...Ch. 7.2 - a. Radio signals emitted from points (8,0)and(8,0)...Ch. 7.2 - Prob. 85PECh. 7.2 - Prob. 86PECh. 7.2 - Prob. 87PECh. 7.2 - Prob. 88PECh. 7.3 - Skill Practice Given a parabola defined by x2=4y,...Ch. 7.3 - Prob. 2SPCh. 7.3 - Prob. 3SPCh. 7.3 - Prob. 4SPCh. 7.3 - Prob. 5SPCh. 7.3 - Skill Practice Determine the standard form of an...Ch. 7.3 - Prob. 7SPCh. 7.3 - Prob. 1PECh. 7.3 - Prob. 2PECh. 7.3 - Prob. 3PECh. 7.3 - Prob. 4PECh. 7.3 - Prob. 5PECh. 7.3 - Prob. 6PECh. 7.3 - Prob. 7PECh. 7.3 - Prob. 8PECh. 7.3 - Prob. 9PECh. 7.3 - Prob. 10PECh. 7.3 - Prob. 11PECh. 7.3 - Prob. 12PECh. 7.3 - Prob. 13PECh. 7.3 - Prob. 14PECh. 7.3 - For Exercises 15–22, a model of the form is...Ch. 7.3 - Prob. 16PECh. 7.3 - For Exercises 1522, a model of the form...Ch. 7.3 - For Exercises 15–22, a model of the form is...Ch. 7.3 - For Exercises 15–22, a model of the form is...Ch. 7.3 - Prob. 20PECh. 7.3 - For Exercises 1522, a model of the form...Ch. 7.3 - Prob. 22PECh. 7.3 - A 20-in. satellite dish for a television has...Ch. 7.3 - 24. Solar cookers provide an alternative form of...Ch. 7.3 - If a cross section of the parabolic mirror in a...Ch. 7.3 - Prob. 26PECh. 7.3 - For Exercises 2734, an equation of a parabola...Ch. 7.3 - Prob. 28PECh. 7.3 - For Exercises 2734, an equation of a parabola...Ch. 7.3 - Prob. 30PECh. 7.3 - For Exercises 2734, an equation of a parabola...Ch. 7.3 - Prob. 32PECh. 7.3 - For Exercises 2734, an equation of a parabola...Ch. 7.3 - Prob. 34PECh. 7.3 - For Exercises 35–44, an equation of a parabola or...Ch. 7.3 - Prob. 36PECh. 7.3 - For Exercises 35–44, an equation of a parabola or...Ch. 7.3 - Prob. 38PECh. 7.3 - For Exercises 35–44, an equation of a parabola or...Ch. 7.3 - Prob. 40PECh. 7.3 - For Exercises 3544, an equation of a parabola...Ch. 7.3 - Prob. 42PECh. 7.3 - For Exercises 3544, an equation of a parabola...Ch. 7.3 - Prob. 44PECh. 7.3 - For Exercises 45–52, an equation of a parabola is...Ch. 7.3 - For Exercises 4552, an equation of a parabola is...Ch. 7.3 - For Exercises 45–52, an equation of a parabola is...Ch. 7.3 - For Exercises 45–52, an equation of a parabola is...Ch. 7.3 - For Exercises 45–52, an equation of a parabola is...Ch. 7.3 - For Exercises 45–52, an equation of a parabola is...Ch. 7.3 - For Exercises 45–52, an equation of a parabola is...Ch. 7.3 - Prob. 52PECh. 7.3 - For Exercises 53–58, fill in the blanks, Let ...Ch. 7.3 - Prob. 54PECh. 7.3 - For Exercises 5358, fill in the blanks, Let |p|...Ch. 7.3 - Prob. 56PECh. 7.3 - For Exercises 53–58, fill in the blanks, Let ...Ch. 7.3 - Prob. 58PECh. 7.3 - 59. If the focal length of a parabola is 6 units...Ch. 7.3 - If the axis of symmetry of a parabola is given by...Ch. 7.3 - For Exercises 6168, determine the standard form of...Ch. 7.3 - Prob. 62PECh. 7.3 - For Exercises 6168, determine the standard form of...Ch. 7.3 - Prob. 64PECh. 7.3 - For Exercises 6168, determine the standard form of...Ch. 7.3 - Prob. 66PECh. 7.3 - For Exercises 6168, determine the standard form of...Ch. 7.3 - Prob. 68PECh. 7.3 - Prob. 69PECh. 7.3 - 70. A solar water heater is made from a long sheet...Ch. 7.3 - The Hubble Space Telescope was launched into space...Ch. 7.3 - 72. The James Webb Space Telescope (JWST) is a now...Ch. 7.3 - Prob. 73PECh. 7.3 - Prob. 74PECh. 7.3 - Prob. 75PECh. 7.3 - Prob. 76PECh. 7.3 - Prob. 77PECh. 7.3 - Prob. 78PECh. 7.3 - Prob. 79PECh. 7.3 - Prob. 80PECh. 7.3 - Prob. 81PECh. 7.3 - Prob. 82PECh. 7.3 - Prob. 83PECh. 7.3 - Prob. 84PECh. 7.3 - Prob. 85PECh. 7.3 - Prob. 86PECh. 7.3 - Prob. 1PRECh. 7.3 - Prob. 2PRECh. 7.3 - Prob. 3PRECh. 7.3 - Prob. 4PRECh. 7.3 - Prob. 5PRECh. 7.3 - Prob. 6PRECh. 7.3 - Prob. 7PRECh. 7.3 - Prob. 8PRECh. 7.3 - For Exercises 916, determine whether the equation...Ch. 7.3 - Prob. 10PRECh. 7.3 - Prob. 11PRECh. 7.3 - Prob. 12PRECh. 7.3 - Prob. 13PRECh. 7.3 - Prob. 14PRECh. 7.3 - Prob. 15PRECh. 7.3 - Prob. 16PRECh. 7.3 - Prob. 17PRECh. 7.3 - Prob. 18PRECh. 7.3 - Prob. 19PRECh. 7.3 - For Exercises 17–22, an equation of a degenerate...Ch. 7.3 - Prob. 21PRECh. 7.3 - Prob. 22PRECh. 7.3 - Prob. 23PRECh. 7.3 - Prob. 24PRECh. 7.3 - Prob. 25PRECh. 7 - 1. How are the graphs of these ellipses similar...Ch. 7 - Prob. 2RECh. 7 - Prob. 3RECh. 7 - Prob. 4RECh. 7 - Prob. 5RECh. 7 - For Exercises 67, a. Write the equation of the...Ch. 7 - Prob. 7RECh. 7 - Prob. 8RECh. 7 - Prob. 9RECh. 7 - Prob. 10RECh. 7 - Prob. 11RECh. 7 - Prob. 12RECh. 7 - Prob. 13RECh. 7 - Prob. 14RECh. 7 - Prob. 15RECh. 7 - Prob. 16RECh. 7 - Prob. 17RECh. 7 - Prob. 18RECh. 7 - Prob. 19RECh. 7 - For Exercises 1720, an equation of a hyperbola is...Ch. 7 - Prob. 21RECh. 7 - Prob. 22RECh. 7 - Prob. 23RECh. 7 - Prob. 24RECh. 7 - Prob. 25RECh. 7 - Prob. 26RECh. 7 - Prob. 27RECh. 7 - Prob. 28RECh. 7 - Prob. 29RECh. 7 - Prob. 30RECh. 7 - Prob. 31RECh. 7 - For Exercises 3033, an equation of a parabola is...Ch. 7 - Prob. 33RECh. 7 - Prob. 34RECh. 7 - Prob. 35RECh. 7 - Prob. 36RECh. 7 - Prob. 37RECh. 7 - Prob. 38RECh. 7 - Prob. 39RECh. 7 - Prob. 40RECh. 7 - Prob. 41RECh. 7 - Prob. 42RECh. 7 - 43. Two large airship hangars were built in Orly,...Ch. 7 - Prob. 1TCh. 7 - Prob. 2TCh. 7 - Prob. 3TCh. 7 - Prob. 4TCh. 7 - Prob. 5TCh. 7 - Prob. 6TCh. 7 - Prob. 7TCh. 7 - Prob. 8TCh. 7 - Prob. 9TCh. 7 - For Exercises 916, a. Identify the equation as...Ch. 7 - Prob. 11TCh. 7 - Prob. 12TCh. 7 - For Exercises 916, a. Identify the equation as...Ch. 7 - Prob. 14TCh. 7 - Prob. 15TCh. 7 - For Exercises 916, a. Identify the equation as...Ch. 7 - 17. The entrance to a tunnel is in the shape of a...Ch. 7 - 18. Neptune orbits the Sun in an elliptical path...Ch. 7 - Prob. 19TCh. 7 - Prob. 20TCh. 7 - The middle span of the Golden Gate Bridge in San...Ch. 7 - Prob. 22TCh. 7 - Prob. 23TCh. 7 - Prob. 24TCh. 7 - Write the standard form of an equation of a...Ch. 7 - Prob. 26TCh. 7 - Prob. 27TCh. 7 - Prob. 28TCh. 7 - Prob. 29TCh. 7 - Prob. 30TCh. 7 - Prob. 31TCh. 7 - 1. Use long division to divide. Ch. 7 - Prob. 2CRECh. 7 - Prob. 3CRECh. 7 - Prob. 4CRECh. 7 - Prob. 5CRECh. 7 - Prob. 6CRECh. 7 - Prob. 7CRECh. 7 - Prob. 8CRECh. 7 - Prob. 9CRECh. 7 - Prob. 10CRECh. 7 - Prob. 11CRECh. 7 - Prob. 12CRECh. 7 - Prob. 13CRECh. 7 - Prob. 14CRECh. 7 - Prob. 15CRECh. 7 - Prob. 16CRECh. 7 - Prob. 17CRECh. 7 - Prob. 18CRECh. 7 - Prob. 19CRECh. 7 - Prob. 20CRECh. 7 - Prob. 21CRECh. 7 - Prob. 22CRECh. 7 - Prob. 23CRECh. 7 - Prob. 24CRECh. 7 - Prob. 25CRECh. 7 - Prob. 26CRECh. 7 - Prob. 27CRECh. 7 - Prob. 28CRECh. 7 - Prob. 29CRECh. 7 - Prob. 30CRE
Knowledge Booster
Background pattern image
Algebra
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.
Similar questions
Recommended textbooks for you
Text book image
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Text book image
Intermediate Algebra
Algebra
ISBN:9780998625720
Author:Lynn Marecek
Publisher:OpenStax College
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
PREALGEBRA
Algebra
ISBN:9781938168994
Author:OpenStax
Publisher:OpenStax
Text book image
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Text book image
College Algebra
Algebra
ISBN:9781337282291
Author:Ron Larson
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
08 - Conic Sections - Hyperbolas, Part 1 (Graphing, Asymptotes, Hyperbola Equation, Focus); Author: Math and Science;https://www.youtube.com/watch?v=Ryj0DcdGPXo;License: Standard YouTube License, CC-BY