Mathematics for Machine Technology
7th Edition
ISBN: 9781133281450
Author: John C. Peterson, Robert D. Smith
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 51, Problem 30A
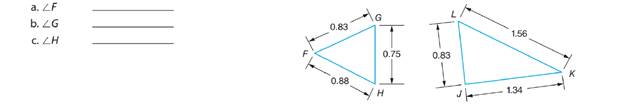
Determine the answers to the following exercises which are based on corresponding parts.
Identify the angle that corresponds with each angle listed. All dimensions are in inches.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
For each of the following functions determine whether it is the real part of a
differentiable function defined on C. If it is, determine a corresponding imaginary
part.
(i) u(x, y) = x² - y²+4xy;
(ii) u(x, y) = x³ — y³ + 3x²y.
-
Let A be an algebra and X C A a subset. Recall that the centraliser of X in
A is defined to be
==
C(X) = {a A | ax = xa for all x = X}.
Let
U =
{ (o b) : a, b, c & R} ≤ M₂(R).
C
as in Question 1.
(i) Show that the centraliser of U in M₂(R) is:
C(U) = {(o 9 ) ; a € R} .
0
:
(ii) Let M=R2, the natural module for U. Show that Endu (M) = R.
(iii) Find all one-dimensional submodules of M.
(iv) Is MXY for some submodules X, Y of M? (no proof is required for
this part.)
Let A be a finite-dimensional algebra. Prove that A is a semisimple algebra if
and only if every left ideal I of A admits a complement (so there exists a left
ideal J such that A = I J as vector spaces).
You I may assume that if a module M can be written as a sum of simple modules
M =
S₁+ S₂++ Sn, then we can find a subset R C {1, 2, ………, n} such that
DER ST is a direct sum of simples. You may also assume that every
non-zero finite-dimensional module has a simple submodule.
M
=
Chapter 51 Solutions
Mathematics for Machine Technology
Ch. 51 - Determine the values of 2, 3, and 4 if l is 113.Ch. 51 - Use a protractor to measure the angle to the...Ch. 51 - Express 191.5326 as degrees, minutes, and seconds....Ch. 51 - Cast iron 10 cm in diameter is turned in a lathe...Ch. 51 - Solve 4t7t2216=12t.Ch. 51 - If m m=5,p=2,and r=12 ,find m24p+3rmp+prmmr.Give...Ch. 51 - Identify each of the triangles 7 through 14 as...Ch. 51 - Identify each of the triangles 7 through 14 as...Ch. 51 - Identify each of the triangles 7 through 14 as...Ch. 51 - Identify each of the triangles 7 through 14 as...
Ch. 51 - Identify each of the triangles 7 through 14 as...Ch. 51 - Identify each of the triangles 7 through 14 as...Ch. 51 - Identify each of the triangles 7 through 14 as...Ch. 51 - Identify each of the triangles 7 through 14 as...Ch. 51 - Solve the following exercises: Find the value of...Ch. 51 - Solve the following exercises: Find the value of...Ch. 51 - Solve the following exercises: Find the value of...Ch. 51 - Solve the following exercises: Find the value of...Ch. 51 - Solve the following exercises: In triangle ABC, BC...Ch. 51 - Solve the following exercises: In triangle EFG,...Ch. 51 - Solve the following exercises: Find the value of...Ch. 51 - Solve the following exercises: All dimensions are...Ch. 51 - Solve the following exercises: Find the value of...Ch. 51 - Solve the following exercises: Hole centrelines...Ch. 51 - Solve the following exercises: Find the value of...Ch. 51 - Solve the following exercises: ABDE,BC is an...Ch. 51 - Determine the answers to the following exercises...Ch. 51 - Determine the answers to the following exercises...Ch. 51 - Determine the answers to the following exercises...Ch. 51 - Determine the answers to the following exercises...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- Show that these two matrices generate the algebra M₂(Q) over the field Q. 0 1 S := and T = == -1 - -1 (13)arrow_forwardLet G = S3, the symmetric group on 3 letters. Show that K(X,Y) KG - (X21, YX XY², Y³ —– 1) ' - (Hint: Write S3 (12) and Y X S3 as a group.) = {id, (12), (23), (13), (123), (132)}. Consider the map from → (123). You may assume that these two elements generatearrow_forwardConsider a simplified two-joint robotic arm where the two joints are connected by rigid links.Each joint has a mass, and the links are modeled as idealized rods. The motion of each jointaffects the other through the coupling of forces. The system can be modeled by the followingsecond-order coupled ODEs: m1θ1" + k1(θ1 − θrest) + k3(θ1 − θ2) = 0 (equation 1)m2θ2" + k2(θ2 − θrest) + k3(θ2 − θ1) = 0 (equation 2) where θ1(t) and θ2(t) represent the angular positions of the first and second joints, respec-tively. m1 and m2 are the effective masses at each joint. k1, k2 and k3 are the stiffnessconstants representing the elastic restoring forces in the joints and links. θrest = 0 representsthe equilibrium position for each joint.The parameters are:m1 = 1, m2 = 1, θrest = 0, k1 = 1, k2 = 2, k3 = 3, θrest = 0The initial conditions are:θ1(0) = 1, θ2(0) = 2Please solve by hand to get θ1(t) and θ2(t) using eigenvectors and eigenvalues. Show all steps/calculations, and provide a written description…arrow_forward
- 3feet in 1secound; 21feet in x secondsarrow_forward(15 pts) Show your work to get full credit! Compute a QR factorization of the matrixarrow_forward(5) Let G be a group. Show that G acts on itself via the action g⚫a (i.e. using the binary operation of G) for all g, a Є G. [ for each of the three axioms, plus 1 for conclusion] = gaarrow_forward
- = (2) Let H {id, (12) (34), (13)(24), (14)(23)}. Prove that H is a sub- group of the symmetric group S4 of degree 4. each of the four axioms, plus 1 for the conclusion] 1 forarrow_forward(15 pts) Show your work to get full credit! Compute a singular value decomposition of the matrix A-( 7²)arrow_forwardSudoku Puzzle Rules Solving a Sudoku puzzle does not require the knowledge of mathematics but it does require logical thinking. A Sudoku puzzle is a grid of nine by nine squares or cells that have been subdivided into nine "regions" of three by three cells. Consider the following diagram: The objective of Sudoku is to enter a digit from 1 through 9 in each cell, in such a way that: Each horizontal row (shown in blue) contains each digit exactly once. Each vertical column (shown in pink) contains each digit exactly once. Each sub grid or region (shown in orange) contains each digit exactly once. In each Sudoku puzzle, some digits have already been entered, you may not change these. Your job is to fill the remaining cells with digits keeping in mind the three rules discussed above. Sudoku puzzles have become so popular that they can be found in newspapers and magazines around the world. If you are ever planning a long trip where you may be stuck in a car, Sudoku can help to pass the…arrow_forward
- (3) Let G be a group and let gЄ G. Prove that the function f : G→ G given by f(x) = gx is bijective (i.e. injective, and surjective). [ 2 for injectivity, 2 for surjectivity, 1 for bijectivity]arrow_forward(4) Let X = {(a,b) : a,bЄ Z, a on X given by (a, b) R(c, d) if ad 0,60}. Show that the relation R = bc is an equivalence relation. [ 1 for reflexivity, 1 for symmetry, 1 for transitivity, 1 for conclusion]arrow_forward(15 pts) Show your work to get full credit! Compute a diagonalization of the matrix -1 0 0 A = 0 1 1 0 20arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning
01 - Angles and Angle Measure in Degrees - Part 1 - Types of Angles & What is an Angle?; Author: Math and Science;https://www.youtube.com/watch?v=hy95VyPet-M;License: Standard YouTube License, CC-BY