Algebra 1
Algebra 1
1st Edition
ISBN: 9780078884801
Author: McGraw-Hill/Glencoe
Publisher: Glencoe/McGraw-Hill School Pub Co
bartleby

Videos

Question
Book Icon
Chapter 5.1, Problem 15PPS
To determine

Solve for the unknown variable from the inequality equation.

Expert Solution & Answer
Check Mark

Answer to Problem 15PPS

  t>−5

Explanation of Solution

Given:

The inequality equation: t−3>−8

Concept Used:

In mathematics, an inequality is a relation which makes a non-equal comparison between two numbers or other mathematical expressions. It is used most often to compare two numbers on the number line by their size.

An inequality compares two values, showing if one is less than, greater than, or simply not equal to another value. a ≠ b says that a is not equal to b. a < b says that a is less than b. a > b says that a is greater than b.

There are four different types of inequalities:

Greater than − (>) ; Less than − (<) ; Greater than or equal to − (≥) ; Less than or equal to − (≤)

For Inequality equation: If b>c  ⇒  c  <  b  or  b  <  c  ⇒  c  >  b

Rules for solving inequality equations:

These things do not affect the direction of the inequality:

  • Add (or subtract) a number from both sides
  • Multiply (or divide) both sides by a positive number
  • Simplify a side

But these things do change the direction of the inequality (" < " becomes " > " for example):

  • Multiply (or divide) both sides by a negative number
  • Swapping left and right hand sides

Calculation:

The inequality equation: t−3>−8

Solve:

  t−3>−8t−3+3>−8+3  [ Add  3  to  both  sides ] t>−5

An open circle is used for greater than (>) or less than (<). The point is not part of the solution. The graph then extends endlessly in one direction.

Solution set on the number line

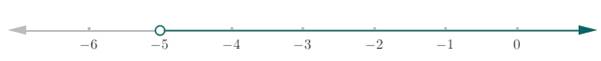
  Algebra 1, Chapter 5.1, Problem 15PPS

Solution of the inequality equation is t>−5

Solution set: (  −5  ,  ∞  )

Thus, the solution of the inequality equation t−3>−8 is t>−5

Previous
Chapter 5.1, Problem 14PPS
Next
Chapter 5.1, Problem 16PPS

Chapter 5 Solutions

Algebra 1

Ch. 5.1 - Prob. 1ACYPCh. 5.1 - Prob. 1BCYPCh. 5.1 - Prob. 2CYPCh. 5.1 - Prob. 3ACYPCh. 5.1 - Prob. 3BCYPCh. 5.1 - Prob. 4CYPCh. 5.1 - Prob. 1CYUCh. 5.1 - Prob. 2CYUCh. 5.1 - Prob. 3CYUCh. 5.1 - Prob. 4CYU
Ch. 5.1 - Prob. 5CYUCh. 5.1 - Prob. 6CYUCh. 5.1 - Prob. 7CYUCh. 5.1 - Prob. 8CYUCh. 5.1 - Prob. 9CYUCh. 5.1 - Prob. 10CYUCh. 5.1 - Prob. 11CYUCh. 5.1 - Prob. 12PPSCh. 5.1 - Prob. 13PPSCh. 5.1 - Prob. 14PPSCh. 5.1 - Prob. 15PPSCh. 5.1 - Prob. 16PPSCh. 5.1 - Prob. 17PPSCh. 5.1 - Prob. 18PPSCh. 5.1 - Prob. 19PPSCh. 5.1 - Prob. 20PPSCh. 5.1 - Prob. 21PPSCh. 5.1 - Prob. 22PPSCh. 5.1 - Prob. 23PPSCh. 5.1 - Prob. 24PPSCh. 5.1 - Prob. 25PPSCh. 5.1 - Prob. 26PPSCh. 5.1 - Prob. 27PPSCh. 5.1 - Prob. 28PPSCh. 5.1 - Prob. 29PPSCh. 5.1 - Prob. 30PPSCh. 5.1 - Prob. 31PPSCh. 5.1 - Prob. 32PPSCh. 5.1 - Prob. 33PPSCh. 5.1 - Prob. 34PPSCh. 5.1 - Prob. 35PPSCh. 5.1 - Prob. 36PPSCh. 5.1 - Prob. 37PPSCh. 5.1 - Prob. 38PPSCh. 5.1 - Prob. 39PPSCh. 5.1 - Prob. 40PPSCh. 5.1 - Prob. 41PPSCh. 5.1 - Prob. 42PPSCh. 5.1 - Prob. 43PPSCh. 5.1 - Prob. 44PPSCh. 5.1 - Prob. 45PPSCh. 5.1 - Prob. 46PPSCh. 5.1 - Prob. 47PPSCh. 5.1 - Prob. 48PPSCh. 5.1 - Prob. 49PPSCh. 5.1 - Prob. 50PPSCh. 5.1 - Prob. 51PPSCh. 5.1 - Prob. 52HPCh. 5.1 - Prob. 53HPCh. 5.1 - Prob. 54HPCh. 5.1 - Prob. 55HPCh. 5.1 - Prob. 56HPCh. 5.1 - Prob. 57STPCh. 5.1 - Prob. 58STPCh. 5.1 - Prob. 59STPCh. 5.1 - Prob. 60STPCh. 5.1 - Prob. 61SRCh. 5.1 - Prob. 62SRCh. 5.1 - Prob. 63SRCh. 5.1 - Prob. 64SRCh. 5.1 - Prob. 65SRCh. 5.1 - Prob. 66SRCh. 5.1 - Prob. 67SRCh. 5.1 - Prob. 68SRCh. 5.1 - Prob. 69SRCh. 5.1 - Prob. 70SRCh. 5.1 - Prob. 71SRCh. 5.1 - Prob. 72SRCh. 5.1 - Prob. 73SRCh. 5.1 - Prob. 74SCh. 5.1 - Prob. 75SCh. 5.1 - Prob. 76SCh. 5.1 - Prob. 77SCh. 5.1 - Prob. 78SCh. 5.1 - Prob. 79SCh. 5.1 - Prob. 80SCh. 5.1 - Prob. 81SCh. 5.2 - Prob. 1CYPCh. 5.2 - Prob. 2ACYPCh. 5.2 - Prob. 2BCYPCh. 5.2 - Prob. 2CCYPCh. 5.2 - Prob. 2DCYPCh. 5.2 - Prob. 3ACYPCh. 5.2 - Prob. 3BCYPCh. 5.2 - Prob. 3CCYPCh. 5.2 - Prob. 3DCYPCh. 5.2 - Prob. 1CYUCh. 5.2 - Prob. 2CYUCh. 5.2 - Prob. 3CYUCh. 5.2 - Prob. 4CYUCh. 5.2 - Prob. 5CYUCh. 5.2 - Prob. 6CYUCh. 5.2 - Prob. 7CYUCh. 5.2 - Prob. 8CYUCh. 5.2 - Prob. 9CYUCh. 5.2 - Prob. 10PPSCh. 5.2 - Prob. 11PPSCh. 5.2 - Prob. 12PPSCh. 5.2 - Prob. 13PPSCh. 5.2 - Prob. 14PPSCh. 5.2 - Prob. 15PPSCh. 5.2 - Prob. 16PPSCh. 5.2 - Prob. 17PPSCh. 5.2 - Prob. 18PPSCh. 5.2 - Prob. 19PPSCh. 5.2 - Prob. 20PPSCh. 5.2 - Prob. 21PPSCh. 5.2 - Prob. 22PPSCh. 5.2 - Prob. 23PPSCh. 5.2 - Prob. 24PPSCh. 5.2 - Prob. 25PPSCh. 5.2 - Prob. 26PPSCh. 5.2 - Prob. 27PPSCh. 5.2 - Prob. 28PPSCh. 5.2 - Prob. 29PPSCh. 5.2 - Prob. 30PPSCh. 5.2 - Prob. 31PPSCh. 5.2 - Prob. 32PPSCh. 5.2 - Prob. 33PPSCh. 5.2 - Prob. 34PPSCh. 5.2 - Prob. 35PPSCh. 5.2 - Prob. 36PPSCh. 5.2 - Prob. 37PPSCh. 5.2 - Prob. 38PPSCh. 5.2 - Prob. 39PPSCh. 5.2 - Prob. 40PPSCh. 5.2 - Prob. 41PPSCh. 5.2 - Prob. 42HPCh. 5.2 - Prob. 43HPCh. 5.2 - Prob. 44HPCh. 5.2 - Prob. 45HPCh. 5.2 - Prob. 46HPCh. 5.2 - Prob. 47HPCh. 5.2 - Prob. 48STPCh. 5.2 - Prob. 49STPCh. 5.2 - Prob. 50STPCh. 5.2 - Prob. 51STPCh. 5.2 - Prob. 52SRCh. 5.2 - Prob. 53SRCh. 5.2 - Prob. 54SRCh. 5.2 - Prob. 55SRCh. 5.2 - Prob. 56SRCh. 5.2 - Prob. 57SRCh. 5.2 - Prob. 58SRCh. 5.2 - Prob. 59SRCh. 5.2 - Prob. 60SRCh. 5.2 - Prob. 61SRCh. 5.2 - Prob. 62SRCh. 5.2 - Prob. 63SCh. 5.2 - Prob. 64SCh. 5.2 - Prob. 65SCh. 5.2 - Prob. 66SCh. 5.2 - Prob. 67SCh. 5.2 - Prob. 68SCh. 5.3 - Prob. 1CYPCh. 5.3 - Prob. 2ACYPCh. 5.3 - Prob. 2BCYPCh. 5.3 - Prob. 3CYPCh. 5.3 - Prob. 4ACYPCh. 5.3 - Prob. 4BCYPCh. 5.3 - Prob. 5ACYPCh. 5.3 - Prob. 5BCYPCh. 5.3 - Prob. 1CYUCh. 5.3 - Prob. 2CYUCh. 5.3 - Prob. 3CYUCh. 5.3 - Prob. 4CYUCh. 5.3 - Prob. 5CYUCh. 5.3 - Prob. 6CYUCh. 5.3 - Prob. 7CYUCh. 5.3 - Prob. 8CYUCh. 5.3 - Prob. 9CYUCh. 5.3 - Prob. 10CYUCh. 5.3 - Prob. 11CYUCh. 5.3 - Prob. 12PPSCh. 5.3 - Prob. 13PPSCh. 5.3 - Prob. 14PPSCh. 5.3 - Prob. 15PPSCh. 5.3 - Prob. 16PPSCh. 5.3 - Prob. 17PPSCh. 5.3 - Prob. 18PPSCh. 5.3 - Prob. 19PPSCh. 5.3 - Prob. 20PPSCh. 5.3 - Prob. 21PPSCh. 5.3 - Prob. 22PPSCh. 5.3 - Prob. 23PPSCh. 5.3 - Prob. 24PPSCh. 5.3 - Prob. 25PPSCh. 5.3 - Prob. 26PPSCh. 5.3 - Prob. 27PPSCh. 5.3 - Prob. 28PPSCh. 5.3 - Prob. 29PPSCh. 5.3 - Prob. 30PPSCh. 5.3 - Prob. 31PPSCh. 5.3 - Prob. 32PPSCh. 5.3 - Prob. 33PPSCh. 5.3 - Prob. 34PPSCh. 5.3 - Prob. 35PPSCh. 5.3 - Prob. 36PPSCh. 5.3 - Prob. 37PPSCh. 5.3 - Prob. 38PPSCh. 5.3 - Prob. 39PPSCh. 5.3 - Prob. 40PPSCh. 5.3 - Prob. 41PPSCh. 5.3 - Prob. 42PPSCh. 5.3 - Prob. 43PPSCh. 5.3 - Prob. 44PPSCh. 5.3 - Prob. 45PPSCh. 5.3 - Prob. 46PPSCh. 5.3 - Prob. 47PPSCh. 5.3 - Prob. 48PPSCh. 5.3 - Prob. 49PPSCh. 5.3 - Prob. 50PPSCh. 5.3 - Prob. 51PPSCh. 5.3 - Prob. 52PPSCh. 5.3 - Prob. 53PPSCh. 5.3 - Prob. 54PPSCh. 5.3 - Prob. 55HPCh. 5.3 - Prob. 56HPCh. 5.3 - Prob. 57HPCh. 5.3 - Prob. 58HPCh. 5.3 - Prob. 59HPCh. 5.3 - Prob. 60STPCh. 5.3 - Prob. 61STPCh. 5.3 - Prob. 62STPCh. 5.3 - Prob. 63STPCh. 5.3 - Prob. 64SRCh. 5.3 - Prob. 65SRCh. 5.3 - Prob. 66SRCh. 5.3 - Prob. 67SRCh. 5.3 - Prob. 68SRCh. 5.3 - Prob. 69SRCh. 5.3 - Prob. 70SRCh. 5.3 - Prob. 71SRCh. 5.3 - Prob. 72SRCh. 5.3 - Prob. 73SRCh. 5.3 - Prob. 74SRCh. 5.3 - Prob. 75SRCh. 5.3 - Prob. 76SRCh. 5.3 - Prob. 77SRCh. 5.3 - Prob. 78SCh. 5.3 - Prob. 79SCh. 5.3 - Prob. 80SCh. 5.3 - Prob. 81SCh. 5.3 - Prob. 82SCh. 5.3 - Prob. 83SCh. 5.4 - Prob. 1ACYPCh. 5.4 - Prob. 1BCYPCh. 5.4 - Prob. 2BCYPCh. 5.4 - Prob. 3ACYPCh. 5.4 - Prob. 3BCYPCh. 5.4 - Prob. 1CYUCh. 5.4 - Prob. 2CYUCh. 5.4 - Prob. 3CYUCh. 5.4 - Prob. 4CYUCh. 5.4 - Prob. 5CYUCh. 5.4 - Prob. 6PPSCh. 5.4 - Prob. 7PPSCh. 5.4 - Prob. 8PPSCh. 5.4 - Prob. 9PPSCh. 5.4 - Prob. 10PPSCh. 5.4 - Prob. 11PPSCh. 5.4 - Prob. 12PPSCh. 5.4 - Prob. 13PPSCh. 5.4 - Prob. 14PPSCh. 5.4 - Prob. 15PPSCh. 5.4 - Prob. 16PPSCh. 5.4 - Prob. 17PPSCh. 5.4 - Prob. 18PPSCh. 5.4 - Prob. 19PPSCh. 5.4 - Prob. 20PPSCh. 5.4 - Prob. 21PPSCh. 5.4 - Prob. 22PPSCh. 5.4 - Prob. 23PPSCh. 5.4 - Prob. 24PPSCh. 5.4 - Prob. 25PPSCh. 5.4 - Prob. 26PPSCh. 5.4 - Prob. 27PPSCh. 5.4 - Prob. 28PPSCh. 5.4 - Prob. 29PPSCh. 5.4 - Prob. 30PPSCh. 5.4 - Prob. 31PPSCh. 5.4 - Prob. 32PPSCh. 5.4 - Prob. 33PPSCh. 5.4 - Prob. 34PPSCh. 5.4 - Prob. 35PPSCh. 5.4 - Prob. 36PPSCh. 5.4 - Prob. 37PPSCh. 5.4 - Prob. 38PPSCh. 5.4 - Prob. 39HPCh. 5.4 - Prob. 40HPCh. 5.4 - Prob. 41HPCh. 5.4 - Prob. 42HPCh. 5.4 - Prob. 43HPCh. 5.4 - Prob. 44STPCh. 5.4 - Prob. 45STPCh. 5.4 - Prob. 46STPCh. 5.4 - Prob. 47STPCh. 5.4 - Prob. 48SRCh. 5.4 - Prob. 49SRCh. 5.4 - Prob. 50SRCh. 5.4 - Prob. 51SRCh. 5.4 - Prob. 52SRCh. 5.4 - Prob. 53SRCh. 5.4 - Prob. 54SRCh. 5.4 - Prob. 55SRCh. 5.4 - Prob. 56SRCh. 5.4 - Prob. 57SRCh. 5.4 - Prob. 58SRCh. 5.4 - Prob. 59SRCh. 5.4 - Prob. 60SRCh. 5.4 - Prob. 61SRCh. 5.4 - Prob. 62SRCh. 5.4 - Prob. 63SRCh. 5.4 - Prob. 64SCh. 5.4 - Prob. 65SCh. 5.4 - Prob. 66SCh. 5.4 - Prob. 67SCh. 5.4 - Prob. 68SCh. 5.4 - Prob. 69SCh. 5.4 - Prob. 70SCh. 5.4 - Prob. 71SCh. 5.4 - Prob. 72SCh. 5.5 - Prob. 1BCYPCh. 5.5 - Prob. 2CYPCh. 5.5 - Prob. 3ACYPCh. 5.5 - Prob. 3BCYPCh. 5.5 - Prob. 20PPSCh. 5.5 - Prob. 24PPSCh. 5.5 - Prob. 25PPSCh. 5.5 - Prob. 26PPSCh. 5.5 - Prob. 27PPSCh. 5.5 - Prob. 28PPSCh. 5.5 - Prob. 29PPSCh. 5.5 - Prob. 32PPSCh. 5.5 - Prob. 33PPSCh. 5.5 - Prob. 34PPSCh. 5.5 - Prob. 35PPSCh. 5.5 - Prob. 36PPSCh. 5.5 - Prob. 37PPSCh. 5.5 - Prob. 38PPSCh. 5.5 - Prob. 39PPSCh. 5.5 - Prob. 40PPSCh. 5.5 - Prob. 41PPSCh. 5.5 - Prob. 43HPCh. 5.5 - Prob. 44HPCh. 5.5 - Prob. 45HPCh. 5.5 - Prob. 1ACYPCh. 5.5 - Prob. 1CYUCh. 5.5 - Prob. 2CYUCh. 5.5 - Prob. 3CYUCh. 5.5 - Prob. 4CYUCh. 5.5 - Prob. 5CYUCh. 5.5 - Prob. 6CYUCh. 5.5 - Prob. 7CYUCh. 5.5 - Prob. 8PPSCh. 5.5 - Prob. 9PPSCh. 5.5 - Prob. 10PPSCh. 5.5 - Prob. 11PPSCh. 5.5 - Prob. 12PPSCh. 5.5 - Prob. 13PPSCh. 5.5 - Prob. 14PPSCh. 5.5 - Prob. 15PPSCh. 5.5 - Prob. 16PPSCh. 5.5 - Prob. 17PPSCh. 5.5 - Prob. 18PPSCh. 5.5 - Prob. 19PPSCh. 5.5 - Prob. 21PPSCh. 5.5 - Prob. 22PPSCh. 5.5 - Prob. 23PPSCh. 5.5 - Prob. 30PPSCh. 5.5 - Prob. 31PPSCh. 5.5 - Prob. 42PPSCh. 5.5 - Prob. 46HPCh. 5.5 - Prob. 47HPCh. 5.5 - Prob. 48STPCh. 5.5 - Prob. 49STPCh. 5.5 - Prob. 50STPCh. 5.5 - Prob. 51STPCh. 5.5 - Prob. 52SRCh. 5.5 - Prob. 53SRCh. 5.5 - Prob. 54SRCh. 5.5 - Prob. 55SRCh. 5.5 - Prob. 56SRCh. 5.5 - Prob. 57SRCh. 5.5 - Prob. 58SRCh. 5.5 - Prob. 59SRCh. 5.5 - Prob. 60SRCh. 5.5 - Prob. 61SCh. 5.5 - Prob. 62SCh. 5.5 - Prob. 63SCh. 5.5 - Prob. 64SCh. 5.5 - Prob. 65SCh. 5.5 - Prob. 66SCh. 5.5 - Prob. 67SCh. 5.5 - Prob. 68SCh. 5.6 - Prob. 13PPSCh. 5.6 - Prob. 14PPSCh. 5.6 - Prob. 15PPSCh. 5.6 - Prob. 16PPSCh. 5.6 - Prob. 17PPSCh. 5.6 - Prob. 20PPSCh. 5.6 - Prob. 21PPSCh. 5.6 - Prob. 22PPSCh. 5.6 - Prob. 25PPSCh. 5.6 - Prob. 1ACYPCh. 5.6 - Prob. 1BCYPCh. 5.6 - Prob. 2ACYPCh. 5.6 - Prob. 2BCYPCh. 5.6 - Prob. 3ACYPCh. 5.6 - Prob. 3BCYPCh. 5.6 - Prob. 4CYPCh. 5.6 - Prob. 1CYUCh. 5.6 - Prob. 2CYUCh. 5.6 - Prob. 3CYUCh. 5.6 - Prob. 4CYUCh. 5.6 - Prob. 5CYUCh. 5.6 - Prob. 6CYUCh. 5.6 - Prob. 7CYUCh. 5.6 - Prob. 8CYUCh. 5.6 - Prob. 9CYUCh. 5.6 - Prob. 10CYUCh. 5.6 - Prob. 11CYUCh. 5.6 - Prob. 12PPSCh. 5.6 - Prob. 18PPSCh. 5.6 - Prob. 19PPSCh. 5.6 - Prob. 23PPSCh. 5.6 - Prob. 24PPSCh. 5.6 - Prob. 26PPSCh. 5.6 - Prob. 27PPSCh. 5.6 - Prob. 28PPSCh. 5.6 - Prob. 29PPSCh. 5.6 - Prob. 30PPSCh. 5.6 - Prob. 31PPSCh. 5.6 - Prob. 32PPSCh. 5.6 - Prob. 33PPSCh. 5.6 - Prob. 34PPSCh. 5.6 - Prob. 35PPSCh. 5.6 - Prob. 36PPSCh. 5.6 - Prob. 37PPSCh. 5.6 - Prob. 38PPSCh. 5.6 - Prob. 39PPSCh. 5.6 - Prob. 40PPSCh. 5.6 - Prob. 41PPSCh. 5.6 - Prob. 42PPSCh. 5.6 - Prob. 43PPSCh. 5.6 - Prob. 44PPSCh. 5.6 - Prob. 45PPSCh. 5.6 - Prob. 46HPCh. 5.6 - Prob. 47HPCh. 5.6 - Prob. 48HPCh. 5.6 - Prob. 49HPCh. 5.6 - Prob. 50HPCh. 5.6 - Prob. 51STPCh. 5.6 - Prob. 52STPCh. 5.6 - Prob. 53STPCh. 5.6 - Prob. 54STPCh. 5.6 - Prob. 55SRCh. 5.6 - Prob. 56SRCh. 5.6 - Prob. 57SRCh. 5.6 - Prob. 58SRCh. 5.6 - Prob. 59SRCh. 5.6 - Prob. 60SRCh. 5.6 - Prob. 61SRCh. 5.6 - Prob. 62SRCh. 5.6 - Prob. 63SRCh. 5.6 - Prob. 64SRCh. 5.6 - Prob. 65SCh. 5 - Prob. 1SGRCh. 5 - Prob. 2SGRCh. 5 - Prob. 3SGRCh. 5 - Prob. 4SGRCh. 5 - Prob. 5SGRCh. 5 - Prob. 6SGRCh. 5 - Prob. 7SGRCh. 5 - Prob. 9SGRCh. 5 - Prob. 10SGRCh. 5 - Prob. 11SGRCh. 5 - Prob. 12SGRCh. 5 - Prob. 13SGRCh. 5 - Prob. 14SGRCh. 5 - Prob. 15SGRCh. 5 - Prob. 16SGRCh. 5 - Prob. 17SGRCh. 5 - Prob. 18SGRCh. 5 - Prob. 20SGRCh. 5 - Prob. 21SGRCh. 5 - Prob. 22SGRCh. 5 - Prob. 23SGRCh. 5 - Prob. 24SGRCh. 5 - Prob. 25SGRCh. 5 - Prob. 26SGRCh. 5 - Prob. 30SGRCh. 5 - Prob. 41SGRCh. 5 - Prob. 44SGRCh. 5 - Prob. 46SGRCh. 5 - Prob. 47SGRCh. 5 - Prob. 48SGRCh. 5 - Prob. 49SGRCh. 5 - Prob. 50SGRCh. 5 - Prob. 51SGRCh. 5 - Prob. 52SGRCh. 5 - Prob. 53SGRCh. 5 - Prob. 15PTCh. 5 - Prob. 16PTCh. 5 - Prob. 19PTCh. 5 - Prob. 20PTCh. 5 - Prob. 21PTCh. 5 - Prob. 22PTCh. 5 - Prob. 23PTCh. 5 - Prob. 24PTCh. 5 - Prob. 1QCCh. 5 - Prob. 2QCCh. 5 - Prob. 3QCCh. 5 - Prob. 4QCCh. 5 - Prob. 5QCCh. 5 - Prob. 6QCCh. 5 - Prob. 7QCCh. 5 - Prob. 8QCCh. 5 - Prob. 9QCCh. 5 - Prob. 10QCCh. 5 - Prob. 11QCCh. 5 - Prob. 12QCCh. 5 - Prob. 13QCCh. 5 - Prob. 14QCCh. 5 - Prob. 15QCCh. 5 - Prob. 1MCQCh. 5 - Prob. 2MCQCh. 5 - Prob. 3MCQCh. 5 - Prob. 4MCQCh. 5 - Prob. 5MCQCh. 5 - Prob. 6MCQCh. 5 - Prob. 7MCQCh. 5 - Prob. 8MCQCh. 5 - Prob. 9MCQCh. 5 - Prob. 10MCQCh. 5 - Prob. 11MCQCh. 5 - Prob. 12MCQCh. 5 - Prob. 13MCQCh. 5 - Prob. 14MCQCh. 5 - Prob. 15MCQCh. 5 - Prob. 16MCQCh. 5 - Prob. 17MCQCh. 5 - Prob. 18MCQCh. 5 - Prob. 19MCQCh. 5 - Prob. 20MCQCh. 5 - Prob. 21MCQCh. 5 - Prob. 22MCQCh. 5 - Prob. 23MCQCh. 5 - Prob. 24MCQCh. 5 - Prob. 25MCQCh. 5 - Prob. 26MCQCh. 5 - Prob. 27MCQCh. 5 - Prob. 8SGRCh. 5 - Prob. 19SGRCh. 5 - Prob. 27SGRCh. 5 - Prob. 28SGRCh. 5 - Prob. 29SGRCh. 5 - Prob. 31SGRCh. 5 - Prob. 32SGRCh. 5 - Prob. 33SGRCh. 5 - Prob. 34SGRCh. 5 - Prob. 35SGRCh. 5 - Prob. 36SGRCh. 5 - Prob. 37SGRCh. 5 - Prob. 38SGRCh. 5 - Prob. 39SGRCh. 5 - Prob. 40SGRCh. 5 - Prob. 42SGRCh. 5 - Prob. 43SGRCh. 5 - Prob. 45SGRCh. 5 - Prob. 1PTCh. 5 - Prob. 2PTCh. 5 - Prob. 3PTCh. 5 - Prob. 4PTCh. 5 - Prob. 5PTCh. 5 - Prob. 6PTCh. 5 - Prob. 7PTCh. 5 - Prob. 8PTCh. 5 - Prob. 9PTCh. 5 - Prob. 10PTCh. 5 - Prob. 11PTCh. 5 - Prob. 12PTCh. 5 - Prob. 13PTCh. 5 - Prob. 14PTCh. 5 - Prob. 17PTCh. 5 - Prob. 18PTCh. 5 - Prob. 25PTCh. 5 - Prob. 26PTCh. 5 - Prob. 1STPCh. 5 - Prob. 2STPCh. 5 - Prob. 3STPCh. 5 - Prob. 4STPCh. 5 - Prob. 5STPCh. 5 - Prob. 6STPCh. 5 - Prob. 7STPCh. 5 - Prob. 8STPCh. 5 - Prob. 9STPCh. 5 - Prob. 10STPCh. 5 - Prob. 11STPCh. 5 - Prob. 12STPCh. 5 - Prob. 13STPCh. 5 - Prob. 14STPCh. 5 - Prob. 15STPCh. 5 - Prob. 16STPCh. 5 - Prob. 17STP

Additional Math Textbook Solutions

Find more solutions based on key concepts
In Problems 19-30, state the domain and range for each relation. Then determine whether each relation represent...

Precalculus

Inequality for life span of tortoise.

Pre-Algebra Student Edition

Assessment 1-1A How many triangles are in the following figure?

A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)

Twenty five people, consisting of 15 women and 10 men are lined up in a random order. Find the probability that...

A First Course in Probability (10th Edition)

Critical Values. In Exercises 41–44, find the indicated critical value. Round results to two decimal places. 43...

Elementary Statistics (13th Edition)

Explain the meaning of the term “statistically significant difference” in statistics terminology.

Intro Stats, Books a la Carte Edition (5th Edition)

Knowledge Booster
Background pattern image
Algebra
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Text book image
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Text book image
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Text book image
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Text book image
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
Text book image
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
SEE MORE TEXTBOOKS
2.1 Introduction to inequalities; Author: Oli Notes;https://www.youtube.com/watch?v=D6erN5YTlXE;License: Standard YouTube License, CC-BY
GCSE Maths - What are Inequalities? (Inequalities Part 1) #56; Author: Cognito;https://www.youtube.com/watch?v=e_tY6X5PwWw;License: Standard YouTube License, CC-BY
Introduction to Inequalities | Inequality Symbols | Testing Solutions for Inequalities; Author: Scam Squad Math;https://www.youtube.com/watch?v=paZSN7sV1R8;License: Standard YouTube License, CC-BY