Chapter 4, Problem 4.24P

(a)

To determine

The gravitational force on a point mass at a distance $\rho$.

Answer to Problem 4.24P

The gravitational force on a point mass at a distance $\rho$ is $\underset{\_}{\frac{-2GM\mu }{\rho }\widehat{\rho }}$.

Explanation of Solution

Linear mass density id defined as the mass per unit length.

    $\mu =\frac{M}{dz}$        (I)

Here, $M$ is the mass of the rod, $dz$ is the small element of the rod.

Write the expression for force on the point mass due to small segment.

    $dF=-\frac{GMm}{r^2}$        (II)

Here, $r$ is the distance between point mass and small segment, and $G$ is the gravitational constant.

Substitute the value of $M$ from the equation (I) in (II).

    $dF=-\frac{Gm\mu dz}{r^2}$        (III)

An infinitely long uniform rod is situated on the z axis is shown in the Figure below.

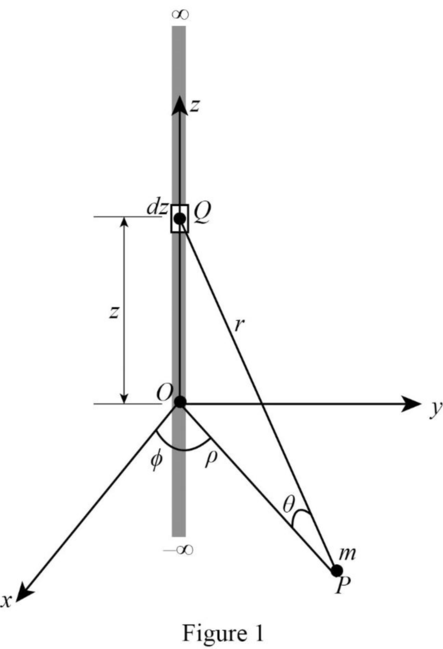
Using the figure shown above and equation (III), write the expression for force along $\rho$ direction.

    $d{F}_{\rho }=-\frac{Gm\mu }{r^2}\cos \theta dz$        (IV)

The z component of the force from –z to z will be cancel each other.

From the figure, write the expression for $\cos \theta$.

    $\cos \theta =\frac{\rho }{r}$        (V)

Integrate the equation (IV) with the interval minus infinity to plus infinity and Use equation in (V) in (IV), to get the total $\rho$ component of force.

    $\begin{array}{c}{\displaystyle \int d{F}_{\rho }}=-{\displaystyle \underset{-\infty }{\overset{\infty }{\int }}\frac{Gm\mu }{r^2}\left(\frac{\rho }{r}\right)dz}\\ F_{\rho }=-Gm\mu \rho {\displaystyle \underset{-\infty }{\overset{\infty }{\int }}\frac{dz}{r^3}}\end{array}$        (VI)

Write the expression for distance between point mass and small element from the figure.

    $r=\sqrt{{\rho }^2+z^2}$        (VII)

Use equation (VII) in (VI).

    $F_{\rho }=-Gm\mu \rho {\displaystyle \underset{-\infty }{\overset{\infty }{\int }}\frac{dz}{{\left({\rho }^2+z^2\right)}^{\frac{3}{2}}}}$        (VIII)

Use $z=\rho \tan \theta$, then $dz=\rho {\sec }^2\theta d\theta$. So the limit changes as $-\pi /2$ to $\pi /2$. Then the equation (VIII) can be rewritten as,

    $\begin{array}{c}{F}_{\rho }=-Gm\mu \rho {\displaystyle \underset{-\pi /2}{\overset{\pi /2}{\int }}\frac{\rho {\sec }^2\theta d\theta }{{\left[{\rho }^2+{\left(\rho \tan \theta \right)}^2\right]}^{\frac{3}{2}}}}\\ =-Gm\mu \rho {\displaystyle \underset{-\pi /2}{\overset{\pi /2}{\int }}\frac{\rho {\sec }^2\theta d\theta }{{\rho }^3{\left(1+{\tan }^2\theta \right)}^{\frac{3}{2}}}}\\ =-Gm\mu \rho {\displaystyle \underset{-\pi /2}{\overset{\pi /2}{\int }}\frac{{\sec }^2\theta d\theta }{{\rho }^2{\left({\sec }^2\theta \right)}^{\frac{3}{2}}}}\\ =\frac{-Gm\mu \rho }{{\rho }^2}{\displaystyle \underset{-\pi /2}{\overset{\pi /2}{\int }}\frac{d\theta }{\sec \theta }}\end{array}$        (IX)

Solve the equation (IX).

    $\begin{array}{c}{F}_{\rho }=\frac{-Gm\mu \rho }{{\rho }^2}{\displaystyle \underset{-\pi /2}{\overset{\pi /2}{\int }}\cos \theta d\theta }\\ =\frac{-Gm\mu }{\rho }{\left[\sin \theta \right]}_{-\pi /2}^{\pi /2}\\ =\frac{-Gm\mu }{\rho }\left[\sin \left(\pi /2\right)-\sin \left(-\pi /2\right)\right]\\ =\frac{-2Gm\mu }{\rho }\end{array}$        (X)

Conclusion:

Therefore, the gravitational force on a point mass at a distance $\rho$ is $\underset{\_}{\frac{-2GM\mu }{\rho }\widehat{\rho }}$.

(b)

To determine

Force in terms of rectangular coordinates of the point and verify that $\nabla \times F=0$.

Answer to Problem 4.24P

Force in terms of rectangular coordinates of the point is $\underset{\_}{-2G\mu m\left[\frac{x}{x^2+y^2}\widehat{x}+\frac{y}{x^2+y^2}\widehat{y}\right]}$ and verified that $\nabla \times F=0$.

Explanation of Solution

Write the expression for $\rho$ in rectangular coordinates.

    $\rho =\sqrt{x^2+y^2}$        (XI)

Write the expression for unit vector of $\rho$ in rectangular coordinates.

    $\widehat{\rho }=\frac{x}{\sqrt{x^2+y^2}}\widehat{x}+\frac{y}{\sqrt{x^2+y^2}}\widehat{y}$        (XII)

Write the expression for gravitational force on a point mass at a distance $\rho$ from the z axis.

    $F=\frac{-2Gm\mu }{\rho }\widehat{\rho }$        (XIII)

Use equation (XI) and (XII) in (XIII).

    $F=-2Gm\mu \left(\frac{x}{x^2+y^2}\widehat{x}+\frac{y}{x^2+y^2}\widehat{y}\right)$        (XIV)

Write the expression for curl of a function.

    $\begin{array}{c}\nabla \times F=\left(\begin{array}{ccc}\widehat{x}& \widehat{y}& \widehat{z}\\ \frac{\partial }{\partial x}& \frac{\partial }{\partial y}& \frac{\partial }{\partial z}\\ F_x& F_y& F_z\end{array}\right)\\ =\widehat{x}\left[\frac{\partial F_z}{\partial y}-\frac{\partial F_y}{\partial z}\right]-\widehat{y}\left[\frac{\partial F_z}{\partial z}-\frac{\partial F_x}{\partial x}\right]+\widehat{z}\left[\frac{\partial F_y}{\partial x}-\frac{\partial F_x}{\partial y}\right]\end{array}$        (XV)

Use equation (XIV) in (XV).

    $\begin{array}{c}\nabla \times F=\widehat{x}\left[\frac{\partial \left(0\right)}{\partial y}-\frac{\partial }{\partial z}\left(\frac{-2G\mu my}{x^2+y^2}\right)\right]-\widehat{y}\left[\frac{\partial }{\partial z}\left(\frac{-2G\mu mx}{x^2+y^2}\right)-\frac{\partial \left(0\right)}{\partial x}\right]\\ +\widehat{z}\left[\frac{\partial }{\partial x}\left(\frac{-2G\mu my}{x^2+y^2}\right)-\frac{\partial }{\partial y}\left(\frac{-2G\mu mx}{x^2+y^2}\right)\right]\\ =0\widehat{x}-0\widehat{y}+2G\mu m\left(\frac{-y\left(2x\right)}{{\left(x^2+y^2\right)}^2}-\frac{-x\left(2y\right)}{{\left(x^2+y^2\right)}^2}\right)\widehat{z}\\ =0\end{array}$        (XVI)

From the equation (XVI), it is clear that the force is conservative.

Conclusion:

Therefore, the force in terms of rectangular coordinates of the point is $\underset{\_}{-2G\mu m\left[\frac{x}{x^2+y^2}\widehat{x}+\frac{y}{x^2+y^2}\widehat{y}\right]}$ and verified that $\nabla \times F=0$.

(c)

To determine

Show that $\nabla \times F=0$ in cylindrical polar co-ordinates.

Answer to Problem 4.24P

Showed that $\nabla \times F=0$ in cylindrical polar co-ordinates.

Explanation of Solution

Write the expression for curl in cylindrical polar co-ordinates.

    $\nabla \times F=\widehat{\rho }\left[\frac{1}{\rho }\frac{\partial F_z}{\partial \varphi }-\frac{\partial F_{\varphi }}{\partial z}\right]-\widehat{\varphi }\left[\frac{\partial F_z}{\partial \rho }-\frac{\partial F_{\rho }}{\partial z}\right]+\widehat{z}\frac{1}{\rho }\left[\frac{\partial \left(\rho F_{\varphi }\right)}{\partial \rho }-\frac{\partial F_{\rho }}{\partial \varphi }\right]$        (XVII)

Use $\frac{-2G\mu m}{\rho }$ for $F_{\rho }$, and $0$ for $F_{\varphi },F_z$ in the equation (XVII).

    $\begin{array}{c}\nabla \times F=\widehat{\rho }\left[\frac{1}{\rho }\frac{\partial \left(0\right)}{\partial \varphi }-\frac{\partial \left(0\right)}{\partial z}\right]-\widehat{\varphi }\left[\frac{\partial \left(0\right)}{\partial \rho }-\frac{\partial }{\partial z}\left(\frac{-2G\mu m}{\rho }\right)\right]+\widehat{z}\frac{1}{\rho }\left[\frac{\partial \left(0\right)}{\partial \rho }-\frac{\partial }{\partial \varphi }\left(\frac{-2G\mu m}{\rho }\right)\right]\\ =0\end{array}$

Conclusion:

Therefore, $\nabla \times F=0$ in cylindrical polar co-ordinates.

(d)

To determine

The potential energy in polar coordinates.

Answer to Problem 4.24P

The potential energy is $\underset{\_}{2Gm\mu \ln \left(\frac{\rho }{{\rho }_0}\right)}$.

Explanation of Solution

Potential energy of the system of masses is the negative integral of gravitational force to bring the masses from infinite distance to a distance $r$.

    $U\left(r\right)=-{\displaystyle \underset{r_0}{\overset{r}{\int }}F\cdot dr}$        (XVIII)

Rewrite the equation (XVIII) n polar co-ordinates,.

    $U\left(r\right)=-{\displaystyle \underset{{\rho }_0}{\overset{\rho }{\int }}F\cdot d\rho }$        (XIX)

Use $-\frac{2Gm\mu }{\rho }$ for $F$ in the equation (XIX), and solve.

    $\begin{array}{c}U\left(r\right)=-{\displaystyle \underset{{\rho }_0}{\overset{\rho }{\int }}-\frac{2Gm\mu }{\rho }\cdot d\rho }\\ =2G\mu m{\displaystyle \underset{{\rho }_0}{\overset{\rho }{\int }}\frac{d\rho }{\rho }}\\ =2G\mu m{\left[\ln \right]}_{{\rho }_0}^{\rho }\\ =2G\mu m\ln \left(\frac{\rho }{{\rho }_0}\right)\end{array}$

Conclusion:

Therefore, the potential energy is $\underset{\_}{2Gm\mu \ln \left(\frac{\rho }{{\rho }_0}\right)}$.