Calculus and Its Applications (11th Edition)
11th Edition
ISBN: 9780321979391
Author: Marvin L. Bittinger, David J. Ellenbogen, Scott J. Surgent
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Question
Chapter 3.4, Problem 50E
To determine
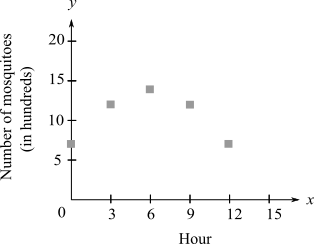
The functions from the provided options that fits the
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Exercise 1
Given are the following planes:
plane 1:
3x4y+z = 1
0
plane 2:
(s, t) =
( 2 ) + (
-2
5 s+
0
(
3 t
2
-2
a) Find for both planes the Hessian normal form and for plane 1 in addition the parameter form.
b) Use the cross product of the two normal vectors to show that the planes intersect in a line.
c) Calculate the intersection line.
d) Calculate the intersection angle of the planes. Make a sketch to indicate which angle you are
calculating.
Only 100% sure experts solve it correct complete solutions ok
rmine the immediate settlement for points A and B shown in
figure below knowing that Aq,-200kN/m², E-20000kN/m², u=0.5, Depth
of foundation (DF-0), thickness of layer below footing (H)=20m.
4m
B
2m
2m
A
2m
+
2m
4m
Chapter 3 Solutions
Calculus and Its Applications (11th Edition)
Ch. 3.1 - Graph. y=5xCh. 3.1 - Graph. y=4xCh. 3.1 - Graph. y=23xCh. 3.1 - Graph. y=34xCh. 3.1 - Graph.
5.
Ch. 3.1 - Graph.
6.
Ch. 3.1 - Prob. 7ECh. 3.1 - Prob. 8ECh. 3.1 - Prob. 9ECh. 3.1 - Graph. y=1.13(0.81)x
Ch. 3.1 - Differentiate. f(x)=exCh. 3.1 - Differentiate.
12.
Ch. 3.1 - Differentiate.
13.
Ch. 3.1 - Differentiate. g(x)=e3xCh. 3.1 - Differentiate.
15.
Ch. 3.1 - Differentiate.
16.
Ch. 3.1 - Differentiate.
17.
Ch. 3.1 - Differentiate. F(x)=e4xCh. 3.1 - Differentiate. g(x)=3e5xCh. 3.1 - Differentiate.
20.
Ch. 3.1 - Differentiate.
21.
Ch. 3.1 - Differentiate. f(x)=3exCh. 3.1 - Differentiate.
23.
Ch. 3.1 - Differentiate.
24.
Ch. 3.1 - Differentiate.
25.
Ch. 3.1 - Differentiate. g(x)=45ex3Ch. 3.1 - Differentiate. F(x)=4e2xCh. 3.1 - Differentiate.
28.
Ch. 3.1 - Differentiate.
29.
Ch. 3.1 - Differentiate. f(x)=x52e6xCh. 3.1 - Differentiate.
31.
Ch. 3.1 - Differentiate.
32.
Ch. 3.1 - Differentiate. F(x)=e2xx4Ch. 3.1 - Differentiate. g(x)=e3xx6Ch. 3.1 - Differentiate. f(x)=(x22x+2)exCh. 3.1 - Differentiate.
36.
Ch. 3.1 - Differentiate.
37.
Ch. 3.1 - Differentiate. f(x)=exx5Ch. 3.1 - Differentiate.
39.
Ch. 3.1 - Differentiate.
40.
Ch. 3.1 - Differentiate. f(x)=ex2/2Ch. 3.1 - Differentiate.
42.
Ch. 3.1 - Differentiate. y=ex7Ch. 3.1 - Differentiate.
44.
Ch. 3.1 - Differentiate.
45.
Ch. 3.1 - Differentiate.
46.
Ch. 3.1 - Differentiate. y=ex+x3xexCh. 3.1 - Prob. 48ECh. 3.1 - Differentiate. y=1e3xCh. 3.1 - Differentiate. y=1exCh. 3.1 - Differentiate. y=1ekxCh. 3.1 - Differentiate. y=1emxCh. 3.1 - Differentiate. g(x)=(4x2+3x)ex27xCh. 3.1 - Differentiate.
54.
Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Graph each function. Then determine any critical...Ch. 3.1 - Prob. 65ECh. 3.1 - Prob. 66ECh. 3.1 - Prob. 67ECh. 3.1 - Prob. 68ECh. 3.1 - Prob. 69ECh. 3.1 - Prob. 70ECh. 3.1 - a. 65-74. For each function given in Exercises...Ch. 3.1 - Prob. 72ECh. 3.1 - Prob. 73ECh. 3.1 - a. 65-74. For each function given in Exercises...Ch. 3.1 - Find the slope of the line tangent to the graph of...Ch. 3.1 - Find the slope of the line tangent to the graph of...Ch. 3.1 - 77. Find an equation of the line tangent to the...Ch. 3.1 - Find an equation of the line tangent to the graph...Ch. 3.1 - For each of Exercises 77 and 78, graph the...Ch. 3.1 - For each of Exercises 77 and 78, graph the...Ch. 3.1 - 81. U.S. Travel Exports. U.S. travel exports...Ch. 3.1 - Organic food. More Americans are buying organic...Ch. 3.1 - 83. Marginal Cost. The total cost, in millions of...Ch. 3.1 - Marginal cost. The total cost, in millions of...Ch. 3.1 - 85. Marginal demand. At a price of x dollars, the...Ch. 3.1 - 86. Marginal supply. At a price of x dollars, the...Ch. 3.1 - For Exercises 87-90, use the Tangent feature from...Ch. 3.1 - For Exercises 87-90, use the Tangent feature from...Ch. 3.1 - For Exercises 87-90, use the Tangent feature from...Ch. 3.1 - For Exercises 87-90, use the Tangent feature from...Ch. 3.1 - Medication concentration. The concentration C, in...Ch. 3.1 - 92. Ebbinghaus learning model. Suppose that you...Ch. 3.1 - Differentiate. y=(e3x+1)5Ch. 3.1 - Prob. 94ECh. 3.1 - Prob. 95ECh. 3.1 - Differentiate.
96.
Ch. 3.1 - Differentiate. f(x)=ex/2x1Ch. 3.1 - Differentiate. f(x)=xex1+x2Ch. 3.1 - Differentiate. f(x)=exexex+exCh. 3.1 - Differentiate.
100.
Ch. 3.1 - 101. Use the results from Exercises 85 and 86 to...Ch. 3.1 - Exercises 102 and 103 each give an expression for...Ch. 3.1 - Prob. 103ECh. 3.1 - Prob. 104ECh. 3.1 - A student made the following error on test:...Ch. 3.1 - Prob. 106ECh. 3.1 - Prob. 107ECh. 3.1 - Prob. 108ECh. 3.1 - For each of the functions in Exercises 109 – 112,...Ch. 3.1 - For each of the functions in Exercises 109 – 112,...Ch. 3.1 - For each of the functions in Exercises 109 – 112,...Ch. 3.1 - For each of the functions in Exercises 109 – 112,...Ch. 3.1 - 113. Graph
Use the Table feature and very large...Ch. 3.1 - Prob. 114ECh. 3.2 - Write an equivalent equation.
1.
Ch. 3.2 - Write an equivalent equation.
2.
Ch. 3.2 - Write an equivalent equation. log273=13Ch. 3.2 - Write an equivalent equation.
4.
Ch. 3.2 - Write an equivalent equation. logaJ=KCh. 3.2 - Write an equivalent equation.
6.
Ch. 3.2 - Write an equivalent equation. logbV=wCh. 3.2 - Write an equivalent equation. log10h=pCh. 3.2 - Solve for x. log749=xCh. 3.2 - Solve for x. log5125=xCh. 3.2 - Solve for x.
11.
Ch. 3.2 - Solve for x. logx64=3Ch. 3.2 - Solve for x. log3x=5Ch. 3.2 - Solve for x.
14.
Ch. 3.2 - Solve for x.
15.
Ch. 3.2 - Solve for x.
16.
Ch. 3.2 - Write an equivalent logarithmic equation. et=pCh. 3.2 - Write an equivalent logarithmic equation.
18.
Ch. 3.2 - Write an equivalent logarithmic equation.
19.
Ch. 3.2 - Write an equivalent logarithmic equation. 102=100Ch. 3.2 - Write an equivalent logarithmic equation. 102=0.01Ch. 3.2 - Write an equivalent logarithmic equation. 101=0.1Ch. 3.2 - Write an equivalent logarithmic equation.
23.
Ch. 3.2 - Write an equivalent logarithmic equation.
24.
Ch. 3.2 - Given logb3=1.099 and logb5=1.609, find each...Ch. 3.2 - Given and , find each value.
26.
Ch. 3.2 - Given logb3=1.099 and logb5=1.609, find each...Ch. 3.2 - Given logb3=1.099 and logb5=1.609, find each...Ch. 3.2 - Given logb3=1.099 and logb5=1.609, find each...Ch. 3.2 - Given and , find each value.
30.
Ch. 3.2 - Given and , find each value. Do not use
31.
Ch. 3.2 - Given ln4=1.3863 and ln5=1.6094, find each value....Ch. 3.2 - Given ln4=1.3863 and ln5=1.6094, find each value....Ch. 3.2 - Given and , find each value. Do not use
34.
Ch. 3.2 - Given and , find each value. Do not use
35.
Ch. 3.2 - Given and , find each value. Do not use
36.
Ch. 3.2 - Given and , find each value. Do not use
37.
Ch. 3.2 - Given and , find each value. Do not use
38.
Ch. 3.2 - Given and , find each value. Do not use
39.
Ch. 3.2 - Given ln4=1.3863 and ln5=1.6094, find each value....Ch. 3.2 - Given and , find each value. Do not use
41.
Ch. 3.2 - Given and , find each value. Do not use
42.
Ch. 3.2 - Find each logarithm. Round to six decimal...Ch. 3.2 - Find each logarithm. Round to six decimal places....Ch. 3.2 - Find each logarithm. Round to six decimal...Ch. 3.2 - Find each logarithm. Round to six decimal...Ch. 3.2 - Find each logarithm. Round to six decimal...Ch. 3.2 - Find each logarithm. Round to six decimal places....Ch. 3.2 - Solve for t.
49.
Ch. 3.2 - Solve for t. et=10Ch. 3.2 - Solve for t. e3t=900Ch. 3.2 - Solve for t. e2t=1000Ch. 3.2 - Solve for t. et=0.01Ch. 3.2 - Solve for t.
54.
Ch. 3.2 - Solve for t. e0.02t=0.06Ch. 3.2 - Solve for t.
56.
Ch. 3.2 - Differentiate y=9lnxCh. 3.2 - Differentiate y=8lnxCh. 3.2 - Differentiate y=7ln|x|Ch. 3.2 - Differentiate y=4ln|x|Ch. 3.2 - Differentiate y=x6lnx14x4Ch. 3.2 - Differentiate
62.
Ch. 3.2 - Differentiate f(x)=ln(9x)Ch. 3.2 - Differentiate
64.
Ch. 3.2 - Differentiate f(x)=ln|5x|Ch. 3.2 - Differentiate f(x)=ln|10x|Ch. 3.2 - Differentiate g(x)=x5ln(3x)Ch. 3.2 - Differentiate
68.
Ch. 3.2 - Differentiate g(x)=x4ln|6x|Ch. 3.2 - Differentiate
70.
Ch. 3.2 - Differentiate
71.
Ch. 3.2 - Differentiate y=lnxx4Ch. 3.2 - Differentiate y=ln|3x|x2Ch. 3.2 - Differentiate
74.
Ch. 3.2 - Differentiate
75.
Ch. 3.2 - Differentiate
76.
Ch. 3.2 - Differentiate y=ln(3x2+2x1)Ch. 3.2 - Differentiate
78.
Ch. 3.2 - Differentiate
79.
Ch. 3.2 - Differentiate f(x)=ln(x2+5X)Ch. 3.2 - Differentiate g(x)=exlnx2Ch. 3.2 - Differentiate g(x)=e2xlnxCh. 3.2 - Differentiate
83.
Ch. 3.2 - Differentiate f(x)=ln(ex2)Ch. 3.2 - Differentiate g(x)=(lnx)4 (Hint: Use the Extended...Ch. 3.2 - Differentiate
86.
Ch. 3.2 - Differentiate f(x)=ln(ln(8x))Ch. 3.2 - Differentiate f(x)=ln(ln(3x))Ch. 3.2 - Differentiate
89.
Ch. 3.2 - Differentiate g(x)=ln(2x)ln(7x)Ch. 3.2 - 91. Find the equation of the line tangent to the...Ch. 3.2 -
92. Find the equation of the line tangent to the...Ch. 3.2 - Find the equation of the line tangent to the graph...Ch. 3.2 - Find the equation of the line tangent to the graph...Ch. 3.2 - Business and Economics
95. Advertising. A model...Ch. 3.2 - Business and Economics
96. Advertising. A model...Ch. 3.2 - An advertising model. Solve Example 10 if the...Ch. 3.2 - Business and Economics
98. An advertising model....Ch. 3.2 - Prob. 99ECh. 3.2 - Growth of a stock. The value, V(t), in dollars, of...Ch. 3.2 - Business and Economics
101. Marginal Profit. The...Ch. 3.2 - 102. Acceptance of a new medicine. The percentage...Ch. 3.2 - Social Sciences
103. Forgetting. Students in a...Ch. 3.2 - Social Sciences
104. Forgetting. As part of a...Ch. 3.2 - Social Sciences Walking speed. Bornstein and...Ch. 3.2 - Social Sciences Hullian learning model. A...Ch. 3.2 - 107. Solve for t.
Ch. 3.2 - Differentiate. f(x)=ln(x3+1)5Ch. 3.2 - Differentiate.
109.
Ch. 3.2 - Differentiate.
110.
Ch. 3.2 - Differentiate.
111.
Ch. 3.2 - Differentiate. f(x)=log5xCh. 3.2 - Differentiate. f(x)=log7xCh. 3.2 - Differentiate. y=ln5+x2Ch. 3.2 - Prob. 116ECh. 3.2 - Prob. 117ECh. 3.2 - Prob. 118ECh. 3.2 - To prove Proprieties P1, P2, P3, and P7 of Theorem...Ch. 3.2 - To prove Proprieties P1, P2, P3, and P7 of Theorem...Ch. 3.2 - To prove Proprieties P1, P2, P3, and P7 of Theorem...Ch. 3.2 - To prove Proprieties P1, P2, P3, and P7 of Theorem...Ch. 3.2 - Prob. 124ECh. 3.2 - Prob. 125ECh. 3.2 - 126. Explain why is not defined. (Hint: Rewrite...Ch. 3.2 - Prob. 127ECh. 3.2 - Prob. 128ECh. 3.2 - Prob. 129ECh. 3.3 - 1. Find the general form of if .
Ch. 3.3 - 2. Find the general form of g if.
Ch. 3.3 - 3. Find the general form of the function that...Ch. 3.3 - Find the general form of the function that...Ch. 3.3 - Find the general form of the function that...Ch. 3.3 - Find the general form of the function that...Ch. 3.3 - U.S. patents. The number of applications for...Ch. 3.3 - 8. Franchise Expansion. Pete Zah’s is selling...Ch. 3.3 - Compound Interest. If an amount P0 is invested in...Ch. 3.3 - 10. Compound interest. If an amount is invested...Ch. 3.3 - 11. Bottled Water Sales. Since 2000, sales of...Ch. 3.3 - Annual net sales. Green Mountain Coffee Roasters...Ch. 3.3 - Annual interest rate. Euler Bank advertises that...Ch. 3.3 - 14. Annual interest rate. Hardy Bank advertises...Ch. 3.3 - Oil demand. The growth rate of the demand for oil...Ch. 3.3 - Coal demand. The growth rate of the demand for...Ch. 3.3 - Interest compounded continuously.
For Exercises...Ch. 3.3 - Interest compounded continuously. For Exercises...Ch. 3.3 - Interest compounded continuously. For Exercises...Ch. 3.3 - Interest compounded continuously. For Exercises...Ch. 3.3 - 21. Art masterpieces. In 2004, a collector paid...Ch. 3.3 - 22. Per capita income. In 2009, U.S. per capita...Ch. 3.3 - 23. Federal receipts. In 2011, U.S. federal...Ch. 3.3 - Consumer price index. The consumer price index...Ch. 3.3 - Total mobile data traffic. The following graph...Ch. 3.3 - Total mobile data traffic. The following graph...Ch. 3.3 - Value of Manhattan Island. Peter Minuit of the...Ch. 3.3 - 28. Total Revenue. Intel, a computer chip...Ch. 3.3 -
29. The U.S. Forever Stamp. The U.S. Postal...Ch. 3.3 - Prob. 30ECh. 3.3 - Effect of advertising. Suppose that SpryBorg Inc....Ch. 3.3 - Cost of a Hershey bar. The cost of a Hershey bar...Ch. 3.3 - Superman comic book. In August 2014, a 1938 comic...Ch. 3.3 - 34. Batman comic book. Refer to Example 6. In what...Ch. 3.3 - Batman comic book. Refer to Example 6. In what...Ch. 3.3 - Population Growth
For Exercise 36-40, complete the...Ch. 3.3 - Population Growth
For Exercise 36-40, complete the...Ch. 3.3 - Population Growth For Exercise 36-40, complete the...Ch. 3.3 - Population Growth For Exercise 36-40, complete the...Ch. 3.3 - Population Growth
For Exercise 36-40, complete the...Ch. 3.3 - Bicentennial growth of the United States. The...Ch. 3.3 - Limited population growth: Human Population....Ch. 3.3 - 43. Limited population growth: tortoise...Ch. 3.3 - 44. Limited population growth. A lake is stocked...Ch. 3.3 - Women college graduates. The number of women...Ch. 3.3 - Hullian learning model. The Hullian learning model...Ch. 3.3 - Spread of infection. Spread by skin-to-skin...Ch. 3.3 - 48. Diffusion of information. Pharmaceutical firms...Ch. 3.3 - 49. Spread of a rumor. The rumor “People who study...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - We have now studied models for linear, quadratic,...Ch. 3.3 - 61. Find an expression relating the exponential...Ch. 3.3 - Find an expression relating the exponential growth...Ch. 3.3 - 63. Quantity grows exponentially with a doubling...Ch. 3.3 - 64. To what exponential growth rate per hour does...Ch. 3.3 - 65. Complete the table below, which relates growth...Ch. 3.3 - Describe the differences in the graphs of an...Ch. 3.3 - Estimate the time needed for an amount of money to...Ch. 3.3 - 68. Estimate the time needed for the population in...Ch. 3.3 - Using a calculator, find the exact doubling times...Ch. 3.3 - 70. Describe two situations where it would be...Ch. 3.3 - Business: total revenue. The revenue of Red Rock,...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - In Exercise 1-8, find the half-line for each...Ch. 3.4 - Life and Physical Sciences Radioactive Decay....Ch. 3.4 - Life and Physical Sciences
10. Radioactive Decay....Ch. 3.4 - Life and Physical Sciences
11. Chemistry....Ch. 3.4 - Life and Physical Sciences Chemistry. Substance A...Ch. 3.4 - Radioactive Decay.
For Exercises 13-16, complete...Ch. 3.4 - Radioactive Decay. For Exercises 13-16, complete...Ch. 3.4 - Radioactive Decay.
For Exercises 13-16, complete...Ch. 3.4 - Radioactive Decay.
For Exercises 13-16, complete...Ch. 3.4 - Half-life. Of an initial amount of 1000g of...Ch. 3.4 - Half-life. Of an initial amount of 1000g of...Ch. 3.4 - Prob. 19ECh. 3.4 - Prob. 20ECh. 3.4 - 21. Cancer Treatment. Iodine-125 is often used to...Ch. 3.4 - Prob. 22ECh. 3.4 - Carbon Dating. Recently, while digging in Chaco...Ch. 3.4 - Present value. Following the birth of a child, a...Ch. 3.4 - Present value. Following the birth of their child,...Ch. 3.4 - Present value. Desmond wants to have $15,000...Ch. 3.4 - 27. Sports salaries. An athlete signs a contract...Ch. 3.4 - 28. Actor’s salaries. An actor signs a film...Ch. 3.4 - 29. Estate planning. Shannon has a trust fund that...Ch. 3.4 - 30. Supply and demand. The supply and demand for...Ch. 3.4 - Salvage value. Lucas Mining estimates that the...Ch. 3.4 - 32. Salvage value. Wills Investments tracks the...Ch. 3.4 - 33. Actuarial Science. An actuary works for an...Ch. 3.4 - Actuarial science. Use the formula from Exercise...Ch. 3.4 - U.S. farms. The number N of farms in the United...Ch. 3.4 - Prob. 36ECh. 3.4 - 37. Decline in beef consumption. Annual...Ch. 3.4 - Population decrease of russia. The population of...Ch. 3.4 - Population decrease of Ukraine. The population of...Ch. 3.4 - 40. Cooling. After warming the water in a hot tub...Ch. 3.4 - 41. Cooling. The temperature in a whirlpool bath...Ch. 3.4 - Forensics. A coroner arrives at a murder scene at...Ch. 3.4 - 43. Forensics. A coroner arrives at 11 p.m. She...Ch. 3.4 - Prisoner-of-war protest. The initial weight of a...Ch. 3.4 - 45. Political Protest. A monk weighing 170 lb...Ch. 3.4 - 46. Atmospheric Pressure. Atmospheric pressure P...Ch. 3.4 - 47. Satellite power. The power supply of a...Ch. 3.4 - Cases of tuberculosis. The number of cases N of...Ch. 3.4 - For each of the scatterplots in Exercise 49-58,...Ch. 3.4 - Prob. 50ECh. 3.4 - Prob. 51ECh. 3.4 - Prob. 52ECh. 3.4 - Prob. 53ECh. 3.4 - For each of the scatterplots in Exercise 49-58,...Ch. 3.4 - Prob. 55ECh. 3.4 - Prob. 56ECh. 3.4 - For each of the scatterplots in Exercise 49-58,...Ch. 3.4 - Prob. 58ECh. 3.4 - A sample of an element lost 25% of its mass in 5...Ch. 3.4 - 60. A vehicle lost 15% of its value in 2 yr....Ch. 3.4 - 61. Economics: supply and demand elasticity. The...Ch. 3.4 - The Beer-Lambert Law. A beam of light enters a...Ch. 3.4 - The Beer-Lambert Law. A beam of light enters a...Ch. 3.4 - An interest rate decreases from 8% to 7.2%....Ch. 3.4 - Prob. 66ECh. 3.5 - Differentiate.
1.
Ch. 3.5 - Differentiate. y=7xCh. 3.5 - Differentiate. f(x)=8xCh. 3.5 - Differentiate.
4.
Ch. 3.5 - Differentiate. g(x)=x5(3.7)xCh. 3.5 - Differentiate. g(x)=x3(5.4)xCh. 3.5 - Differentiate. y=7x4+2Ch. 3.5 - Differentiate.
8.
Ch. 3.5 - Differentiate.
9.
Ch. 3.5 - Prob. 10ECh. 3.5 - Differentiate. f(x)=3x4+1Ch. 3.5 - Differentiate. f(x)=127x4Ch. 3.5 - Differentiate. y=log8xCh. 3.5 - Differentiate. y=log4xCh. 3.5 - Differentiate. y=log17xCh. 3.5 - Prob. 16ECh. 3.5 - Differentiate. g(x)=log32(9x2)Ch. 3.5 - Differentiate. g(x)=log6(5x+1)Ch. 3.5 - Differentiate. F(x)=log(6x7)Ch. 3.5 - Differentiate.
20.
Ch. 3.5 - Differentiate.
21.
Ch. 3.5 - Differentiate.
22.
Ch. 3.5 - Differentiate. f(x)=4log7(x2)Ch. 3.5 - Differentiate. g(x)=log6(x3+5)Ch. 3.5 - Differentiate.
25.
Ch. 3.5 - Differentiate.
26.
Ch. 3.5 - Differentiate. G(x)=(log12x)5Ch. 3.5 - Prob. 28ECh. 3.5 - Differentiate.
29.
Ch. 3.5 - Differentiate.
30.
Ch. 3.5 - Differentiate. y=52x31log(6x+5)Ch. 3.5 - Prob. 32ECh. 3.5 - Differentiate.
33.
Ch. 3.5 - Differentiate.
34.
Ch. 3.5 - Differentiate. f(x)=(3x5+x)5log3xCh. 3.5 - Differentiate. g(x)=x3x(log5x)Ch. 3.5 - Double declining balance depreciation. An office...Ch. 3.5 - Recycling aluminum cans. It is known that 45% of...Ch. 3.5 - 39. Recycling glass. In 2012, 34.1% of all glass...Ch. 3.5 - Household liability. The total financial...Ch. 3.5 - Small Business. The number of nonfarm...Ch. 3.5 - Annuities. Yukiko opens a savings account to pay...Ch. 3.5 - 43. Annuities. Nasim opens a retirement savings...Ch. 3.5 - Prob. 44ECh. 3.5 - The magnitude R (measured on the Richter scale) of...Ch. 3.5 - The magnitude R (measured on the Richter scale) of...Ch. 3.5 - If two earthquakes have magnitudes R1 and R2,...Ch. 3.5 - Prob. 48ECh. 3.5 - Prob. 49ECh. 3.5 - Prob. 50ECh. 3.5 - Prob. 51ECh. 3.5 - Prob. 52ECh. 3.5 - Prob. 53ECh. 3.5 - Finding Natural Logarithms as Limits. Given that...Ch. 3.5 - Finding Natural Logarithms as Limits. Given that...Ch. 3.5 - Finding Natural Logarithms as Limits. Given that...Ch. 3.5 - Finding Natural Logarithms as Limits.
Given that...Ch. 3.5 - Use the Chain Rule, implicit differentiation, and...Ch. 3.5 - Use the Chain Rule, implicit differentiation, and...Ch. 3.5 - Prob. 60ECh. 3.5 - Prob. 61ECh. 3.5 - Prob. 62ECh. 3.5 - Prob. 63ECh. 3.5 - Use the Chain Rule, implicit differentiation, and...Ch. 3.5 - Use the Chain Rule, implicit differentiation, and...Ch. 3.5 - 66. Consider the function, with.
a. Find. (Hint:...Ch. 3.5 - Prob. 67ECh. 3.5 - Prob. 68ECh. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - In Exercises 1-10, find the payment amount p...Ch. 3.6 - Car loans. Todd purchase a new Honda Accord LX for...Ch. 3.6 - Car loans. Katie purchases a new Jeep Wrangler...Ch. 3.6 - 13. Home mortgages. The Hogansons purchase a new...Ch. 3.6 - Mortgages. Andre purchases an office building for...Ch. 3.6 - 15. Credit cards. Joanna uses her credit card to...Ch. 3.6 - 16. Credit cards. Isaac uses his credit card to...Ch. 3.6 - In Exercises 17-22, complete the first two lines...Ch. 3.6 - Prob. 18ECh. 3.6 - In Exercises 17-22, complete the first two lines...Ch. 3.6 - In Exercises 17-22, complete the first two lines...Ch. 3.6 - In Exercises 17-22, complete the first two lines...Ch. 3.6 - In Exercises 17-22, complete the first two lines...Ch. 3.6 - Prob. 23ECh. 3.6 - Maximum loan amount. Curtis plans to purchase a...Ch. 3.6 - 25. Maximum loan amount. The Daleys plan to...Ch. 3.6 - Prob. 26ECh. 3.6 - Prob. 27ECh. 3.6 - 28. Comparing loan options. The Aubrys plan to...Ch. 3.6 - 29. Comparing Rates. Darnell plans to finance...Ch. 3.6 - Prob. 30ECh. 3.6 - Prob. 31ECh. 3.6 - Retirement Planning. Kenna is 30 years old. She...Ch. 3.6 - Prob. 33ECh. 3.6 - 34. Structured settlement. Suppose you won a...Ch. 3.6 - Amortization gives the borrower an advantage: by...Ch. 3.6 - Prob. 36ECh. 3.6 - Prob. 37ECh. 3.6 - a. 3944. Use a spreadsheet to complete the first...Ch. 3.6 - a. 3944. Use a spreadsheet to complete the first...Ch. 3.6 - Prob. 41ECh. 3.6 - Prob. 42ECh. 3.6 - a. 39–44. Use a spreadsheet to complete the first...Ch. 3.6 - Prob. 44ECh. 3 - In Exercises 1-6, match each equation in column A...Ch. 3 - In Exercises 1-6, match each equation in column A...Ch. 3 - In Exercises 1-6, match each equation in column A...Ch. 3 - In Exercises 1-6, match each equation in column A...Ch. 3 - In Exercises 1-6, match each equation in column A...Ch. 3 - Prob. 6RECh. 3 - Classify each statement as either true or...Ch. 3 - Prob. 8RECh. 3 - Prob. 9RECh. 3 - Classify each statement as either true or...Ch. 3 - Classify each statement as either true or false. A...Ch. 3 - Classify each statement as either true or false. A...Ch. 3 - Classify each statement as either true or false....Ch. 3 - Classify each statement as either true or...Ch. 3 - Classify each statement as either true or...Ch. 3 - 16. Find
a.
b.
c.
Ch. 3 - Differentiate each function. y=lnxCh. 3 - Differentiate each function.
18.
Ch. 3 - Differentiate each function.
19.
Ch. 3 - Differentiate each function. y=e2xCh. 3 - Differentiate each function. f(x)=lnxCh. 3 - Differentiate each function. f(x)=x4e3xCh. 3 - Differentiate each function. f(x)=lnxx3Ch. 3 - Differentiate each function.
24.
Ch. 3 - Differentiate each function.
25.
Ch. 3 - Prob. 26RECh. 3 - Differentiate each function. F(x)=9xCh. 3 - Prob. 28RECh. 3 - Differentiate each function.
29.
Ch. 3 - Graph each function. f(x)=4xCh. 3 - Graph each function.
31.
Ch. 3 - Given and, find each logarithm.
32.
Ch. 3 - Given and, find each logarithm.
33.
Ch. 3 - Prob. 34RECh. 3 - Given loga2=1.8301 and loga7=5.0999, find each...Ch. 3 - Given and, find each logarithm.
36.
Ch. 3 - Given and, find each logarithm.
37.
Ch. 3 - Find the function Q that satisfies dQ/dt=7Q, given...Ch. 3 - Prob. 39RECh. 3 - Business: Interest compounded continuously....Ch. 3 - Prob. 41RECh. 3 - 42. Business: Cost of Oreo Cookies. The average...Ch. 3 - 43. Business: Franchise Growth. Fashionista...Ch. 3 - Prob. 44RECh. 3 - Life Science: Decay Rate. The decay rate of a...Ch. 3 - Prob. 46RECh. 3 - Life Science: Decay Rate. A certain radioactive...Ch. 3 - Prob. 48RECh. 3 - 49. Business: Present Value. Find the present...Ch. 3 - Business: Annuity. Patrice deposits $50 into a...Ch. 3 - Business: Car Loan. Glenda buys a used Subaru...Ch. 3 - Prob. 52RECh. 3 - Business: Credit Card. Vicki uses her credit card...Ch. 3 - 54. Differentiate: .
Ch. 3 -
55. Find the minimum value of.
Ch. 3 - Prob. 56RECh. 3 - Prob. 57RECh. 3 - Business: shopping on the internet. Online sales...Ch. 3 - Differentiate. y=2e3xCh. 3 - Differentiate. y=(lnx)4Ch. 3 - Differentiate.
3.
Ch. 3 - Differentiate. f(x)=lnx7Ch. 3 - Differentiate.
5.
Ch. 3 - Differentiate. f(x)=3exlnxCh. 3 - Differentiate.
7.
Ch. 3 - Prob. 8TCh. 3 - Prob. 9TCh. 3 - Prob. 10TCh. 3 - Given logb2=0.2560 and logb9=0.8114, find each of...Ch. 3 - Given logb2=0.2560 and logb9=0.8114, find each of...Ch. 3 - 13. Find the function that satisfies, if at.
Ch. 3 - 14. The doubling time for a certain bacteria...Ch. 3 - 15. Business: interest compounded continuously. An...Ch. 3 - Business: Cost of Milk. The cost C of a gallon of...Ch. 3 - 17. Life science: drug dosage. A dose of a drug is...Ch. 3 - 18. Life Science: decay rate. The decay rate of...Ch. 3 - 19. Life science: half-rate. The half-life of...Ch. 3 - Business: effect of advertising. Twin City...Ch. 3 - Prob. 21TCh. 3 - 22. Business: Amortized Loan. The Langways...Ch. 3 - 23. Business: Car Loan. Giselle qualifies for a...Ch. 3 - Differentiate: y=x(lnx)22xlnx+2x.Ch. 3 - Find the maximum and minimum values of f(x)=x4ex...Ch. 3 - Prob. 26TCh. 3 - Prob. 27TCh. 3 - Prob. 1ETECh. 3 - Use the exponential function to predict gross...Ch. 3 - Prob. 3ETECh. 3 - Prob. 5ETECh. 3 - Prob. 7ETECh. 3 - Prob. 8ETE
Additional Math Textbook Solutions
Find more solutions based on key concepts
In hypothesis testing, the common level of significance is =0.05. Some might argue for a level of significance ...
Basic Business Statistics, Student Value Edition
Fill in each blank so that the resulting statement is true. An equation that expresses a relationship between t...
Algebra and Trigonometry (6th Edition)
Sampling Method. In Exercises 9-12, determine whether the sampling method appears to be sound or is flawed.
9. ...
Elementary Statistics
In Exercises 25–28, use the confidence interval to find the margin of error and the sample mean.
25. (12.0, 14....
Elementary Statistics: Picturing the World (7th Edition)
Find the additive inverse of each of the following integers. Write the answer in the simplest possible form. a....
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
CHECK POINT 1 Find a counterexample to show that the statement The product of two two-digit numbers is a three-...
Thinking Mathematically (6th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- sy = f(x) + + + + + + + + + X 3 4 5 7 8 9 The function of shown in the figure is continuous on the closed interval [0, 9] and differentiable on the open interval (0, 9). Which of the following points satisfies conclusions of both the Intermediate Value Theorem and the Mean Value Theorem for f on the closed interval [0, 9] ? (A A B B C Darrow_forward= Q6 What will be the allowable bearing capacity of sand having p = 37° and ydry 19 kN/m³ for (i) 1.5 m strip foundation (ii) 1.5 m x 1.5 m square footing and (iii)1.5m x 2m rectangular footing. The footings are placed at a depth of 1.5 m below ground level. Assume F, = 2.5. Use Terzaghi's equations. 0 Ne Na Ny 35 57.8 41.4 42.4 40 95.7 81.3 100.4arrow_forwardQ1 The SPT records versus depth are given in table below. Find qan for the raft 12% foundation with BxB-10x10m and depth of raft D-2m, the allowable settlement is 50mm. Elevation, m 0.5 2 2 6.5 9.5 13 18 25 No.of blows, N 11 15 29 32 30 44 0 estigate shear 12%arrow_forward
- Q2 A/ State the main field tests which may be carried out to investigate shear strength of a soil layer? B/ What are the main factors that affecting the spacing and number of boreholes for a given project? C/ Illustrate the causes of disturbance of Shelby tubes samples.arrow_forwarddw z = Find using direct dt If w = + x = (cost), y = (sint), z= substitution and chain rule methods.arrow_forwardSolve thisarrow_forward
- What are the correct answers for the second and third question on this page. I am on the Cartesian vectors unit in calculuarrow_forwardTrolley of the overhead crane moves along the bridge rail. The trolley position is measured from the center of the bridge rail (x = 0) is given by x(t) = 0.5t^3-6t^2+19.5t-14 : 0 <= t <= 3 min. The trolley moves from point A to B in the forward direction, B to C in the reverse direction and C to D again in the forward direction. CONTROL PANEL END TRUCK- RUNWAY BEAM- BRIDGE RAIL HOIST -TROLLEY TROLLEY BUMPER TROLLEY DRIVE LPENDANT TRACK -TROLLEY CONDUCTOR TRACK WIRE ROPE -HOOK BLOCK -BRIDGE DRIVE -END TRUCK BUMPER -RUNWAY RAIL TROLLEY END STOP -CONDUCTOR BAR PENDANT FESTOONING TROLLEY FESTOONING PENDANT CABLE PENDANT x(t)=0.5t^3-6t^2+19.5t-14 v(t)=1.5t^2-12t+19.5 a(t)=(dv(t))/dt=3t-12 Fig. T2.2: The overhead crane Total masses of the trolley, hook block, and the load attached to the hook block are 110 kg, 20 kg, and 150 kg. Damping coefficient, D, is 40 kg/s. What is the total amount of energy required from the trolley motor to move the system [Hint: Use Newton's 2nd law to obtain the…arrow_forwardCONTROL PANEL- BRIDGE RAIL HOIST -TROLLEY TROLLEY BUMPER -BRIDGE DRIVE END TRUCK- RUNWAY BEAM- END TRUCK BUMPER -RUNWAY RAIL TROLLEY DRIVE TROLLEY END STOP -CONDUCTOR BAR LPENDANT TRACK TROLLEY CONDUCTOR TRACK -WIRE ROPE PENDANT FESTOONING TROLLEY FESTOONING -PENDANT CABLE -HOOK BLOCK PENDANTarrow_forward
- chool Which of the following functions describes the graph of g(x)--2√9-x²+37 9 8 7 6 4 2 -10-9-8-7-6-5-4-3-2-1 1 -1 -2 -4 -6 10 9 8 B 5 4 3 3 6 -10-9-8-7-6-5-4-3-2-1 2 3 4 6 1 -2 4 -5 -6 -8 -9 -10 10 -10-9-8-7-6-5-4-3-2-1 9 8 Lessons Assessments 6 5 4 + 2 1 1 2 3 4 5 6 8 -1 2 4 -5 -B 8 10 10 9 8 7 6 5 4 3 2 1 -10-9-8-7-6-5-4-3-2-1 1 2 3 4 5 6 B 9 10 -1 -2 -3 -5arrow_forwardPlease sketch questions 1, 2 and 6arrow_forwardsolve questions 3, 4,5, 7, 8, and 9arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Elementary AlgebraAlgebraISBN:9780998625713Author:Lynn Marecek, MaryAnne Anthony-SmithPublisher:OpenStax - Rice University
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Elementary Algebra
Algebra
ISBN:9780998625713
Author:Lynn Marecek, MaryAnne Anthony-Smith
Publisher:OpenStax - Rice University
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Area Between The Curve Problem No 1 - Applications Of Definite Integration - Diploma Maths II; Author: Ekeeda;https://www.youtube.com/watch?v=q3ZU0GnGaxA;License: Standard YouTube License, CC-BY