
Concept explainers
The contribution to the circulation integral due to each segment of the loop, and the net current through the loop that must be present.
Answer to Problem 17PQ
The contribution of side 1 is 0.0313 T⋅m , the contribution of side 2 is −0.0438 T⋅m , the contribution of side 3 is −0.0313 T⋅m and the contribution of side 4 is 0.0438 T⋅m.
Explanation of Solution
Draw the figure for the system as shown below:
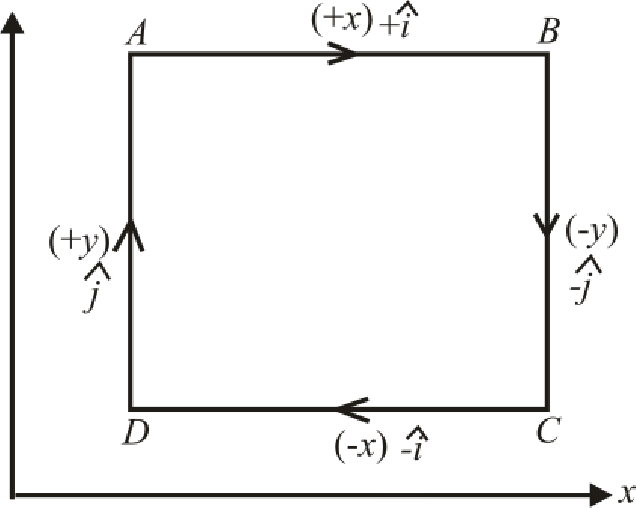
Refer to the above figure, consider a square loop ABCD in xy-plane. The sides AB and CD have equal magnitude in opposite direction whereas side BC and side DA has equal magnitude in opposite direction.
Write the general expression for Ampere’s Circuital Law for the given above figure as.
μ0Ithru=∮→B.→dl (I)
Here, →dl is length of element, μ0 is the permittivity of free space, Ithru is the current through the conductor and →B is magnetic field.
Substitute dxˆi for →dl in equation (I) for side AB as.
(μ0Ithru)AB=B∫A→B.dxˆi (II)
Substitute dy(−ˆj) for →dl in equation (I) for side BC as.
(μ0Ithru)BC=C∫B→B.(−dy)ˆj (III)
Substitute dx(−ˆi) for →dl in equation (I) for side CD as.
(μ0Ithru)CD=D∫C→B.(−dx)ˆi (IV)
Substitute dy(ˆj) for →dl in equation (I) for side DA as.
(μ0Ithru)DA=A∫D→B.dyˆj (V)
Conclusion:
Substitute (1.25ˆi+1.75ˆj)T for →B and 2.50 cmˆi for ∫dxˆi in equation (II).
(μ0Ithru)AB=((1.25ˆi+1.75ˆj)T)⋅(2.50 cm)ˆi=((1.25ˆi+1.75ˆj)T)⋅(2.50 cm(1 m100 cm))ˆi=((1.25ˆi+1.75ˆj)T)⋅(0.025 m)ˆi=0.03125 T⋅m
Further,simplify the above equation as.
(μ0Ithru)AB=0.0313 T⋅m
Thus, contribution of side AB is 0.0313 T⋅m_.
Substitute (1.25ˆi+1.75ˆj)T for →B and 2.50 cm(−ˆj) for ∫dy(−ˆj) in equation (III).
(μ0Ithru)BC=((1.25ˆi+1.75ˆj)T)⋅(2.50 cm)(−ˆj)=((1.25ˆi+1.75ˆj)T)⋅(2.50 cm(1 m100 cm))(−ˆj)=((1.25ˆi+1.75ˆj)T)⋅(0.025 m)(−ˆj)=−0.0438 T⋅m
Further,simplify the above equation as.
(μ0Ithru)BC=−0.0438 T⋅m
Thus, contribution of side BC is −0.0438 T⋅m_.
Substitute (1.25ˆi+1.75ˆj) T for →B and 2.50 cm(−ˆi) for ∫dx(−ˆi) in equation (IV).
(μ0Ithru)CD=((1.25ˆi+1.75ˆj) T)⋅(2.50 cm)(−ˆi)=((1.25ˆi+1.75ˆj) T)⋅(2.50 cm×1 m100 cm)(−ˆi)=((1.25ˆi+1.75ˆj) T)⋅(0.025 m)(−ˆi)=−0.03125 T⋅m
Further,simplify the above equation as.
(μ0Ithru)CD=−0.0313 T⋅m
Thus, the contribution of side CD is −0.0313 T⋅m_.
Substitute (1.25ˆi+1.75ˆj) T for →B and (2.50 cm)ˆj for ∫dyˆj in equation (V).
(μ0Ithru)DA=((1.25ˆi+1.75ˆj) T)⋅(2.50 cm)ˆj=((1.25ˆi+1.75ˆj) T)⋅(2.50 cm(1 m100 cm))ˆj=((1.25ˆi+1.75ˆj) T)⋅(0.025 m)ˆj=0.0438 T⋅m
Further,simplify the above equation as.
(μ0Ithru)DA=0.0438 T
Thus, the contribution of side AB is 0.0438 T_.
Thus, the contribution of side 1 is 0.0313 T⋅m , the contribution of side 2 is −0.0438 T⋅m , the contribution of side 3 is −0.0313 T⋅m and the contribution of side 4 is 0.0438 T⋅m.
Want to see more full solutions like this?
Chapter 31 Solutions
Physics for Scientists and Engineers: Foundations and Connections
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning





