Chapter 3, Problem 83PP

BIO Motion Camouflage in Dragonflies

Dragonflies, whose ancestors were once the size of hawks, have prowled the skies in search of small flying insects for over 250 million years. Faster and more maneuverable than any other insect, they even fold their front two legs in flight and tuck them behind their head to be as streamlined as possible. They also employ an intriguing stalking strategy known as “motion camouflage” to approach their prey almost undetected.

The basic idea of motion camouflage is for the dragonfly to move in such a way that the line of sight from the prey to the dragonfly is always in the same direction. Moving in this way, the dragonfly appears almost motionless to its prey, as if it were an object at infinity. Eventually the prey notices the dragonfly has grown in size and is therefore closer but by that time it’s too late for the prey to evade capture.

A typical capture scenario is shown in Figure 3-51, where the prey moves in the positive y direction with the constant speed u_p = 0.750 m/s, and the dragonfly moves at an angle θ = 48.5° to the x axis with the constant speed v_d. If the dragonfly chooses its speed correctly, the line of sight from the prey to the dragonfly will always be in the same direction—parallel to the x axis in this case.

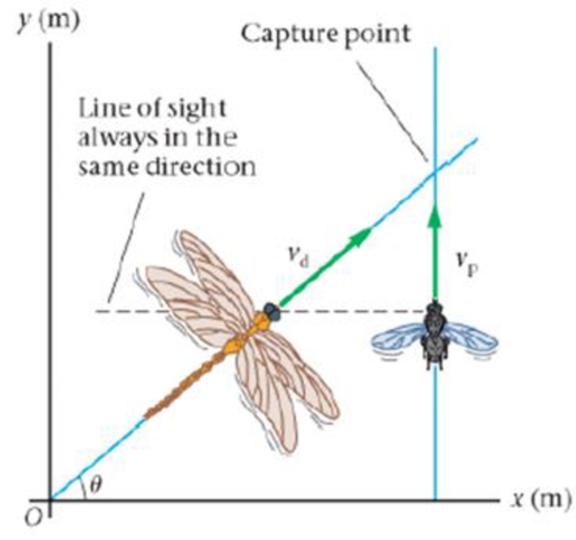
Figure 3-51 Problems 83, 84, 85, and 86

83. • What speed must the dragonfly have if the line of sight, which is parallel to the x axis initially is to remain parallel to the x axis?

1. A.    0.562 m/s
2. B.     0.664 m/s
3. C.     1.00m/s
4. D.    1.13 m/s