
The following nonlinear, parasitic ODE was suggested by Hornbeck (1975):
If the initial condition is
(a) Analytically
(b) Using the fourth-order RK method with a constant step size of 0.03125.
(c) Using the MATLAB function ode45.
(d) Using the MATLAB function ode23s.
(e) Using the MATLAB function ode23tb.
Present your results in graphical form.
(a)
To calculate: The analytical solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The analytical solution of the differential equation is
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Formula used:
The general linear differential equation is,
Calculation:
Consider the nonlinear ordinary differential equation,
Rearrange the above differential equationto get,
Now, compare the above differential equation with the general linear differential equation
Thus,
Now, find the integrating factor (I. F.) as shown below,
Therefore, the solution of the linear differential equation is given as,
Substitute the value of integrating factor (I. F.) in above equation,
Now, integrate the right-hand-side of the above equation,
Solve further, to get
Thus, the solution is
Now, to determine the constant c, use the initial condition
Substitute
Therefore,
Hence, the solution of the differential equation is
(b)
To calculate: Thesolution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Calculation:
Consider the nonlinear ordinary differential equation,
The VBA code to solve the above differential equation with fourth-order RK method with a constant step size of 0.03125 is given below,
The output given below is obtained in the Excel after the execution of the above code:
| 4th order RK | ||
| t | y1 | |
| 0 | 0.08 | 0.08 |
| 0.03125 | 0.093476 | 0.093477 |
| 0.0625 | 0.108906 | 0.108906 |
| 0.09375 | 0.126289 | 0.126289 |
| 0.125 | 0.145625 | 0.145625 |
| 0.15625 | 0.166914 | 0.166914 |
| 0.1875 | 0.190156 | 0.190156 |
| 0.21875 | 0.215351 | 0.215352 |
| 0.25 | 0.242499 | 0.2425 |
| 0.28125 | 0.2716 | 0.271602 |
| 0.3125 | 0.302655 | 0.302656 |
| 0.34375 | 0.335662 | 0.335664 |
| 0.375 | 0.370622 | 0.370625 |
| 0.40625 | 0.407536 | 0.407539 |
| 0.4375 | 0.446403 | 0.446406 |
| 0.46875 | 0.487222 | 0.487227 |
| 0.5 | 0.529995 | 0.53 |
| 0.53125 | 0.57472 | 0.574727 |
| 0.5625 | 0.621399 | 0.621406 |
| 0.59375 | 0.670031 | 0.670039 |
| 0.625 | 0.720615 | 0.720625 |
| 0.65625 | 0.773152 | 0.773164 |
| 0.6875 | 0.827642 | 0.827656 |
| 0.71875 | 0.884085 | 0.884102 |
| 0.75 | 0.942481 | 0.9425 |
| 0.78125 | 1.002829 | 1.002852 |
| 0.8125 | 1.06513 | 1.065156 |
| 0.84375 | 1.129383 | 1.129414 |
| 0.875 | 1.195589 | 1.195625 |
| 0.90625 | 1.263747 | 1.263789 |
| 0.9375 | 1.333857 | 1.333906 |
| 0.96875 | 1.405919 | 1.405977 |
| 1 | 1.479932 | 1.48 |
| 1.03125 | 1.555897 | 1.555977 |
| 1.0625 | 1.633814 | 1.633906 |
| 1.09375 | 1.713681 | 1.713789 |
| 1.125 | 1.795498 | 1.795625 |
| 1.15625 | 1.879266 | 1.879414 |
| 1.1875 | 1.964983 | 1.965156 |
| 1.21875 | 2.052649 | 2.052852 |
| 1.25 | 2.142263 | 2.1425 |
| 1.28125 | 2.233824 | 2.234102 |
| 1.3125 | 2.327332 | 2.327656 |
| 1.34375 | 2.422785 | 2.423164 |
| 1.375 | 2.520181 | 2.520625 |
| 1.40625 | 2.61952 | 2.620039 |
| 1.4375 | 2.7208 | 2.721406 |
| 1.46875 | 2.824017 | 2.824727 |
| 1.5 | 2.929171 | 2.93 |
| 1.53125 | 3.036257 | 3.037227 |
| 1.5625 | 3.145273 | 3.146406 |
| 1.59375 | 3.256214 | 3.257539 |
| 1.625 | 3.369075 | 3.370625 |
| 1.65625 | 3.483852 | 3.485664 |
| 1.6875 | 3.600538 | 3.602656 |
| 1.71875 | 3.719125 | 3.721602 |
| 1.75 | 3.839605 | 3.8425 |
| 1.78125 | 3.961966 | 3.965352 |
| 1.8125 | 4.086198 | 4.090156 |
| 1.84375 | 4.212287 | 4.216914 |
| 1.875 | 4.340215 | 4.345625 |
| 1.90625 | 4.469964 | 4.476289 |
| 1.9375 | 4.601512 | 4.608906 |
| 1.96875 | 4.734831 | 4.743477 |
| 2 | 4.869893 | 4.88 |
| 2.03125 | 5.00666 | 5.018477 |
| 2.0625 | 5.145091 | 5.158906 |
| 2.09375 | 5.285137 | 5.301289 |
| 2.125 | 5.426742 | 5.445625 |
| 2.15625 | 5.569837 | 5.591914 |
| 2.1875 | 5.714346 | 5.740156 |
| 2.21875 | 5.860176 | 5.890352 |
| 2.25 | 6.007221 | 6.0425 |
| 2.28125 | 6.155356 | 6.196602 |
| 2.3125 | 6.304436 | 6.352656 |
| 2.34375 | 6.454288 | 6.510664 |
| 2.375 | 6.604715 | 6.670625 |
| 2.40625 | 6.755482 | 6.832539 |
| 2.4375 | 6.906318 | 6.996406 |
| 2.46875 | 7.056903 | 7.162227 |
| 2.5 | 7.206864 | 7.33 |
| 2.53125 | 7.355766 | 7.499727 |
| 2.5625 | 7.503099 | 7.671406 |
| 2.59375 | 7.648268 | 7.845039 |
| 2.625 | 7.790577 | 8.020625 |
| 2.65625 | 7.92921 | 8.198164 |
| 2.6875 | 8.063218 | 8.377656 |
| 2.71875 | 8.191486 | 8.559102 |
| 2.75 | 8.312714 | 8.7425 |
| 2.78125 | 8.425381 | 8.927852 |
| 2.8125 | 8.527709 | 9.115156 |
| 2.84375 | 8.617619 | 9.304414 |
| 2.875 | 8.69268 | 9.495625 |
| 2.90625 | 8.750052 | 9.688789 |
| 2.9375 | 8.786412 | 9.883906 |
| 2.96875 | 8.797877 | 10.08098 |
| 3 | 8.779905 | 10.28 |
| 3.03125 | 8.727189 | 10.48098 |
| 3.0625 | 8.633523 | 10.68391 |
| 3.09375 | 8.491649 | 10.88879 |
| 3.125 | 8.293087 | 11.09563 |
| 3.15625 | 8.027917 | 11.30441 |
| 3.1875 | 7.684546 | 11.51516 |
| 3.21875 | 7.249417 | 11.72785 |
| 3.25 | 6.706683 | 11.9425 |
| 3.28125 | 6.037815 | 12.1591 |
| 3.3125 | 5.221152 | 12.37766 |
| 3.34375 | 4.231369 | 12.59816 |
| 3.375 | 3.038857 | 12.82063 |
| 3.40625 | 1.609002 | 13.04504 |
| 3.4375 | -0.09867 | 13.27141 |
| 3.46875 | -2.13146 | 13.49973 |
| 3.5 | -4.5447 | 13.73 |
| 3.53125 | -7.40305 | 13.96223 |
| 3.5625 | -10.7821 | 14.19641 |
| 3.59375 | -14.7703 | 14.43254 |
| 3.625 | -19.4709 | 14.67063 |
| 3.65625 | -25.0048 | 14.91066 |
| 3.6875 | -31.5132 | 15.15266 |
| 3.71875 | -39.1613 | 15.3966 |
| 3.75 | -48.1421 | 15.6425 |
| 3.78125 | -58.6814 | 15.89035 |
| 3.8125 | -71.043 | 16.14016 |
| 3.84375 | -85.5354 | 16.39191 |
| 3.875 | -102.519 | 16.64563 |
| 3.90625 | -122.417 | 16.90129 |
| 3.9375 | -145.72 | 17.15891 |
| 3.96875 | -173.006 | 17.41848 |
| 4 | -204.949 | 17.68 |
| 4.03125 | -242.336 | 17.94348 |
| 4.0625 | -286.088 | 18.20891 |
| 4.09375 | -337.283 | 18.47629 |
| 4.125 | -397.179 | 18.74563 |
| 4.15625 | -467.248 | 19.01691 |
| 4.1875 | -549.211 | 19.29016 |
| 4.21875 | -645.079 | 19.56535 |
| 4.25 | -757.205 | 19.8425 |
| 4.28125 | -888.338 | 20.1216 |
| 4.3125 | -1041.69 | 20.40266 |
| 4.34375 | -1221.03 | 20.68566 |
| 4.375 | -1430.74 | 20.97063 |
| 4.40625 | -1675.96 | 21.25754 |
| 4.4375 | -1962.71 | 21.54641 |
| 4.46875 | -2297.99 | 21.83723 |
| 4.5 | -2690.02 | 22.13 |
| 4.53125 | -3148.39 | 22.42473 |
| 4.5625 | -3684.34 | 22.72141 |
| 4.59375 | -4310.97 | 23.02004 |
| 4.625 | -5043.62 | 23.32063 |
| 4.65625 | -5900.23 | 23.62316 |
| 4.6875 | -6901.75 | 23.92766 |
| 4.71875 | -8072.7 | 24.2341 |
| 4.75 | -9441.73 | 24.5425 |
| 4.78125 | -11042.3 | 24.85285 |
| 4.8125 | -12913.7 | 25.16516 |
| 4.84375 | -15101.5 | 25.47941 |
| 4.875 | -17659.5 | 25.79563 |
| 4.90625 | -20650.1 | 26.11379 |
| 4.9375 | -24146.4 | 26.43391 |
| 4.96875 | -28234.2 | 26.75598 |
Now, plot the following chart using the data obtained in Excel.
In the above plot, the series1 represent the numerical solution whereas the series2 represent the exact solution.
(c)
The solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
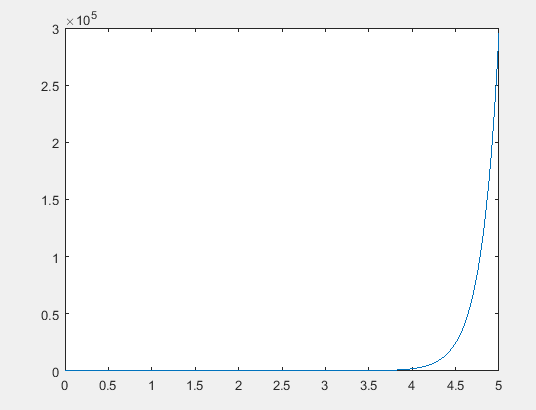
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Consider the nonlinear ordinary differential equation,
Use the MATLAB function
Write the code given below in MATLAB editor window and save it.
function
Now, write the following code in MATLAB command window

Following graph is obtained after the execution of the above MATLAB code.

(d)
The solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
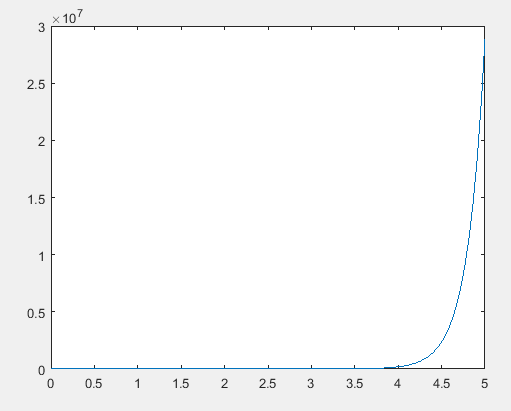
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Consider the nonlinear ordinary differential equation,
Use the MATLAB function
Write the code given below in MATLAB editor window and save it.
function
Now, write the following code in MATLAB command window

Following graph is obtained after the execution of the above MATLAB code.

(e)
The solution of the nonlinear, parasitic ordinary differential equation,
Answer to Problem 27P
Solution:
The graph of the solution of the differential equation is,
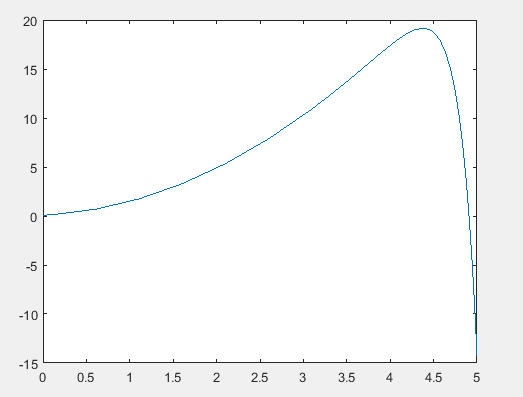
Explanation of Solution
Given Information:
A nonlinear, parasitic ordinary differential equation,
Consider the nonlinear ordinary differential equation,
Use the MATLAB function
Write the code given below in MATLAB editor window and save it.
function
Now, write the following code in MATLAB command window

Following graph is obtained after the execution of the above MATLAB code.

Want to see more full solutions like this?
Chapter 27 Solutions
Numerical Methods for Engineers
Additional Math Textbook Solutions
Introductory Statistics
Calculus: Early Transcendentals (2nd Edition)
Precalculus: A Unit Circle Approach (3rd Edition)
A First Course in Probability (10th Edition)
Precalculus: Mathematics for Calculus (Standalone Book)
Elementary Statistics ( 3rd International Edition ) Isbn:9781260092561
- An Inclining experiment done on a ship thats 6500 t, a mass of 30t was moved 6.0 m transvesly causing a 30 cm deflection in a 6m pendulum, calculate the transverse meta centre height.arrow_forwarda ship 150 m long and 20.5 m beam floats at a draught of8 m and displaces 19 500 tonne. The TPC is 26.5 and midshipsection area coefficient 0.94. Calculate the block, prismatic andwaterplane area coefficients.arrow_forwardA vessel loads 680 t fuel between forward and aft deep tanks. centre of gravity of forward tank is 24m forward of ships COG. centre to centre between tanks is 42 m. how much in each tank to keep trim the samearrow_forward
- Beam of a vessel is 11% its length. Cw =0.72. When floating in SW of relative denisity 1.03, TPC is 0.35t greater than in freshwater. Find the length of the shiparrow_forwardAn inclining experiment was carried out on a ship of 4000tonne displacement, when masses of 6 tonne were moved transverselythrough 13.5 m. The deflections of a 7.5 m pendulurnwere 81, 78, 85, 83, 79, 82, 84 and 80 mm respectively.Caiculate the metacentric height.arrow_forwardA ship of 10 000 tonne displacement has a waterplanearea of 1300 m2. The ship loads in water of 1.010 t/m3 andmoves into water of 1.026 t/m3. Find the change in meandraughtarrow_forward
- A ship of 7000 tonne displacement has a waterplane areaof 1500 m2. In passing from sea water into river water of1005 kg/m3 there is an increase in draught of 10 cm. Find the Idensity of the sea water.arrow_forwardA ship has 300 tonne of cargo in the hold, 24 m forward ofmidships. The displacement of the vessel is 6000 tonne and its centre of gravity is 1.2 m forward of midships.Find the new position of the centre of gravity if this cargo ismoved to an after hold, 40 m from midshipsarrow_forwardSketch and describe how ships are supported in dry dock. When and where does the greatest amount of stresses occur?arrow_forward
- Sketch and desribe a balanced rudder and how it is suspendedarrow_forwardA ship 140 m long and 18 m beam floats at a draught of9 m. The immersed cross-sectionai areas at equai intervais are 5,60, 116, 145, 152, 153, 153, 151, 142, 85 and 0 m2 respectively.Calculate:(a) displacement(b) block coefficient(c) midship section area coefficient(d) prismatic coefficient.arrow_forwardA steamer has waterplane area 1680m2 recorded in water with relative denisty 1.013. Displacement = 1200 t, calculate difference in draught in salwater reltive denisity 1.025.arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





