Chapter 2.1, Problem 21P

Expand Your knowledge: Decimal Data The fallowing data represent tonnes of wheat harvested each year (1894-1925) from Plot 19 at the Rothamsted Agricultural Experiment Stations, England.

2.71	1.62	2.60	1.64	2.20	2.02	1.67	1.99	2.34	1.26	1.31
1.80	2.82	2.15	2.07	1.62	1.47	2.19	0.59	1.48	0.77	1.04
1.32	0.89	1.35	0.95	0.94	1.39	1.19	1.18	0.46	0.70

(a) Multiply each data value by 100 to “clear" the decimals.

(b) Use the standard procedures of this section to make a frequency table and histogram with your whole-number data. Use six classes.

(c) Divide class limits, class boundaries, and class midpoints by 100 to get back to your original data values.

To determine

To find: The data that are multiply with 100 for each value in the data..

Answer to Problem 21P

Solution: The data multiply with 100 for each value in the data is as follows:

data	data*100	data	data*100	data	data*100	data	data*100
2.71	271	2.34	234	1.47	147	1.35	135
1.62	162	1.26	126	2.19	219	0.95	95
2.6	260	1.31	131	0.59	59	0.94	94
1.64	164	1.8	180	1.48	148	1.39	139
2.2	220	2.82	282	0.77	77	1.19	119
2.02	202	2.15	215	2.04	204	1.18	118
1.67	167	2.07	207	1.32	132	0.46	46
1.99	199	1.62	162	0.89	89	0.7	70

Explanation of Solution

Calculation: The data represent the tons of wheat harvested each year and there are 32 values in the data set. To find the decimal data in to “clear” data that multiply with each value in the data by 100. The calculation is as follows:

Data	Data*100	Data	Data*100	Data	Data*100	Data	Data*100
2.71	$2.71\times 100=271$	2.34	234	1.47	147	1.35	135
1.62	$1.62\times 100=162$	1.26	126	2.19	219	0.95	95
2.6	$2.6\times 100=260$	1.31	131	0.59	59	0.94	94
1.64	164	1.8	180	1.48	148	1.39	139
2.2	220	2.82	282	0.77	77	1.19	119
2.02	202	2.15	215	2.04	204	1.18	118
1.67	167	2.07	207	1.32	132	0.46	46
1.99	199	1.62	162	0.89	89	0.7	70

Interpretation: Hence, the data multiplied with 100 is as follows:

Data	Data*100	Data	Data*100	Data	Data*100	Data	Data*100
2.71	271	2.34	234	1.47	147	1.35	135
1.62	162	1.26	126	2.19	219	0.95	95
2.6	260	1.31	131	0.59	59	0.94	94
1.64	164	1.8	180	1.48	148	1.39	139
2.2	220	2.82	282	0.77	77	1.19	119
2.02	202	2.15	215	2.04	204	1.18	118
1.67	167	2.07	207	1.32	132	0.46	46
1.99	199	1.62	162	0.89	89	0.7	70

To determine

To find: The class width, class limits, class boundaries, midpoint, frequency, relative frequency, and cumulative frequency of the data..

Answer to Problem 21P

Solution: The complete frequency table is as follows:

class limits	class boundaries	midpoints	freq	relative freq	cumulative freq
46-85	45.5-85.5	65.5	4	0.12	4
86-125	85.5-125.5	105.5	5	0.16	9
126-165	125.5-165.5	145.5	10	0.31	19
166-205	165.5-205.5	185.5	5	0.16	24
206-245	205.5-245.5	225.5	5	0.16	29
246-285	245.5-285.5	265.5	3	0.09	32

Explanation of Solution

Calculation: To find the class width for the whole data of 32 values, it is observed that largest value of the data set is 282 and the smallest value is 46 in the data. Using 6 classes, the class width calculated in the following way:

$\begin{array}{l}Classwidth=\frac{Largestdatavalue-smallestdatavalue}{Desirednumberofclasses}\\ \end{array}$

$\begin{array}{c}Classwidth=\frac{282-46}{6}\\ =39.33\\ \approx 40\end{array}$

The value is round up to the nearest whole number. Hence, the class width of the data set is 40. The class width for the data is 40 and the lowest data value (46) will be the lower class limit of the first class. Because the class width is 40, it must add 40 to the lowest class limit in the first class to find the lowest class limit in the second class. There are 6 desired classes. Hence, the class limits are 46–85, 86–125, 126–165, 166–205, 206–245, and 246–285. Now, to find the class boundaries subtract 0.5 from lower limit of every class and add 0.5 to the upper limit of every class interval. Hence, the class boundaries are 45.5–85.5, 85.5–125.5, 125.5–165.5, 165.5–205.5, 205.5–245.5, and 245.5–285.5.

Next to find the midpoint of the class is calculated by using formula,

$\begin{array}{l}\text{Midpoint=}\frac{\text{Lower class limit + Upper class limit}}{\text{2}}\\ \end{array}$

Midpoint of first class is calculated as:

$\begin{array}{c}\text{Midpoint}=\frac{\text{45}\text{.5+ 85}\text{.5}}{2}\\ =65.5\end{array}$

The frequencies for respective classes are 4, 5, 10, 5, 5, and 3.

Relative frequency is calculated by using the formula

$\text{Relative frequency}=\frac{f}{n}=\frac{\text{frequency}}{\text{Total of all frequencies}}$

The frequency for 1^st class is 4 and total frequencies are 32 so the relative frequency is $\frac{4}{32}=0.13$(Same calculation for other class).

The calculated frequency table is as follows:

Class limits	Class boundaries	Midpoints	Freq	Relative freq	Cumulative freq
46-85	45.5-85.5	65.5	4	0.12	4
86-125	85.5-125.5	105.5	5	0.16	9
126-165	125.5-165.5	145.5	10	0.31	19
166-205	165.5-205.5	185.5	5	0.16	24
206-245	205.5-245.5	225.5	5	0.16	29
246-285	245.5-285.5	265.5	3	0.09	32

Graph: To construct the histogram by using the MINITAB, the steps are as follows:

Step 1: Enter the class boundaries in C1 and frequency in C2.

Step 2: Go to Graph > Histogram > Simple.

Step 3: Enter C1 in Graph variable then go to Data options > Frequency > C2.

Step 4: Click on OK.

The obtained histogram is as follows:

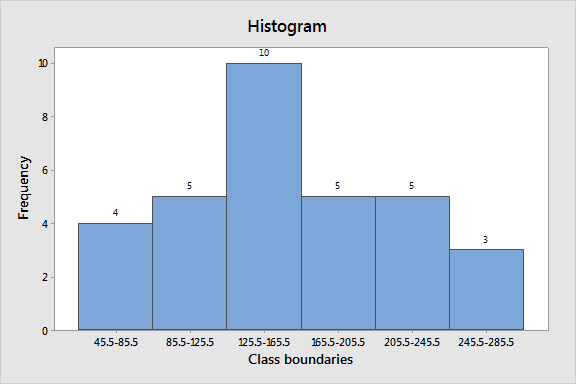
Interpretation: Hence, the complete frequency table is as follows:

Class limits	Class boundaries	Midpoints	Freq	Relative freq	Cumulative freq
46-85	45.5-85.5	65.5	4	0.12	4
86-125	85.5-125.5	105.5	5	0.16	9
126-165	125.5-165.5	145.5	10	0.31	19
166-205	165.5-205.5	185.5	5	0.16	24
206-245	205.5-245.5	225.5	5	0.16	29
246-285	245.5-285.5	265.5	3	0.09	32

To determine

To find: The class limits, class boundaries, and midpoints in the table by dividing 100..

Answer to Problem 21P

Solution: The frequency table of original data is as:

class limits	class boundaries	Midpoints
0.46-0.85	0.455-0.855	0.655
0.86-1.25	0.855-1.255	1.055
1.26-1.65	1.255-1.655	1.455
1.66-2.05	1.655-2.055	1.855
2.06-2.45	2.055-2.455	2.255
2.46-2.85	2.455-2.855	2.655

Explanation of Solution

Calculation: The frequency table for whole number is obtained in above part. It is the data that multiply each value by 100 to ‘clear’ decimals from the data. The frequency table for whole number is as follows:

class limits	class boundaries	Midpoints
46-85	45.5-85.5	65.5
86-125	85.5-125.5	105.5
126-165	125.5-165.5	145.5
166-205	165.5-205.5	185.5
206-245	205.5-245.5	225.5
246-285	245.5-285.5	265.5

To find the decimal or original data, divide the class limits, class boundaries and midpoints by 100. The calculation as follows:

class limits	class boundaries	Midpoints
0.46-0.85	0.455-0.855	0.655
0.86-1.25	0.855-1.255	1.055
1.26-1.65	1.255-1.655	1.455
1.66-2.05	1.655-2.055	1.855
2.06-2.45	2.055-2.455	2.255
2.46-2.85	2.455-2.855	2.655

Interpretation: Hence, the data divide by 100 is as:

class limits	class boundaries	Midpoints
0.46-0.85	0.455-0.855	0.655
0.86-1.25	0.855-1.255	1.055
1.26-1.65	1.255-1.655	1.455
1.66-2.05	1.655-2.055	1.855
2.06-2.45	2.055-2.455	2.255
2.46-2.85	2.455-2.855	2.655