
Concept explainers
A 10-kg block is attached to spring A and connected to spring B by a cord and pulley. The block is held in the position shown with both springs unstretched when the support is removed and the block is released with no initial velocity. Knowing that the constant of each spring is 2 kN/m, determine (a) the velocity of the block after it has moved down 50 mm, (b) the maximum velocity achieved by the block.
Fig. P13.30
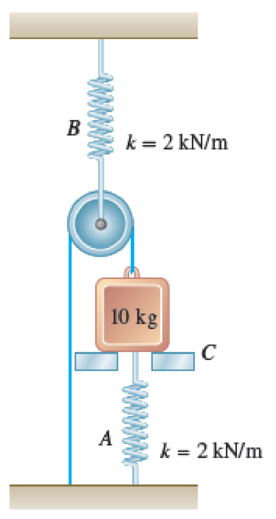
(a)
Find the velocity (v) of the block after it has moved down 50mm.
Answer to Problem 13.30P
The velocity (v) of the block after it has moved down 50mm is 0.597m/s_.
Explanation of Solution
Given information:
The mass of the block (m) is 10kg.
The spring constant at A (kA) is 2kN/m.
The spring constant at B (kB) is 2kN/m.
The depth where the spring A moves down (xA) is 50mm or 0.05m.
Assume the acceleration due to gravity (g) is 9.81m/s2.
Calculation:
Show the free body diagram of the block with two spring’s attachment acting as in Figure (1).
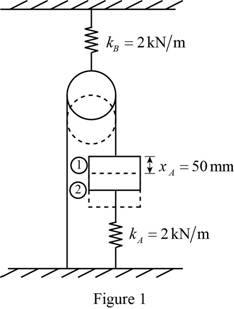
Calculate the depth of spring B moves down due to block (xB) using the formula:
xB=12xA
Substitute 0.05m for xA.
xB=12(0.05)=0.025m
Calculate the weight of the block (W) using the relation:
W=mg
Substitute 10kg for m and 9.81m/s2 for g.
W=(10)(9.81)=98.1N
Here, the initial kinetic energy (T1) is zero respectively.
Calculate the final kinetic energy (T2) using the formula:
T2=12mv2
Substitute 10kg for m.
T2=12(10)v2=5v2
Calculate the work done (U1−2)G due to gravity using the formula:
(U1−2)G=WxA
Substitute 98.1N for W and 0.05m for xA.
(U1−2)G=(98.1)0.05=4.905N⋅m
Calculate the work done (U1−2)A due to spring A using the formula:
(U1−2)A=12kAx2A
Substitute 0.05m for xA and 2kN/m for kA.
(U1−2)A=12(2×103)(0.05)2=2.5N⋅m
Calculate the work done (U1−2)B due to spring B using the formula:
(U1−2)B=12kBx2B
Substitute 0.025m for xB and 2kN/m for kB.
(U1−2)B=12(2×103)(0.025)2=0.625N⋅m
Calculate the total work done (U1−2) using the relation:
(U1−2)=(U1−2)G−(U1−2)A−(U1−2)B
Substitute 4.905N⋅m for (U1−2)G, 2.5N⋅m for (U1−2)A, and 0.625N⋅m for (U1−2)B.
(U1−2)=4.905−2.5−0.625=1.78N⋅m
Use work and energy principle which states that kinetic energy of the particle at a displaced point can be obtained by adding the initial kinetic energy and the work done on the particle during its displacement.
Find the velocity (v) of the block after it has moved down 50mm:
T1+U1−2=T2
Substitute 0 for T1, 1.78N⋅m for U1−2, and 5v2 for T2.
0+(1.78N⋅m)=5v2v=√1.785v=0.597m/s
Therefore, the velocity (v) of the block after it has moved down 50mm is 0.597m/s_.
(b)
Find the maximum velocity (vmax) achieved by the block.
Answer to Problem 13.30P
The maximum velocity (vmax) achieved by the block is 0.620m/s_
Explanation of Solution
Given information:
The mass of the block (m) is 10kg.
The spring constant at A (kA) is 2kN/m.
The spring constant at B (kB) is 2kN/m.
The depth where the spring A moves down (xA) is 50mm or 0.05m.
Assume the acceleration due to gravity (g) is 9.81m/s2.
Calculation:
Assume x be the distance moved down by the 10kg block.
Calculate the work done (U1−2)G due to gravity using the formula:
(U1−2)G=Wx
Substitute 98.1N for W.
(U1−2)G=98.1x
Calculate the work done (U1−2)A due to spring A using the formula:
(U1−2)A=12kAx2
Substitute 2kN/m for kA.
(U1−2)A=12(2×103)x2=1000x2
Calculate the work done (U1−2)B due to spring B using the formula:
(U1−2)B=12kB(x2)2
Substitute 2kN/m for kB.
(U1−2)B=12(2×103)(x2)2=1000(x24)=250x2
Calculate the total work done (U1−2) using the relation:
(U1−2)=(U1−2)G−(U1−2)A−(U1−2)B
Substitute 98.1x for (U1−2)G, 1000x2 for (U1−2)A, and 250x2 for (U1−2)B.
(U1−2)=98.1x−1000x2−250x2=98.1x−1250x2 (1)
Differentiate the above equation with respect to ‘x’.
dU1−2dx=0
Substitute 98.1x−1250x2 for U1−2.
ddx(98.1x−1250x2)=098.1−2500x=0x=98.12,500x=0.03924m(39.24mm)
Substitute 0.03924m for x in Equation (1).
(U1−2)=98.1(0.03924)−1000(0.03924)2−250(0.03924)2=1.925N⋅m
Use work and energy principle which states that kinetic energy of the particle at a displaced point can be obtained by adding the initial kinetic energy and the work done on the particle during its displacement.
Find the maximum velocity (vmax) achieved by the block:
T1+U1−2=T2
Substitute 0 for T1, 1.925N⋅m for U1−2, and 5v2 for T2.
0+(1.925N⋅m)=5v2v=√1.9255v=0.620m/s
Therefore, the maximum velocity (vmax) achieved by the block is 0.620m/s_.
Want to see more full solutions like this?
Chapter 13 Solutions
Vector Mechanics for Engineers: Statics and Dynamics
- CORRECT AND DETAILED HANDWRITTEN SOLUTION WITH FBD ONLY. I WILL UPVOTE THANK YOU. CORRECT ANSWER IS ALREADY PROVIDED. The cantilevered spandrel beam shown whose depth tapers from d1 to d2, has a constant width of 120mm. It carries a triangularly distributed end reaction.Given: d1 = 600 mm, d2 = 120 mm, L = 1 m, w = 100 kN/m1. Calculate the maximum flexural stress at the support, in kN-m.2. Determine the distance (m), from the free end, of the section with maximum flexural stress.3. Determine the maximum flexural stress in the beam, in MPa.ANSWERS: (1) 4.630 MPa; (2) 905.8688 m; (3) 4.65 MPaarrow_forwardCORRECT AND DETAILED HANDWRITTEN SOLUTION WITH FBD ONLY. I WILL UPVOTE THANK YOU. CORRECT ANSWER IS ALREADY PROVIDED. A concrete wall retains water as shown. Assume that the wall is fixed at the base. Given: H = 3 m, t = 0.5m, Concrete unit weight = 23 kN/m3Unit weight of water = 9.81 kN/m3(Hint: The pressure of water is linearly increasing from the surface to the bottom with intensity 9.81d.)1. Find the maximum compressive stress (MPa) at the base of the wall if the water reaches the top.2. If the maximum compressive stress at the base of the wall is not to exceed 0.40 MPa, what is the maximum allowable depth(m) of the water?3. If the tensile stress at the base is zero, what is the maximum allowable depth (m) of the water?ANSWERS: (1) 1.13 MPa, (2) 2.0 m, (3) 1.20 marrow_forwardCORRECT AND DETAILED HANDWRITTEN SOLUTION WITH FBD ONLY. I WILL UPVOTE THANK YOU. CORRECT ANSWER IS ALREADY PROVIDED. A short plate is attached to the center of the shaft as shown. The bottom of the shaft is fixed to the ground.Given: a = 75 mm, h = 125 mm, D = 38 mmP1 = 24 kN, P2 = 28 kN1. Calculate the maximum torsional stress in the shaft, in MPa.2. Calculate the maximum flexural stress in the shaft, in MPa.3. Calculate the maximum horizontal shear stress in the shaft, in MPa.ANSWERS: (1) 167.07 MPa; (2) 679.77 MPa; (3) 28.22 MPaarrow_forward
- A counter flow double pipe heat exchanger is being used to cool hot oil from 320°F to 285°F using cold water. The water, which flows through the inner tube, enters the heat exchanger at 70°F and leaves at 175°F. The inner tube is ¾-std type L copper. The overall heat transfer coefficient based on the outside diameter of the inner tube is 140 Btu/hr-ft2-°F. Design conditions call for a total heat transfer duty (heat transfer rate between the two fluids) of 20,000 Btu/hr. Determine the required length of this heat exchanger (ft).arrow_forward! Required information A one-shell-pass and eight-tube-passes heat exchanger is used to heat glycerin (cp=0.60 Btu/lbm.°F) from 80°F to 140°F by hot water (Cp = 1.0 Btu/lbm-°F) that enters the thin-walled 0.5-in-diameter tubes at 175°F and leaves at 120°F. The total length of the tubes in the heat exchanger is 400 ft. The convection heat transfer coefficient is 4 Btu/h-ft²°F on the glycerin (shell) side and 70 Btu/h-ft²°F on the water (tube) side. NOTE: This is a multi-part question. Once an answer is submitted, you will be unable to return to this part. Determine the rate of heat transfer in the heat exchanger before any fouling occurs. Correction factor F 1.0 10 0.9 0.8 R=4.0 3.0 2.0.15 1.0 0.8.0.6 0.4 0.2 0.7 0.6 R= T1-T2 12-11 0.5 12-11 0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 (a) One-shell pass and 2, 4, 6, etc. (any multiple of 2), tube passes P= T₁-11 The rate of heat transfer in the heat exchanger is Btu/h.arrow_forward! Required information Air at 25°C (cp=1006 J/kg.K) is to be heated to 58°C by hot oil at 80°C (cp = 2150 J/kg.K) in a cross-flow heat exchanger with air mixed and oil unmixed. The product of heat transfer surface area and the overall heat transfer coefficient is 750 W/K and the mass flow rate of air is twice that of oil. NOTE: This is a multi-part question. Once an answer is submitted, you will be unable to return to this part. Air Oil 80°C Determine the effectiveness of the heat exchanger.arrow_forward
- In an industrial facility, a counter-flow double-pipe heat exchanger uses superheated steam at a temperature of 155°C to heat feed water at 30°C. The superheated steam experiences a temperature drop of 70°C as it exits the heat exchanger. The water to be heated flows through the heat exchanger tube of negligible thickness at a constant rate of 3.47 kg/s. The convective heat transfer coefficient on the superheated steam and water side is 850 W/m²K and 1250 W/m²K, respectively. To account for the fouling due to chemical impurities that might be present in the feed water, assume a fouling factor of 0.00015 m²-K/W for the water side. The specific heat of water is determined at an average temperature of (30 +70)°C/2 = 50°C and is taken to be J/kg.K. Cp= 4181 Water Steam What would be the required heat exchanger area in case of parallel-flow arrangement? The required heat exchanger area in case of parallel-flow arrangement is 1m².arrow_forwardA single-pass crossflow heat exchanger is used to cool jacket water (cp = 1.0 Btu/lbm.°F) of a diesel engine from 190°F to 140°F, using air (Cp = 0.245 Btu/lbm.°F) at inlet temperature of 90°F. Both air flow and water flow are unmixed. If the water and air mass flow rates are 85500 lbm/h and 400,000 lbm/h, respectively, determine the log mean temperature difference for this heat exchanger. Assume the correction factor F to be 0.92. Air flow (unmixed) Water flow (unmixed) The log mean temperature difference of the heat exchanger is °F.arrow_forwardusing the theorem of three moments, find all the reactions and supports, I need concise calculations only. the answers are at the bottom, I need concise steps and minimal explanationsarrow_forward
- In an industrial facility, a counter-flow double-pipe heat exchanger uses superheated steam at a temperature of 155°C to heat feed water at 30°C. The superheated steam experiences a temperature drop of 70°C as it exits the heat exchanger. The water to be heated flows through the heat exchanger tube of negligible thickness at a constant rate of 3.47 kg/s. The convective heat transfer coefficient on the superheated steam and water side is 850 W/m²K and 1250 W/m²K, respectively. To account for the fouling due to chemical impurities that might be present in the feed water, assume a fouling factor of 0.00015 m² K/W for the water side. The specific heat of water is determined at an average temperature of (30+70)°C/2 = 50°C and is taken to be Cp J/kg-K. Water Steam Determine the heat exchanger area required to maintain the exit temperature of the water to a minimum of 70°C. The heat exchanger area required isarrow_forwardStress, ksi 160 72 150- 140 80 70 ༄ ྃ ༈ ཎྜ རྦ ༅ ཎྜ ྣཧྨ ➢ 130 120 110 100 90 2.0 2.8 3.6 4.4 5 Wire diameter, mm 6.0 6.8 2 7.6 8.4 Compression and extension springs. ASTM A227 Class II Light service Average service 0.020 0.060 0.100 0.140 0.180 0.220 0.260 0.300 0.340 0.380 0.420 0.460 0.500 Wire diameter, in Torsional stress due to initial tension, ksi 10 ४ 20 Preferred range 100 Stress, MPa 9.2 10.0 10.8 11.6 12.4 1100 1035 965 895 825 760 Severe service 690 620 550 50 150 3456789 10 11 12 13 14 15 16 Spring index, C = DJD FIGURE 18-21 Recommended torsional shear stress in an extension spring due to initial tension (Data from Associated Spring, Barnes Group, Inc.) 50 200 485 Stress, MPaarrow_forwardBolted Joint Design Bolted Frames Total Force due to door weight: P = 240 lb Number of Bolts: N = Distance to Bolt C/L: a = 4 N/A Bolt Material - Allowable shear stress of bolt material: T₂ = x Distance from Bolt centroid to bolt: x = y Distance from Bolt centroid to bolt: y = Degrees per Radian- Results y-Load on each bolt: F, = Moment resisted by bolt pattern: M = Radial distance from Bolt centroid to bolt: r = Sum squares of all radial distances: Σr² Force on each bolt to resist moment: F, - Angle for force composition: e= X-Force on each bolt to resist moment: F- y-Force on each bolt to resist moment: Fly Total y-Force on each bolt: Fy = Resultant force on bolt 1: R₁ = Required shear stress area for a bolt: A₂ = ASTM Grade A307 Steel 10,000 0 psi from Table 20-1 3.0 57.296 in degrees lb per bolt lb-in Formula FS-P/N M-Px XB r = (x² + y²)0.5 in² Σ 4r² Mr F₁ = Στ lb degrees lb lb lb Minimum Bolt Diameter: Din = Rounded up Bolt Diameter: D = 55 P. 1.5 in 2 in (3x) 1 in This bracket…arrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





