Elementary Statistics: Picturing the World (7th Edition)
7th Edition
ISBN: 9780134683416
Author: Ron Larson, Betsy Farber
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 11.2, Problem 1TY
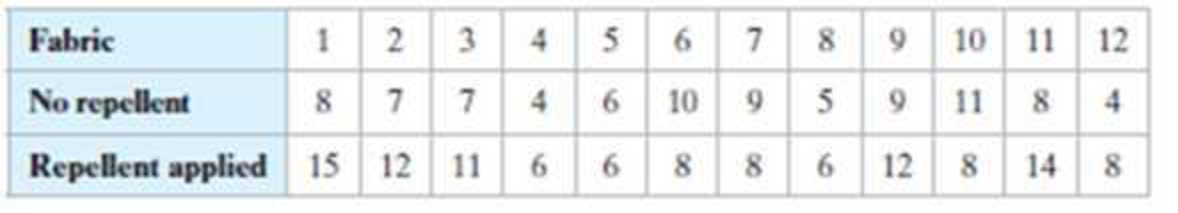
A quality control inspector wants to test the claim that a spray-on water repellent is effective. To test this claim, he selects 12 pieces of fabric, sprays water on each, and measures the amount of water repelled (in milliliters). He then applies the water repellent and repeats the experiment. The table shows the results. At a = 0.01, can he conclude that the water repellent is effective?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
An automobile battery manufacturer offers a 39/50 warranty on its batteries. The first number in the warranty code is the free-replacement period; the second number is the prorated-credit period. Under this warranty, if a battery fails within 39 months of purchase, the manufacturer replaces the battery at no charge to the consumer. If the battery fails after 39 months but within 50 months, the manufacturer provides a prorated credit toward the purchase of a new battery.
The manufacturer assumes that X, the lifetime of its auto batteries, is normally distributed with a mean of 44 months and a standard deviation of 3.6 months.
Use the following Distributions tool to help you answer the questions that follow. (Hint: When you adjust the parameters of a distribution, you must reposition the vertical line (or lines) for the correct areas to be displayed.)
0123
Select a Distribution
If the manufacturer’s assumptions are correct, it would need to replace of its…
In regards to conducting a linear contrast after a one-way ANOVA, can you explain how seemingly arbitrary weights that "emphasize or de-emphasize" certain variables in a linear combination and sum to zero are able to provide information about how certain groups differ from each other? For example, if we havethree groups A, B, and C, and we want tocompare the mean of group A with theaverage of groups B and C, the weights inthis case are 1 for group A, and -0.5 for groupsB and C, which sum to zero. But how do these numbers model the relationship of comparing one group to the average of the other two? Does it have to do with how the math is carried out, such as how the test statistic is created?
Can you simply and intuitively explain the purpose of a contrast to the treatment sum of squares? For example, do orthogonal contrasts partition the treatment sum of squares into additive components that represent the variation due to each contrast? If so, what would be the purpose of this?
Chapter 11 Solutions
Elementary Statistics: Picturing the World (7th Edition)
Ch. 11.1 - A real estate agency claims that the median number...Ch. 11.1 - An organization claims that the median age of...Ch. 11.1 - A medical researcher claims that a new vaccine...Ch. 11.1 - Prob. 1ECh. 11.1 - When the sign test is used, what population...Ch. 11.1 - Describe the test statistic for the sign test when...Ch. 11.1 - In your own words, explain why the hypothesis test...Ch. 11.1 - Explain how to use the sign test to test a...Ch. 11.1 - List the two conditions that must be met in order...Ch. 11.1 - Performing a Sign Test In Exercises 722, (a)...
Ch. 11.1 - Temperature A meteorologist claims that the median...Ch. 11.1 - Prob. 9ECh. 11.1 - Temperature During a weather report, a...Ch. 11.1 - Credit Card Debt A financial services institution...Ch. 11.1 - Financial Debt A financial services accountant...Ch. 11.1 - Prob. 13ECh. 11.1 - Social Networking A research group claims that the...Ch. 11.1 - Unit Size A renters organization claims that the...Ch. 11.1 - Prob. 16ECh. 11.1 - Hourly Wages A labor organization claims that the...Ch. 11.1 - Prob. 18ECh. 11.1 - Prob. 19ECh. 11.1 - Prob. 20ECh. 11.1 - Prob. 21ECh. 11.1 - Prob. 22ECh. 11.1 - Prob. 23ECh. 11.1 - Contacting Parents A research organization...Ch. 11.1 - In Exercises 2528, use a right-tailed test and (a)...Ch. 11.1 - Prob. 26ECh. 11.1 - Prob. 27ECh. 11.1 - Ages of Grooms A marriage counselor claims that...Ch. 11.2 - A quality control inspector wants to test the...Ch. 11.2 - Prob. 2TYCh. 11.2 - Prob. 1ECh. 11.2 - Prob. 2ECh. 11.2 - Prob. 3ECh. 11.2 - Prob. 4ECh. 11.2 - Prob. 5ECh. 11.2 - Prob. 6ECh. 11.2 - Performing a Wilcoxon Test In Exercises 38, (a)...Ch. 11.2 - Performing a Wilcoxon Test In Exercises 38, (a)...Ch. 11.2 - Wilcoxon Signed-Rank Test for n 30 When you are...Ch. 11.2 - Prob. 10ECh. 11.2 - Construct a side-by-side box-and-whisker plot for...Ch. 11.2 - Prob. 2CSCh. 11.2 - In Exercises 25, use the sign test to test the...Ch. 11.2 - In Exercises 25, use the sign test to test the...Ch. 11.2 - In Exercises 25, use the sign test to test the...Ch. 11.2 - In Exercises 6 and 7, use the Wilcoxon rank sum...Ch. 11.2 - In Exercises 6 and 7, use the Wilcoxon rank sum...Ch. 11.3 - Prob. 1TYCh. 11.3 - Prob. 1ECh. 11.3 - Explain why the Kruskal-Wallis test is always a...Ch. 11.3 - Prob. 3ECh. 11.3 - Performing a Kruskal-Wallis Test In Exercises 36,...Ch. 11.3 - Prob. 5ECh. 11.3 - Prob. 6ECh. 11.3 - Comparing Two Tests In Exercises 7 and 8, (a)...Ch. 11.3 - Comparing Two Tests In Exercises 7 and 8, (a)...Ch. 11.4 - Prob. 1TYCh. 11.4 - Prob. 1ECh. 11.4 - Prob. 2ECh. 11.4 - Prob. 3ECh. 11.4 - Prob. 4ECh. 11.4 - Prob. 5ECh. 11.4 - Prob. 6ECh. 11.4 - Prob. 7ECh. 11.4 - Prob. 8ECh. 11.4 - Prob. 9ECh. 11.4 - Prob. 10ECh. 11.4 - Prob. 11ECh. 11.4 - Prob. 12ECh. 11.4 - Prob. 13ECh. 11.4 - Prob. 14ECh. 11.5 - A machine produces engine parts. An inspector...Ch. 11.5 - Prob. 2TYCh. 11.5 - Prob. 3TYCh. 11.5 - In your own words, explain why the hypothesis test...Ch. 11.5 - Describe the test statistic for the runs test when...Ch. 11.5 - Finding the Number of Runs In Exercises 36,...Ch. 11.5 - Prob. 4ECh. 11.5 - Finding the Number of Runs In Exercises 36,...Ch. 11.5 - Prob. 6ECh. 11.5 - Prob. 7ECh. 11.5 - Prob. 8ECh. 11.5 - Prob. 9ECh. 11.5 - Prob. 10ECh. 11.5 - Finding Critical Values In Exercises 1114, use the...Ch. 11.5 - Prob. 12ECh. 11.5 - Prob. 13ECh. 11.5 - Prob. 14ECh. 11.5 - Prob. 15ECh. 11.5 - Prob. 16ECh. 11.5 - Prob. 17ECh. 11.5 - Prob. 18ECh. 11.5 - Prob. 19ECh. 11.5 - Prob. 20ECh. 11.5 - Prob. 21ECh. 11.5 - Prob. 22ECh. 11.5 - Runs Test with Quantitative Data In Exercises...Ch. 11 - Using an Inappropriate Test Discuss the...Ch. 11 - Prob. 11.1.1RECh. 11 - Prob. 11.1.2RECh. 11 - Prob. 11.1.3RECh. 11 - Prob. 11.1.4RECh. 11 - Prob. 11.1.5RECh. 11 - Prob. 11.1.6RECh. 11 - In Exercises 7 and 8, use a Wilcoxon test to test...Ch. 11 - In Exercises 7 and 8, use a Wilcoxon test to test...Ch. 11 - Prob. 11.3.9RECh. 11 - Prob. 11.3.10RECh. 11 - Prob. 11.4.11RECh. 11 - Prob. 11.4.12RECh. 11 - Prob. 11.5.13RECh. 11 - Prob. 11.5.14RECh. 11 - Prob. 1CQCh. 11 - Prob. 2CQCh. 11 - Prob. 3CQCh. 11 - Prob. 4CQCh. 11 - Prob. 5CQCh. 11 - Prob. 1CTCh. 11 - Prob. 2CTCh. 11 - Prob. 3CTCh. 11 - An employment agency claims that there is a...Ch. 11 - Prob. 5CTCh. 11 - How Would You Do It? (a) What sampling technique...Ch. 11 - In a recent year, according to the Bureau of Labor...Ch. 11 - Prob. 3RSRDCh. 11 - Construct a box-and-whisker plot for each region....Ch. 11 - In Exercises 15, refer to the annual incomes of...Ch. 11 - Use technology to perform a Wilcoxon rank sum test...Ch. 11 - Prob. 4TCh. 11 - Prob. 5TCh. 11 - Prob. 6T
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- The height of the graph of the probability density function f(x) varies with X as follows (round to four decimal places): X 16 Height of the Graph of the Probability Density Function You are flying out of Terminal 3 at JFK on a Wednesday afternoon between 3:00 and 4:00 PM. You get stuck in a traffic jam on the way to the airport, and if it takes you longer than 12 minutes to clear security, you'll miss your flight. The probability that you'll miss your flight is You have arrived at the airport and have been waiting 10 minutes at the security checkpoint. Recall that if you spend more than 12 minutes clearing security, you will miss your flight. Now what is the probability that you'll miss your flight? ○ 0.5 O 0.25 ○ 0.8333 ○ 0.6667arrow_forwardonsider a random variable x that follows a uniform distribution, with a = 2 and b = 9. What is the probability that x is less than 6? P(x < 6) = 0.2857 P(x < 6) = 0.5714 P(x < 6) = 0.17142 P(x < 6) = 0.4286 What is the probability that x is between 4 and 6? P(4 ≤ x ≤ 6) = 0.2857 P(4 ≤ x ≤ 6) = 0.157135 P(4 ≤ x ≤ 6) = 0.0928525 P(4 ≤ x ≤ 6) = 0.11428arrow_forwardConsider a random variable x that follows a uniform distribution, with a = 8 and b = 14. What is the probability that x is less than 13? P(x < 13) = 0.1667 P(x < 13) = 0.41665 P(x < 13) = 0.24999 P(x < 13) = 0.8333 What is the probability that x is between 11 and 12? P(11 ≤ x ≤ 12) = 0.0541775 P(11 ≤ x ≤ 12) = 0.1667 P(11 ≤ x ≤ 12) = 0.06668 P(11 ≤ x ≤ 12) = 0.091685arrow_forward
- please solve this problem step by step and make it quick pleasearrow_forwardWHAT IS THE CORRECT ANSWER AND WHY?arrow_forwardA common way for two people to settle a frivolous dispute is to play a game of rock-paper-scissors. In this game, each person simultaneously displays a hand signal to indicate a rock, a piece of paper, or a pair of scissors. Rock beats scissors, scissors beats paper, and paper beats rock. If both players select the same hand signal, the game results in a tie. Two roommates, roommate A and roommate B, are expecting company and are arguing over who should have to wash the dishes before the company arrives. Roommate A suggests a game of rock-paper-scissors to settle the dispute. Consider the game of rock-paper-scissors to be an experiment. In the long run, roommate A chooses rock 21% of the time, and roommate B chooses rock 61% of the time; roommate A selects paper 39% of the time, and roommate B selects paper 21% of the time; roommate A chooses scissors 40% of the time, and roommate B chooses scissors 18% of the time. (These choices are made randomly and independently of each…arrow_forward
- A qualifying exam for a graduate school program has a math section and a verbal section. Students receive a score of 1, 2, or 3 on each section. Define X as a student’s score on the math section and Y as a student’s score on the verbal section. Test scores vary according to the following bivariate probability distribution. y 1 2 3 1 0.22 0.33 0.05 x 2 0.00 0.08 0.20 3 0.07 0.05 0.00 μXX = , and μYY = σXX = , and σYY = The covariance of X and Y is . The coefficient of correlation is . The variables X and Y independent. The expected value of X + Y is , and the variance of X + Y is . To be accepted to a particular graduate school program, a student must have a combined score of 4 on the qualifying exam. What is the probability that a randomly selected exam taker qualifies for the program? 0.45 0.47 0.46 0.33 Chebysheff’s Theorem states that the…arrow_forwardwhat is the correct answer and why?arrow_forward(a) How many bit strings of length 10 both begin with a 1 and end with 2 zeroes? (b) How many permutations of the letters PQRSTUV contain PRS and QV?arrow_forward
- (d) A clothing store sells red, white, green, orange and pink charms for a specialty bracelet. How many ways can a customer purchase a bracelet with (i) 16 charms? (ii) 27 charms with at least 3 of each colour?arrow_forward(d) Draw the Venn diagram which represents the set (A U B) U (B NC).arrow_forwardThe ages of undergraduate students at two universities (one in the east and one in the west) are being compared. Researchers want to know if there is a difference in the mean age of students at the two universities. The population standard deviations are known. The following data shows the results of samples collected at each institution: School Location n sample mean population std. dev. West 33 26.78 6.29 East 35 23.16 7.52 What is the value of the test statistic for this problem? what is the p-value? what is the decision (reject or do not reject the null hypothesis?arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
College Algebra (MindTap Course List)
Algebra
ISBN:9781305652231
Author:R. David Gustafson, Jeff Hughes
Publisher:Cengage Learning
Use of ALGEBRA in REAL LIFE; Author: Fast and Easy Maths !;https://www.youtube.com/watch?v=9_PbWFpvkDc;License: Standard YouTube License, CC-BY
Compound Interest Formula Explained, Investment, Monthly & Continuously, Word Problems, Algebra; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=P182Abv3fOk;License: Standard YouTube License, CC-BY
Applications of Algebra (Digit, Age, Work, Clock, Mixture and Rate Problems); Author: EngineerProf PH;https://www.youtube.com/watch?v=Y8aJ_wYCS2g;License: Standard YouTube License, CC-BY