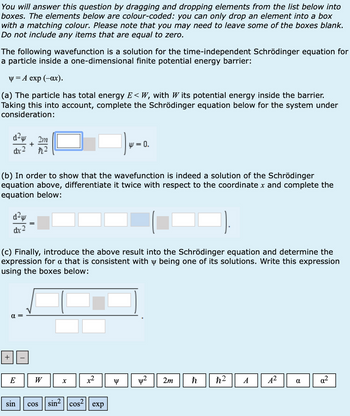
You will answer this question by dragging and dropping elements from the list below into boxes. The elements below are colour-coded: you can only drop an element into a box with a matching colour. Please note that you may need to leave some of the boxes blank. Do not include any items that are equal to zero. The following wavefunction is a solution for the time-independent Schrödinger equation for a particle inside a one-dimensional finite potential energy barrier: y=A exp (-ax). (a) The particle has total energy E

There is an electron, in a 1-d, infinitely deep square potential well with a width of d. If it is in ground state,  1. Draw the electron's wavefunction. Show the position of the walls of the potential well. 2. Explain how the probability distribution for detecting the electron at a given position differs from the wavefunction.

We will consider the Schrödinger equation in this problem as well as the analogies between the wavefunction and how boundary conditions are an essential part of developing this equation for various problems (situations). a) Write the form of the time-independent Schrödinger equation if the potential is that of a spring with spring constant k. Write the form of the time-dependent Schrödinger equation with the same potential. Briefly describe all the terms and variables in these equations. b) One solution to the time independent Schrödinger equation has the form Asin(kx). Why might it be called the wavefunction? If this form represents a wave of light, what is the energy for one photon? (Notek here stands for the wavevector and not the spring constant.) c) Why must all wavefunctions go to zero at infinite distance from the center of the coordinate system in all systems where the potential energy is always finite?

Question A3 Consider the energy eigenstates of a particle in a quantum harmonic oscillator with frequency w. a) Write down expressions for the energies of the three lowest states. b) c) Sketch the potential for this system, along with the position of the three lowest energy levels. Add to your sketch the form of the wavefunction and the probability density in the three lowest energy states. [10 marks]