
Calculus: Early Transcendentals
8th Edition
ISBN: 9781285741550
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
Write and find the general solution of the differential equation that models the verbal statement.
The rate of change of N is proportional to N. (Use k for the proportionality constant.)
|
= |
kN
|
||
| N(t) | = |
Cc4t
|
Evaluate the solution at the specified value of the independent variable.
When t = 0, N = 150, and when t = 1, N = 200. What is the value of N when t = 4?
Expert Solution
arrow_forward
Step 1
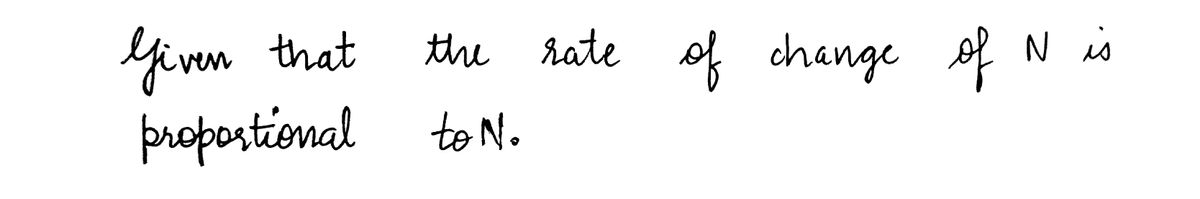
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- Help1arrow_forwardSolve the problem. dV The growth rate of a certain stock is modeled by = k(25 - V), V = 20 when t 0, dt %3D where V is the value of the stock, per share, after time t (in months), and k = a constant. Find the solution to the differential equation in terms of t and k. O V = 25 - 25e-kt O V = 25-5ekt %3D O V = 5 - 25e-kt O V = 25- 5e-ktarrow_forwardDifferential Equationarrow_forward
- A virus spreads by contact and if you get infected you stay contagious. In an isolated population with P persons (no-one dies or are born) the rate of infection at time t (in months after 1/1 2020) is proportional to the product of 1. the number y(t) that are infected, 2. the number that are not infected 3. eat where a is a negative number depending on disease preventive measures. One tenth of the population is infected 1/1-2020. (a) Write out the differential equation y(t) must satisfy and solve it (call the proportionality constant k and remember to explain each step of your solution carefully. Are there for instance any constant solutions?). (b) Find a relation between L = lim->00 y(t), a, Pand k. What can you say about L when a is very small? What can you say about L when a is very big?arrow_forwarddT dt Newton's law of cooling states that the rate of change in the temperature T(t) of a body is proportional to the difference between the temperature of the medium M(t) and the temperature of the body. That is = K[M(t)- T(t)], where K is a constant. Let K = 0.04 (min) temperature of the medium be constant, M(t) = 291 kelvins. If the body is initially at 365 kelvins, use Euler's method with h = 0.1 min to approximate the temperature of the body after (a) 30 minutes and (b) 60 minutes. (a) The temperature of the body after 30 minutes is kelvins. (Round to two decimal places as needed.) (...) and thearrow_forwarddC The rate of change in the concentration of a drug with respect to time in a user's blood is given by = - kC + D(t), where D(t) is dosage at time t and k is the rate that the drug leaves the bloodstream. Complete dt parts (a) and (b) below. t (a) Solve this linear equation to show that, if C(0) = 0, then C(t) = e -kt ,ky D(y)dy. dC dy Begin by writing the differential equation, = - kC + D(t), in the form dt + P(x)y = Q(x). dx dC + %3D dtarrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning

Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning