Weekly Usage (hours) Annual Maintenance Expense ($100s) 13 17.0 22.0 30.0 37.0 10 20 28 32 47.0 17 30.5 24 32.5 31 39.0 40 51.5 38 40.0

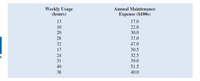
Jensen Tire & Auto is deciding whether to purchase a maintenance contract for its new
computer wheel alignment and balancing machine. Managers feel that maintenance expense
should be related to usage, and they collected the following information on weekly
usage (hours) and annual maintenance expense (in hundreds of dollars).

a. Develop a scatter chart with weekly usage hours as the independent variable. What
does the scatter chart indicate about the relationship between weekly usage and annual
maintenance expense?
b. Use the data to develop an estimated regression equation that could be used to predict
the annual maintenance expense for a given number of hours of weekly usage. What
is the estimated regression model?

c. Test whether each of the regression parameters b0 and b1 is equal to zero at a 0.05
level of significance. What are the correct interpretations of the estimated regression
parameters? Are these interpretations reasonable?
d. How much of the variation in the sample values of annual maintenance expense does
the model you estimated in part b explain?
e. If the maintenance contract costs $3,000 per year, would you recommend purchasing
it? Why or why not?