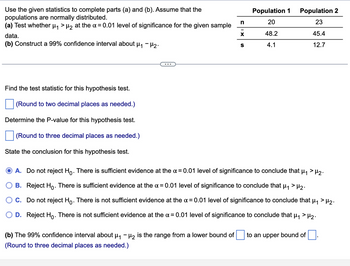
Use the given statistics to complete parts (a) and (b). Assume that the populations are normally distributed. (a) Test whether μ₁ μ₂ at the α = 0.01 level of significance for the given sample data. (b) Construct a 99% confidence interval about μ₁-H₂. Find the test statistic for this hypothesis test. (Round to two decimal places as needed.) Determine the P-value for this hypothesis test. (Round to three decimal places as needed.) State the conclusion for this hypothesis test. ... n X S Population 1 Population 2 20 23 48.2 4.1 45.4 12.7 ⒸA. Do not reject Ho. There is sufficient evidence at the x = 0.01 level of significance to conclude that μ₁ > μ₂. B. Reject Ho. There is sufficient evidence at the α = 0.01 level of significance to conclude that μ₁ > μ₂. C. Do not reject Ho. There is not sufficient evidence at the α = 0.01 level of significance to conclude that μ₁ >μ₂. D. Reject Ho. There is not sufficient evidence at the x = 0.01 level of significance to conclude that µ₁ >µ₂. (b) The 99% confidence interval about μ₁ −µ₂ is the range from a lower bound of to an upper bound of (Round to three decimal places as needed.)

Test the claim about the population​ mean, μ​,at the given level of significance using the given sample statistics. ​Claim: μ=50​; α=0.08​; σ=3.95. Sample​ statistics: x=49.7​, n=67 a. Identify the null and alternative hypotheses b. Calculate the standardized test statistic. c. Determine the critical​ value(s). d. Determine the outcome and conclusion of the test.

Use the given statistics to complete parts​ (a) and​ (b). Assume that the populations are normally distributed. ​(a) Test whether μ1>μ2 at the α=0.01 level of significance for the given sample data. ​(b) Construct a 99​% confidence interval about μ1−μ2.     Population 1 Population 2   n 21 18 x 45.8 43.5 s 5.7 11.8

Test the claim about the population​ mean, μ​, at the given level of significance using the given sample statistics. ​Claim: μ≠6000​; α=0.08​; σ=391. Sample​ statistics: x=5900​, n=49   Determine the outcome and conclusion of the test. Choose from the following. A. Reject H0. At the 8​% significance​ level, there is enough evidence to support the claim. B. Fail to reject H0. At the 8​% significance​ level, there is not enough evidence to support the claim. C. Fail to reject H0. At the 8​% significance​ level, there is not enough evidence to reject the claim. D. Reject H0. At the 8​% significance​ level, there is enough evidence to reject the claim.

Use the t-distribution and the sample results to complete the test of the hypotheses. Use a 5 % significance level. Assume the results come from a random sample, and if the sample size is small, assume the underlying distribution is relatively normal. Test Ho : µ = 10 vs Ha : µ > 10using the sample resultsI = 13.2, s = 8.7, with n = 12. (a) Give the test statistic and the p-value. Round your answer for the test statistic to two decimal places and your answer for the p-value to three decimal places. test statistic = p-value =

Use the given statistics to complete parts (a) and (b). Assume that the populations are normally distributed. (a) Test whether u, >uz at the a = 0.05 level of significance for the given sample data. (b) Construct a 99% confidence interval about u1 -P2. Population 2 23 Population 1 22 46.4 41.8 4.3 13.1 (a) Identify the null and alternative hypotheses for this test. O A. Ho: H1 = H2 H1: H1 # H2 O B. Ho: H1 =H2 H1: H1 H2 OF. Ho: H1 > H2 H1: H1 = H2 Find the test statistic for this hypothesis test. (Round to two decimal places as needed.)

Use the t-distribution and the given sample results to complete the test of the given hypotheses. Assume the results come from random samples, and if the sample sizes are small, assume the underlying distributions are relatively normal. Test Ho: M₁ = μ₂ vs Ha: ₁ ₂ using the sample results n₂ = = 80. Part 1 test statistic = i = (a) Give the test statistic and the p-value. Round your answer for the test statistic to two decimal places and your answer for the p-value to three decimal places. p-value = i 15.3, s₁ = 11.6 with n₁ = 100 and ₂ = 18.4, 82 = 14.3 with

For the given data, (a) find the test statistic, (b) find the standardized test statistic, (c) decide whether the standardized test statistic is in the rejection region, and (d) decide whether you should reject or fail to reject the null hypothesis. The samples are random and independent. Claim: µ1 < H2, a = 0.01. Sample statistics: x, = 1215, n, = 45, x2 = 1195, and n2 = 75. Population statistics: 01 = 65 and 02 = 120. (a) The test statistic for H1 - H2 is

Use the t-distribution table to find the critical value(s) for the indicated alternative hypotheses, level of significance a, and sample sizes n, and n. Assume that the samples are independent, normal, and random. Answer parts (a) and (b). Hg P2. a= 0.20, n, =6, n2 = 8 (a) Find the critical value(s) assuming that the population variances are equal. (Type an integer or decimal rounded to three decimal places as needed. Use a comma to separate answers as needed.) (b) Find the critical value(s) assuming that the population variances are not equal. (Type an integer or decimal rounded to three decimal places as needed. Use a comma to separate answers as needed.) Enter your answer in each of the answer boxes. DELL

Use the given statistics to complete parts (a) and (b). Assume that the populations are normally distributed. (a) Test whether u1 > H2 at the a = 0.01 level of significance for the given sample data. Population 1 Population 2 24 25 49.6 4.1 (b) Construct a 95% confidence interval about u1 - H2. 45.8 12.7 (a) Identify the null and alternative hypotheses for this test. B. Ho: H1 = H2 H1: H1>H2 O A. Ho: H1 = H2 O C. Ho: H1 + H2 H1: H1 = H2 H1: H1 #H2 O D. Ho: H1 H2 H1: H1 = H2 Find the test statistic for this hypothesis test. (Round to two decimal places as needed.)