The test statistic of z= -16.8 is obtained when testing the claim that p= 1/2

a. Using a significance level of α=0.01, find the critical value(s).

b. Should we reject H0 or should we fail to reject H0?
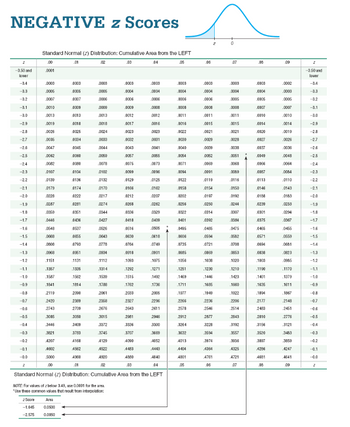
Transcribed Image Text:NEGATIVE z Scores 2 -3.50 and lower -3.4 -3.3 -3.2 -3.1 -3.0 -2.9 -2.8 -2.7 -2.6 -2.5 -24 -2.3 -22 -21 -20 -1.9 -1.8 -1.7 -1.6 -1.5 -1.4 -1.3 -1.2 -1.1 -1.0 -0.9 -0.8 -0.7 -0.6 -0.5 -0.3 -0.2 -0.1 -0.0 2 Standard Normal (2) Distribution: Cumulative Area from the LEFT .00 .01 .02 .03 04 .05 0001 .0003 0005 0007 z Score -1.645 -2.575 .0010 .0013 .0019 .0026 0035 .0047 .0062 0082 .0107 .0139 0179 0228 0287 0359 0446 0548 .0068 0808 0968 1151 .1357 1587 .1841 2119 .2420 2743 3085 .3446 3821 4207 4602 5000 .00 0003 0005 0007 .0009 0013 0018 0025 0034 0.0500 0.0050 0045 .0060 0060 0104 0136 0174 0222 0281 0351 0436 0537 0655 0793 0961 1131 1335 1562 1814 2000 2389 2709 3060 3409 0003 0005 0006 .0009 0013 0018 0024 0033 0044 .0059 .0078 0102 0132 0170 0217 0274 0344 0427 0526 0643 0778 0934 1112 .1314 .1539 .1788 2061 2358 2676 3015 3372 NOTE: For values of z below 3.49, use 0.0001 for the area. *Use these common values that result from interpolation: .0003 .0004 0006 .0009 0012 .0017 .0023 .0032 0043 .0067 .0075 .0099 0129 0166 0212 .0268 .0336 0418 0516 .0630 .0764 0918 .1093 .1292 1515 .1762 2033 2327 2643 .2981 3336 3707 4090 4483 4880 .03 .0003 .0004 .0006 .0008 0012 0016 0023 0031 0041 0065 .0073 .0096 .0125 .0162 .0207 .0262 0329 0409 3783 .3745 4168 4129 4562 4522 4960 4920 .01 .02 Standard Normal (2) Distribution: Cumulative Area from the LEFT .0605 .0618 .0749 .0901 .1075 .1271 1492 1736 2005 2296 2611 2946 3300 3669 4052 4443 4840 04 .0003 .0004 .0006 .0008 .0011 .0016 .0022 0030 .0040 .0054 .0071 0094 0122 .0158 0202 0256 0322 0401 0495 0806 0735 0885 .1056 .1251 .1469 1711 .1977 2266 .2578 .2912 .3264 3632 4013 4404 4801 .05 .06 .0003 .0004 .0006 .0008 .0011 .0015 .0021 .0029 .0009 0062 .0069 .0091 .0119 .0154 .0197 .0250 .0314 .0392 .0485 .0594 .0721 .0869 .1038 1230 .1446 .1685 1949 .2236 2546 2877 3228 .3594 .3974 4364 4761 .06 to .07 .0003 0004 .0005 .0008 .0011 .0015 .0021 .0028 00:38 .0051 .0068 .0069 .0116 0150 0192 .0244 0307 0384 0475 .0582 .0708 0853 .1020 .1210 1423 .1660 1922 2206 2514 2843 3192 3557 .3936 4325 4721 .07 .08 .0003 .0004 .0005 .0007 .0010 .0014 .0020 .0027 .0037 .0049 .0066 .0087 .0113 .0146 .0188 0239 .0301 .0375 0465 .0571 .0694 .0838 .1003 .1190 1401 .1635 .1894 2177 2483 .2810 .3156 3520 .3897 4286 4681 .08 09 .0002 .0003 .0005 .0007 .0010 .0014 .0019 .0026 .0036 .0048 .0064 .0084 .0110 .0143 .0183 0233 .0294 .0367 .0455 .0659 .0681 .0823 .0985 .1170 .1379 .1611 .1867 .2148 .2451 .2776 .3121 3483 38659 4247 4641 09 -3.50 and lower -3.4 -3.3 -3.2 -3.1 -3.0 -2.9 -2.8 -27 -2.6 -2.5 -2.4 -2.3 -2.2 -2.1 -2.0 -1.9 -1.8 -1.7 -1.6 -1.5 -1.4 -1.3 -1.2 -1.1 -1.0 -0.9 -0.8 -0.7 -0.6 -0.5 -0.4 -0.3 -0.2 -0.0
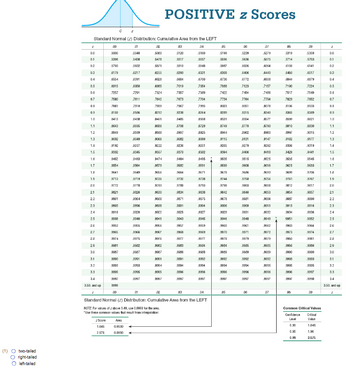
Transcribed Image Text:(1) O two-tailed Oright-tailed O left-tailed Standard Normal (2) Distribution: Cumulative Area from the LEFT .04 z 0.0 0.1 02 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 1.2 1.3 1.4 1.5 1.6 1.7 18 1.9 20 2.1 22 2.3 2.4 2.5 26 2.7 2.8 2.9 3.0 3.1 32 3.3 3.50 and up 2 00 5000 5388 5793 .6179 .6554 6915 7257 7580 .7881 .8159 8413 .8643 zScore 1.645 2.575 9032 .9192 .8332 9452 9554 9713 .9772 9821 .9861 0803 9018 9038 9963 .9965 .9974 9961 9967 9000 9005 9999 .00 .01 5040 5438 5832 6217 6991 6950 7291 7811 .7910 8186 8430 3666 3809 9049 9207 9345 Area 0.9500 0.9950 9463 3564 9649 9719 9778 9826 9064 9806 9820 9940 .9965 9966 .9975 9882 9987 .9991 9993 9996 .9997 5000 5478 5871 6255 6628 6985 7324 7842 .7939 8212 8461 0036 9006 9222 9357 9474 9556 9726 9783 9830 9888 9808 9022 .9966 9967 9976 9902 9987 9001 9005 NOTE: For values of above 3.49, use 0.9999 for the area. *Use these common values that result from interpolation: POSITIVE z Scores 03 5120 5617 5910 .6293 .7019 7357 7673 .7967 8238 8485 .8708 .8907 .9062 .8370 3484 1682 9732 9788 9634 .9871 1001 1025 9043 9957 .9977 9963 9968 1991 9006 9997 03 .5160 .5557 .5948 .6331 .6700 7064 7389 7704 7995 8264 8508 8729 8925 9099 9261 9382 9495 .9591 .9671 9738 .9793 .9638 .9675 9904 9927 9945 .9959 .9969 .01 De 04 Standard Normal (2) Distribution: Cumulative Area from the LEFT .9977 .9984 .9988 9992 9994 9996 9997 .05 .6199 .5596 .5887 .6368 .6736 .7088 7422 .7734 8023 8289 8531 .8749 8944 9115 9265 8394 .9605 0600 .9678 .9744 .9798 .9642 9009 .9960 .9970 .9978 .9984 .9989 9002 9094 9006 .05 .06 5239 5636 6026 6406 .6772 7123 7454 7764 8051 8315 8770 9131 9279 9515 9608 9686 9750 9803 9646 9681 9909 9931 9048 9961 9971 9979 9985 9989 9992 9994 9996 9997 06 .07 .6279 .5675 .6064 .6443 .6308 .7157 7486 .7794 .8078 .8340 .8577 .8790 0900 9147 9292 9418 .9525 .9616 .9693 .9756 .9808 .9850 .9884 .9911 .9932 9049 .9962 .9972 .9979 .9985 .9989 9992 .9995 9996 .9997 .07 05 .5319 .5714 6103 .6450 .6844 7190 7517 .7823 8106 8355 8590 .6810 .8997 .9162 9429 0635 0625 5699 9761 .5812 .9054 .9657 0913 1034 9951 9980 9990 1903 08 .5369 .5753 .6141 .6517 .6879 7224 .7549 7852 .8133 .8389 .8621 .8630 .9015 .9177 .9319 9441 9545 .9633 9706 .9767 .9817 .9667 .9690 0.90 0.96 0.99 9016 9136 9162 9964 .9974 .9981 .9906 .9990 9193 9195 9997 .9998 .09 Common Critical Values Confidence Critical Level Value 1.645 1.96 2.575 0.0 0.1 02 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 1.2 1.3 1.4 1.5 1.6 1.7 1.8 1.9 20 2.1 2.2 2.3 24 25 26 2.7 28 2.9 3.0 3.1 3.2 3.3 - 3.4 3.50 and up

Question

The test statistic of z= -16.8 is obtained when testing the claim that p= 1/2

a. Using a significance level of α=0.01, find the critical value(s).

b. Should we reject H0 or should we fail to reject H0?

Accepted Answer

The test statistic is Claim is

The test statistic of z= -16.8 is obtained when testing the claim that p= 1/2 a. Using a significance level of α=0.01, find the critical value(s). b. Should we reject H0 or should we fail to reject H0​?