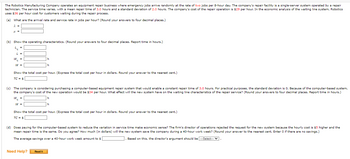
The Robotics Manufacturing Company operates an equipment repair business where emergency jobs arrive randomly at the rate of two jobs per 8-hour day. The company's repair facility is a single-server system operated by a repair technician. The service time varies, with a mean repair time of 3.0 hours and a standard deviation of 2.0 hours. The company's cost of the repair operation is $29 per hour. In the economic analysis of the waiting line system, Robotics uses $36 per hour cost for customers waiting during the repair process. (a) What are the arrival rate and service rate in jobs per hour? (Round your answers to four decimal places.) λ = (c) The company is considering purchasing a computer-based equipment repair system that would enable a constant repair time of 3.0 hours. For practical purposes, the standard deviation is 0. Because of the computer-based system, the company's cost of the new operation would be $34 per hour. What effect will the new system have on the waiting line characteristics of the repair service? (Round your answers to four decimal places. Report time in hours.) W₁ = h h Wa W = (b) Show the operating characteristics. (Round your answers to four decimal places. Report time in hours.) ²g = 9 L = W₁ = W = h Show the total cost per hour. (Express the total cost per hour in dollars. Round your answer to the nearest cent.) TC = $ Show the total cost per hour. (Express the total cost per hour in dollars. Round your answer to the nearest cent.) TC = $ (d) Does paying for the computer-based system to reduce the variation in service time make economic sense? The firm's director of operations rejected the request for the new system because the hourly cost is $5 higher and the mean repair time is the same. Do you agree? How much (in dollars) will the new system save the company during a 40-hour work week? (Round your answer to the nearest cent. Enter O if there are no savings.) The average savings over a 40-hour work week amount to $ . Based on this, the director's argument should be ---Select--- ✓. Need Help? Read It

A waiting-line system that meets the assumptions of M/M/S has λ = 5, μ = 4, and M = 2. For these values, Po is approximately 0.23077, and Ls is approximately 2.05128. What is the average time a unit spends in this system? Select one: a. approximately 0.8013 b. approximately 0.4103 c. Cannot be calculated because λ is larger than μ. d. approximately 0.2083 e. approximately 0.1603

In an M/M/1 queueing system, the arrival rate is 5 customers per hour and the service rate is 7 customers per hour. What is the expected number of customers in the system (L)? (Round your answer to 3 decimal places.)  What is the expected waiting time in the system (W)? (Express the waiting time in hours, round your answer to 3 decimal places.) What is the expected number of customers in the queue(Lq)? (Round your answer to 3 decimal places.) What is the expected waiting time in the queue(Wq)? (Express the waiting time in hours, round your answer to 3 decimal places.)

In improving services to consumers, Limited Company Prima conducted a study of the queue time spent by consumers during the payment process with one available payment service.The average arrival of consumers in 1 hour is 8 consumers, while every hour the number of customers who can be served is 10 consumers. Count: a. The average length of time the customer is in the storeb. Average number of consumers who are being servedc. If the waiter fee is 500 and the subscription fee is waiting for 100, calculate the hourly amount

Ali Baba‘s Car Wash Service Centre is open 6 days a week, but its busiest day isalways on Sunday. From the previous data, Ali Baba estimates that dirty carsarrive at the rate of one every two minutes. One car at a time is cleaned in thisexample of a single-channel waiting line. Assuming Poisson arrivals andexponential service times, find the following: a) Compute the average number of cars in line.

Consider a bank branch that has three distinct customer arrival patterns throughout the day, as measured by average arrival rates (below). Morning (8:30 - 11:30): arrival 1 = 47 per hour. %3D Lunch (11:30 - 1:30): arrival 2 = 70 per hour. Afternoon (1:30 - 4:00): arrival 3 = 30 per hour. Regardless of the time of day, the average time it takes for a teller to serve customers is 3.17 minutes. Because of competition with other banks in the area, management has developed an internal goal to keep the average customer wait before service to be less than 4 minutes. With that in mind, answer the following: a. During the morning period, what is the minimum number of tellers that the bank needs to hire to achieve the 4-minute service goal mentioned above? [ Select] b. During lunch, what is the minimum number of tellers that the bank needs to hire to achieve the 4 minute service goal mentioned above? [ Select ] c. In the afternoon, what is the minimum number of tellers that the bank

Which of the following waiting line systems does not use the FCFS service discipline to serve people? Options:   a fast food restaurant   a hospital emergency room   a fashion retailer   a gas station

Describe the important operating characteristics of a queuing system. Be sure to provide examples to illustrate your understanding of these concepts.

A car mechanic, Carlos, can install new mufflers at an average rate of 3 every hour (following an exponential distribution). Customers needing this service arrive at the shop (following a Poisson distribution) on an average of 2 per hour. They are served on a first-in, first-out basis and come from the very large (almost infinite) population of possible buyers. Calculate the following operating characteristics of the service system: a. The probability there are no cars in the system. b. The average number of cars waiting in line. c. The average number of cars in the system. d. The average time a car spends waiting in line. e. The average time a car spends in the system.