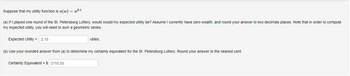
Suppose that my utility function is u(w) = ⁰.1 (a) If I played one round of the St. Petersburg Lottery, would would my expected utility be? Assume I currently have zero wealth, and round your answer to two decimals places. Note that in order to compute my expected utility, you will need to sum a geometric series. Expected Utility = 2.15 utiles. (b) Use your rounded answer from (a) to determine my certainty equivalent for the St. Petersburg Lottery. Round your answer to the nearest cent. Certainty Equivalent = $ 2110.50

answer this properly, remember (0.35x50)+(0.65x10)=£24 here

Prospect Y = ($6, 0.25 ; $15, 0.75) If Will's utility of wealth function is given by u(x)=x0.25, what is the value of CE(Y) for Will? (In other words, what is Will's certainty equivalent for prospect Y?) (The certainty equivalent represents the maximum amount a person would be willing to pay to acquire a risky prospect, and equivalently, the lowest price for which they would be willing to sell a risky prospect if they already owned it) (Note: The answer may not be a whole number; please round to the nearest hundredth) (Note: The numbers may change between questions, so read carefully)

1 Q1. Jerry has wealth of $60 and derives utility from this according to the utility function U(w) = 1 - Where w is his wealth. Jerry now finds a lottery ticket (the drawing takes place the next day) that offers a 50% chance of winning $5. W a) What is the expected value of Jerry if he takes the lottery ticket? (pay attention, it's not jerry's wealth) b) What is the minimum amount for which Jerry would be willing-to-sell the ticket? (Hint: sets a price of p, and at the minimum amount, the expected utility of selling and not selling should be the same) c) Which is bigger, your answer to (a) or (b), and suggest whether Jerry is a risk averse person based on the previous conclusion? d) If he does not sell the ticket, what is Jerry's cost of risk? (The cost of risk is the difference between the expected wealth and the certainty equivalence)

Priyanka has an income of £90,000 and is a von Neumann-Morgenstern expected utility maximiser with von Neumann-Morgenstern utility index . There is a 1 % probability that there is flooding damage at her house. The repair of the damage would cost £80,000 which would reduce the income to £10,000. a) Would Priyanka be willing to spend £500 to purchase an insurance policy that would fully insure her against this loss? Explain. b) What would be the highest price (premium) that she would be willing to pay for an insurance policy that fully insures her against the flooding damage?

Max Pentridge is thinking of starting a pinball palace near a large Melbourne university. His utility is given by u(W) = 1 - (5,000/W), where W is his wealth. Max's total wealth is $15,000. With probability p = 0.9 the palace will succeed and Max's wealth will grow from $15,000 to $x. With probability 1 - p the palace will be a failure and he’ll lose $10,000, so that his wealth will be just $5,000.  What is the smallest value of x that would be sufficient to make Max want to invest in the pinball palace rather than have a wealth of $15,000 with certainty? (Please round your final answer to the whole dollar, if necessary)