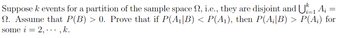
= Suppose k events for a partition of the sample space , i.e., they are disjoint and Ut_1 Ai < P(A₁), then P(A¿|B) > P(A;) for №. Assume that P(B) > 0. Prove that if P(A₁|B) some i 2.....k.

Consider the binomial distribution with n trials and PS) - p. (a) Show that Ply) Ply - 1) (n - y+1)p for y - 1, 2,..., n. Equivalently, for y- 1, 2, .., n, the equation plv) - M-Y+ 1)P ply - 1) gves a recursive relationship between the probabilities assodated with successive values of Y. Ply - 1) " pr-g- -1) - In-y+ 1)p (b) fn- 70 and p- 0.04, use the above relationship to find PY ply - 1) if y is smalI (y (n + 1) p). Thus, successive binamial probabilities increase for a while and decrease from then on. (c) Show that ya (n + 1)p > y (n +1- vp >y+ (n +1- vo> Your answer cannot be undenstood or graded. More Information (n+1-yIP> ya plv) Ply - 1) (n + 1) p. Show that (n + 1lp Ply - 2) >.. Also for y 2 (n + 1p, then ply) a > ply + 2) >.... Thus it is clear that p(y) is madimized when y is as clase to pas possible.