Question
Sketch the electric field lines of the parallel plate capacitor and the variation of the following parameters as a function of position x inside the capacitor: electric potential produced by the capacitor, potential energy of the proton, total energy of the proton, and kinetic energy of the proton.
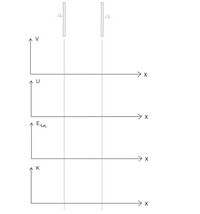
Transcribed Image Text:Exot
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 4 steps with 1 images

Knowledge Booster
Similar questions
- The graph in the figure shows the variation of the electric potential V (measured in volts) as a function of the radial direction T (measured in meters), For which range or value of T is the magnitude of the electric field the largest? (m) O from r = 3 m to r= 4 m from T=0 m to r= 1 m from r = 1 m to r= 2 m O at r= 4 m O at r = 3 m from T = 6 m to r= 8 m O from T = 4 m to r= 6 m Submit Previous Answers Request Answerarrow_forwardA certain parallel-plate capacitor is filled with a dielectric for which κ = 6.26. The area of each plate is 0.0573 m2, and the plates are separated by 2.35 mm. The capacitor will fail (short out and burn up) if the electric field between the plates exceeds 164 kN/C. What is the maximum energy that can be stored in the capacitor?arrow_forwardSuppose an electron orbits a proton in a spherical orbit. Does the electric potential energy of the electron-proton system change as the electron moves, or does it remain constant?arrow_forward
- A parallel-plate capacitor has a capacitance of 570 pF, a plate area of 400 cm², and a mica dielectric (K = 5.40) completely filling the space between the plates. At 53.0 V potential difference, calculate (a) the electric field magnitude E in the mica, (b) the magnitude of the free charge on the plates, and (c) the magnitude of the induced surface charge on the mica. (a) Number (b) Number i M. (c) Number i jeda Units Units Unitsarrow_forwardProblem 18: The electric potential in a certain region is given by the equation V(x,y,z) = 3αx2y3 - 2γx2y4z2 where the potential is in volts when the positions are given in meters. Part (d) The constants in this equation are α = 5.2 V/m5 and γ = 1.39 V/m8. Using this information, calculate the magnitude of the electric field at the point (x1,y1,z1) = (-5.0, 2.0, 1.5) m in units of newtons per coulomb.arrow_forwardPP7arrow_forward
arrow_back_ios
arrow_forward_ios