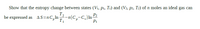
Show that the entropy change between states (V1, pı, T1) and (V2, p2, T2) of n moles an ideal gas can T2 be expressed as AS=nC,In-n(C,-C,)In2 P1

Calculate the efficiency of the reversible cycle in figure for an ideal monoatomic gas where the transformation from 1->2 is described by the equation P2 V = A= constant (Assume V2 = 4 V1). P 3 1 V1 2 V2 V Hint: You might need to use the following integral: = √ + GA little bit of a problem can be found for Q1-2 = AU + W where the integral above may result useful to know.

1 Point i in Fig. 20-19 represents the initial state of an ideal gas at temperature T. Taking algebraic signs into account, rank the entropy changes that the gas undergoes as it moves, successively and reversibly, from point i to points a, b, c, and d, greatest first. T+AT T-AT Volume Pressure

Two moles of an ideal gas undergo an irreversible isothermal expansion fromVa=100 liters to Vb=300 liters at T=300K.(a)  What is the entropy change for this process?(b) What is the Gibbs free energy change?(c) Is this process spontaneous or not? Explain your answer.