Represent the following decimal numbers in binary using 8-bit signed magnitude, one's complement, two's complement, and excess-127 representations: Decimal +38 -54 SM 00101010 11110101 OC 01000100 11110101 TC 00001111 11110101 Excess-127 10001111 11110101 ■
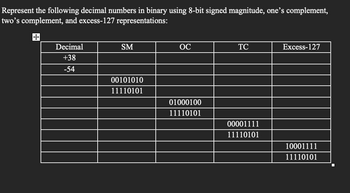
Signed Magnitude:
When a number is expressed in sign-magnitude form, the MSB is used to indicate the number's sign, while the remaining bits reflect its magnitude. Therefore, simply add the sign bit to the leftmost side of an unsigned binary number. This representation is comparable to that of signed decimal numbers.
1's complement:
By complementing each bit in a signed binary number, one can derive the 1's complement of a given number. Therefore, a positive number multiplied by one produces a negative number. The complement of a negative number, 1, yields a positive number. The original signed binary number can be obtained by doing two times the 1's complement of a binary number that includes the sign bit.
2's complement:
One is added to the 1's complement of a signed binary number to produce the binary number's 2's complement. The result is a negative number when a positive number is multiplied by two. Similar to this, adding two to a negative number results in a positive number. The original signed binary number can be obtained by performing two times the 2's complement of a binary number that includes the sign bit.
Excess 127:
The excess-M form of a signed integer yields the unsigned binary value by simply adding M to the signed integer. – The number 010, for instance, is represented as 0 + 7 = 710 = 01112, assuming that we're using excess-7 representation. – The symbol for the number 310 is 3 + 7 (1010 = 10102).
Using the above procedure we can calculate each field in the table...
Step by step
Solved in 2 steps
