Related questions
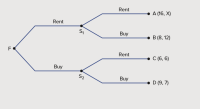
Examine the following game tree. Fred and Sally are planning on running competing restaurants. Each must decide whether to rent space or buy space. Fred goes first at decision node F. Sally goes second at either decision node S1 or decision node S2 (depending on what Fred chose to do at decision node F). Note that the payoff to Sally at terminal node A is X. a. If X < 12, what terminal node will the subgame perfect Nash equilibrium path lead to? b. If X > 12, what terminal node will the subgame perfect Nash Equilibrium path lead to? c. Suppose that X = 11 and that it is now possible for Fred to make a side payment of value V to Sally that will boost her payout at terminal node A from X = 11 to X = 11 + V. What is the minimum amount that V can be such that the subgame perfect Nash equilibrium path will lead to terminal node A? Assume that V can take on only discrete units (0, 1, 2, 3,…).
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps
- Consider the following game. You will roll a fair, 6-sided die either once or twice. You decide whether to do the second roll after you see how the first one lands. The payoff is $n, where n is the outcome of the last roll. For example, if the first roll lands 4 and the second lands 2, you win $2. If you only do one roll and it lands 4, you win $4. Suppose you make your decision about whether to go for a second roll based on expected monetary value. Then you will go for a second roll if (and only if) the first roll lands x or lower. What is x?arrow_forwardO Cell A O Cell C O Cell E O Cell I None of the abovearrow_forwardNonearrow_forward
- Jane is interested in buying a car from a used car dealer. Her maximum willingness to pay for thecar is 12 ($12,000). Bo, the dealer, is willing to sell the car as long as he receives at least 9($9,000). What is the Nash bargaining solution to this game?arrow_forward1.a) If the three executives of a fraudulent organization report nothing to the authorities, each gets a payoff of 100. If at least one of them blows the whistle, then those who reported the fraud get 28, while those who didn’t get -100. Suppose they play a symmetric mixed-strategy Nash equilibrium where each is silent (does not report fraud) with probability p. What is p?A, 0.1B, 0.28C, 0.5D, 0.8 b) In a two-player game, with strategies and (some known and some unknown) payoffs as shown below, suppose a mixed-strategy equilibrium exists where 1 plays C with probability 3/4, and Player 2 randomizes over X, Y, and Z with equal probabilities. What are the pure-strategy equilibria of this game? A, (A, Y) and (B, X)B, (A, Z) and (C, Y)C, (B, X) and (C, X)D, (C, X) and (C, Y)arrow_forward
Principles of Economics (12th Edition)EconomicsISBN:9780134078779Author:Karl E. Case, Ray C. Fair, Sharon E. OsterPublisher:PEARSON
Engineering Economy (17th Edition)EconomicsISBN:9780134870069Author:William G. Sullivan, Elin M. Wicks, C. Patrick KoellingPublisher:PEARSON
Principles of Economics (MindTap Course List)EconomicsISBN:9781305585126Author:N. Gregory MankiwPublisher:Cengage Learning
Managerial Economics: A Problem Solving ApproachEconomicsISBN:9781337106665Author:Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike ShorPublisher:Cengage Learning
Managerial Economics & Business Strategy (Mcgraw-...EconomicsISBN:9781259290619Author:Michael Baye, Jeff PrincePublisher:McGraw-Hill Education