MATLAB: An Introduction with Applications
6th Edition
ISBN: 9781119256830
Author: Amos Gilat
Publisher: John Wiley & Sons Inc
expand_more
expand_more
format_list_bulleted
Related questions
Question
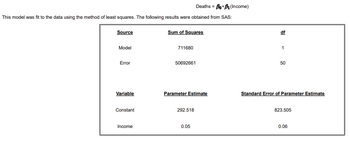
Please help me understand this problem more in depth. A researcher is investigating possible explanations for deaths in traffic accidents. He examined data from 2000 for each of the 52 cities randomly selected in the US. The data included information on the following variables: Deaths: The number of deaths in traffic accidents per city Income: The
Question: Based on the above results, the researcher tested the hypotheses: Ho: B1=0 versus B1 not equal to 0, versus using T test. What do we know about the test statistic of the test? Based on the approximate p-value, what's the conclusion?
Transcribed Image Text:Deaths = Bo+B₁ (Income)
This model was fit to the data using the method of least squares. The following results were obtained from SAS:
Sum of Squares
Source
Model
Error
Variable
Constant
Income
711680
50692661
Parameter Estimate
292.518
0.05
df
1
50
Standard Error of Parameter Estimate
823.505
0.06
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 5 images
Knowledge Booster
Similar questions
- KidsFeet Regression Line y-Intercept The KidsFeet dataframe contains data collected on 39 fourth grade students in Ann Arbor, MI, in October 1997. Two of the measurements taken on the children were the length in centimeters, (length), and width in centimeters, (width), of their longest foot. This data could be used to answer the following Research Question: How is the width of a fourth-grade student's foot related to the length? Which of the following is the y-intercept of the regression line? ## ## Simple Linear Regression## ## Correlation coefficient r = 0.6411 ## ## Equation of Regression Line:## ## length = 9.817 + 1.658 * width ## ## Residual Standard Error: s = 1.025 ## R^2 (unadjusted): R^2 = 0.411 ( ) 1.0248 ( ) 1.6576 ( ) 22.8153 ( ) 0.411 ( ) 9.8172 ( )0.6411arrow_forwardConsider the following passage: I ran a regression, with many variables to predict the result of another variable which was the murder rate. One can see that lots of things, can cause the murder rate to increase or decrease. I tried to account for all the important factors, and those factors are the SAT scores, unemployment rate, and international migration per 1,000. The SAT score is, average combined total score participants did on the SAT exam. The unemployment rate is, "a measure of the prevalence of unemployment and it is calculated as a percentage by dividing the number of unemployed individuals by all individuals currently in the labor force." (Wikipedia) International migration per 1,000 is, the number of people who come into a state from other countries per 1,000 people who live in the state. After I run the regression I will look at the t scores and p values and I should hopefully conclude that international migration does not cause crime. Which writing mistakes, if any, did…arrow_forwardThis is only one questionarrow_forward
- The manager of the Bayville police department motor pool wants to develop a forecast model for annual maintenance on police cars, based on mileage in the past year and age of the cars. The following data have been collected for eight different cars: a. Using Excel, develop a multiple regression equation for these data. b. What is the coefficient of determination for this regression equation? c. Forecast the annual maintenance cost for a police car that is 5 years old and will be driven 10,000 miles in 1 year.arrow_forwardWhat is the difference between the following two regression equations? y=b0+b1x y=B0+B1xarrow_forwardSir Francis Galton, in the late 1800 s, was the first to introduce the statistical concepts of regression and correlation. He studied the relationships between pairs of variables such as the size of parents and the size of their offspring. Data similar to that which he studied are given below, with the variable x denoting the height (in centimeters) of a human father and the variable y denoting the height at maturity (in centimeters) of the father's oldest (adult) son. The data are given in tabular form and also displayed in the scatter plot below, which gives the least-squares regression line as well. The equation for this line is y =95.35 +0.48 x Height of Height of son, father, x (in centimeters) centimeters) y (in 175.8 178.1 193.5 190.3 210. 183.2 176.6 173.3 171.1 200- 183.8 186.5 190. 175.8 175.6 180 159.8 167.2 xx 170 155.5 175.8 162.0 172.1 160- 190.9 188.0 150 180.1 187.1 Tdo ito Ido 130 200 z1o 186.9 177.5 171.5 181.5 Height of father, x (in centimeters) 190.5 195.7 201.0…arrow_forward
- Hiroshi Sato, an owner of a sushi restaurant in San Francisco, has been following an aggressive marketing campaign to thwart the effect of rising unemployment rates on business. He used monthly data on sales ($1,000s), advertising costs ($), and the unemployment rate (%) fromJanuary 2008 to May 2009 to estimate the following sample regression equation: Sales(t) = 17.51 +0.05 Advertising Costs(t-1) – 0.70 Unemployment Rate t-1 Requirement: a. Hiroshi had budgeted $620 toward advertising costs in May 2009. Make a forecast in June2009, if the unemployment rate in May 2009 was 9.1%b. What will be the forecast if he raises his advertisement budget to $700?c. Reevaluate the above forecast if the unemployment rate in May 2009 was 9.5%. Please, if possible, can you do the answer in Excel/Spreadsheetarrow_forwardA social scientist would like to analyze the relationship between educational attainment (in years of higher education) and annual salary (in $1,000s). He collects data on 20 individuals. A portion of the data is as follows: Salary Education 34 3 66 1 ⋮ ⋮ 33 0 Click here for the Excel Data File a. Find the sample regression equation for the model: Salary = β0 + β1Education + ε. (Round answers to 2 decimal places.) Salaryˆ=Salary^= + Education b. Interpret the coefficient for Education. multiple choice As Education increases by 1 year, an individual’s annual salary is predicted to increase by $4,690. As Education increases by 1 year, an individual’s annual salary is predicted to decrease by $4,690. As Education increases by 1 year, an individual’s annual salary is predicted to increase by $8,590. As Education increases by 1 year, an individual’s annual salary is predicted to decrease by $8,590.…arrow_forwardConsider the following passage: I ran a regression, with many variables to predict the result of another variable which was the murder rate. One can see that lots of things, can cause the murder rate to increase or decrease. I tried to account for all the important factors, and those factors are the SAT scores, unemployment rate, and international migration per 1,000. The SAT score is, average combined total score participants did on the SAT exam. The unemployment rate is, "a measure of the prevalence of unemployment and it is calculated as a percentage by dividing the number of unemployed individuals by all individuals currently in the labor force." (Wikipedia) International migration per 1,000 is, the number of people who come into a state from other countries per 1,000 people who live in the state. After I run the regression I will look at the t scores and p values and I should hopefully conclude that international migration does not cause crime. Which writing mistakes, if any, did…arrow_forward
- According to an article, one may be able to predict an individual's level of support for ecology based on demographic and ideological characteristics. The multiple regression model proposed by the authors was the following. y = 3.60-.01.x₁ +.01.x2-.07x3+.12x4+.02xs-.04x6-.01x7.04x8-.02xg+e The variables are defined as follows. y = ecology score (higher values indicate a greater concern for ecology) x₁ = age times 10 x₂ = income (in thousands of dollars) x3 = gender (1 = male, 0 = female) X4 = race (1 = white, 0 = nonwhite) X5 = education (in years) x6 = ideology (4 = conservative, 3 = right of center, 2 = middle of the road, 1 = left of center, and 0 = liberal) X7 = social class (4 = upper, 3 = upper middle, 2 = middle, 1 = lower middle, 0 = lower) x8 = postmaterialist (1 if postmaterialist, 0 otherwise) x9 = materialist (1 if materialist, O otherwise) (a) Suppose you knew a person with the following characteristics: a 30 year old, white female with a college degree (20 years of…arrow_forwardA Moving to another questiof! Question 27 ry .dock Provide an appropriate response. In order for applicants to work for the foreign-service department, they must take a test in the language of the country where they plan to work. The data below shows the relationship between the number of years that applicants have studied a particular language and the grades they received on the proficiency exam. Find the equation of the regression line for the given data. DOCK tigation n.Lab Number of years, x Grades on test, y O - 6.910x- 46.261 reen Shot --05...3.58 PM O = 46.261x + 6.910 O = 46.261x -6.910 O - 6,910x + 46.261 Sterling's Daily Food Log.pdt W A Moving to another question will save this response. arling's lntakeS Question 27 of 28 > Goals Ans and Outs of Energy (1).docx Screen Shot 22-059.03 AM Screen 2022-05 AM Screen Shot x 2022-05.9.31 AM 2022-05.0.09 Screen Shoarrow_forwardIn this section, we introduced a descriptive measure of the utility of the regression equation for making predictions. a. Identify the term and symbol for that descriptive measure. b. Provide an interpretation.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning 
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
MATLAB: An Introduction with Applications
Statistics
ISBN:9781119256830
Author:Amos Gilat
Publisher:John Wiley & Sons Inc
Probability and Statistics for Engineering and th...
Statistics
ISBN:9781305251809
Author:Jay L. Devore
Publisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...
Statistics
ISBN:9781305504912
Author:Frederick J Gravetter, Larry B. Wallnau
Publisher:Cengage Learning
Elementary Statistics: Picturing the World (7th E...
Statistics
ISBN:9780134683416
Author:Ron Larson, Betsy Farber
Publisher:PEARSON
The Basic Practice of Statistics
Statistics
ISBN:9781319042578
Author:David S. Moore, William I. Notz, Michael A. Fligner
Publisher:W. H. Freeman
Introduction to the Practice of Statistics
Statistics
ISBN:9781319013387
Author:David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:W. H. Freeman