Question
Just part e
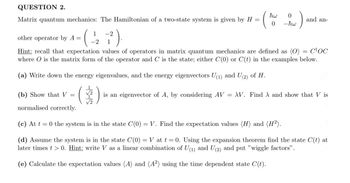
Transcribed Image Text:QUESTION 2.
Matrix quantum mechanics: The Hamiltonian of a two-state system is given by H =
other operator by A =
(
1 -2
-2 1
(
hw
0
and an-
0 -ħw
Hint: recall that expectation values of operators in matrix quantum mechanics are defined as (0) = COC
where O is the matrix form of the operator and C is the state; either C(0) or C(t) in the examples below.
(a) Write down the energy eigenvalues, and the energy eigenvectors U(1) and U(2) of H.
(b) Show that V
=
(
is an eigenvector of A, by considering AV = XV. Find A and show that V is
normalised correctly.
(c) At t = 0 the system is in the state C(0) = V. Find the expectation values (H) and (H2).
(d) Assume the system is in the state C(0) = V at t = 0. Using the expansion theorem find the state C(t) at
later times >0. Hint: write V as a linear combination of U(1) and U(2) and put "wiggle factors".
(e) Calculate the expectation values (A) and (A2) using the time dependent state C(t).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 3 images
