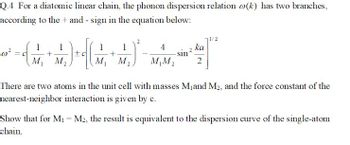
Q.4 For a diatomic linear chain, the phonon dispersion relation o(k) has two branches, according to the+ and - sign in the equation below: 0² 1 1 (+) M₁ M₂ =C 1 + M₁ M₂. 2 1/2 ka 쌀² 4 M₁M₂ 2 sin ² There are two atoms in the unit cell with masses M₁ and M₂, and the force constant of the nearest-neighbor interaction is given by c. Show that for M₁ = M2, the result is equivalent to the dispersion curve of the single-atom chain.

Consider the function v(1,2) =( [1s(1) 3s(2) + 3s(1) 1s(2)] [x(1) B(2) + B(1) a(2)] Which of the following statements is incorrect concerning p(1,2) ? a. W(1,2) is normalized. Ob. The function W(1,2) is symmetric with respect to the exchange of the space and the spin coordinates of the two electrons. OC. y(1,2) is an eigenfunction of the reference (or zero-order) Hamiltonian (in which the electron-electron repulsion term is ignored) of Li with eigenvalue = -5 hartree. d. The function y(1,2) is an acceptable wave function to describe the properties of one of the excited states of Lit. Oe. The function 4(1,2) is an eigenfunction of the operator S,(1,2) = S;(1) + S,(2) with eigenvalue zero.

Show that the minimum cnergy of a simple harmonic oscillator is Fw/2 if ArAp = h/2, where (Ap)² = ((p - (p))?). %3D

please answer c) only 2. a) A spinless particle, mass m, is confined to a two-dimensional box of length L. The stationary Schrödinger equation is - +a) v(x, y) = Ev(x, y), for 0 < r, y < L. The bound- ary conditions on ý are that it vanishes at the edges of the box. Verify that solutions are given by 2 v(1, y) sin L where n., ny = 1,2..., and find the corresponding energy. Let L and m be such that h'n?/(2mL²) = 1 eV. How many states of the system have energies between 9 eV and 24 eV? b) We now consider a macroscopic box (L of order cm) so that h'n?/(2mL?) ~ 10-20 eV. If we define the wave vector k as ("", ""), show that the density of states g(k), defined such that the number of states with |k| between k and k +dk is given by g(k)dk, is Ak 9(k) = 27 c) Use the expression for g(k) to show that at room temperature the partition function for the translational energy of a particle in a macroscopic 2-dimensional box is Z1 = Aoq, where 2/3 oq = ng = mk„T/2nh?. Hence show that the average…

A real wave function is defined on the half-axis: [0≤x≤00) as y(x) = A(x/xo)e-x/xo where xo is a given constant with the dimension of length. a) Plot this function in the dimensionless variables and find the constant A. b) Present the normalized wave function in the dimensional variables. Hint: introduce the dimensionless variables = x/xo and Y(5) = Y(5)/A.

c) How does the classical kinetic energy of the free electron compare in magnitude with the result you obtained in the previous part?

Problem 3. Consider the two example systems from quantum mechanics. First, for a particle in a box of length 1 we have the equation h² d²v 2m dx² EV, with boundary conditions (0) = 0 and (1) = 0. Second, the Quantum Harmonic Oscillator (QHO) V = EV h² d² 2m da² +ka²) 1 +kx² 2 (a) Write down the states for both systems. What are their similarities and differences? (b) Write down the energy eigenvalues for both systems. What are their similarities and differences? (c) Plot the first three states of the QHO along with the potential for the system. (d) Explain why you can observe a particle outside of the "classically allowed region". Hint: you can use any state and compute an integral to determine a probability of a particle being in a given region.

The infinitisimal work done when extending a rod is given by SW = fdL. Which of the following Maxwell relations is derived from the internal energy? Select one: a. Ob. ² C. ○ d. Ꭲ (5) S - (355) f (F), = (²), T (²7) ₂ = ( 5 ) ₁ as S (2/²)₁ = − ( ³ ) ₁