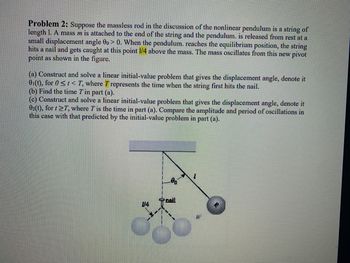
Problem 2: Suppose the massless rod in the discussion of the nonlinear pendulum is a string of length 1. A mass m is attached to the end of the string and the pendulum. is released from rest at a small displacement angle 00> 0. When the pendulum. reaches the equilibrium position, the string hits a nail and gets caught at this point 1/4 above the mass. The mass oscillates from this new pivot point as shown in the figure. (a) Construct and solve a linear initial-value problem that gives the displacement angle, denote it 01(t), for 0≤t< T, where T represents the time when the string first hits the nail. (b) Find the time Tin part (a). (c) Construct and solve a linear initial-value problem that gives the displacement angle, denote it 02(t), for t≥T, where T' is the time in part (a). Compare the amplitude and period of oscillations in this case with that predicted by the initial-value problem in part (a). 00 nail U4

Problem 20.03 A proton traveling at 4.40 km/s suddenly enters a uniform magnetic field of 0.750 T, traveling at an angle of 55.0° with the field lines (see (Figure 1)). Figure 55.0⁰ Ł Proton B < 1 of 1 ▾ Part B Find the magnitude of the force this magnetic field exerts on the proton. Express your answer in newtons to three significant figures. ΑΣΦ F= Submit ▾ Part C Fmax = Submit V Part D 4 Request Answer If you can vary the direction of the proton's velocity, find the magnitude of the maximum force you could achieve. Express your answer in newtons to three significant figures. [V] ΑΣΦ ^ ? Request Answer 13 ? N ⒸE ? If you can vary the direction of the proton's velocity, find the magnitude of the minimum force you could achieve. Express your answer in newtons to three significant figures. VG] ΑΣΦ | € P Pearson N

3 Consider a uniform source of particles inside a box with dx=8 cm , dy=4 cm, and dz=2 cm, centered on the origin. Use hand calculation to model each of the sources (3D, 2D-rectangular x-y, 1D linear x, point at origin) and compare the total flux at distances of 1, 2, 64, and 256 cm above from the origin (in the z direction).

Answer all parts of the physics problem please