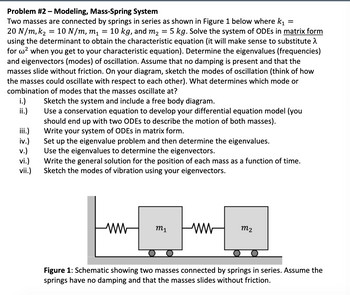
Problem #2 - Modeling, Mass-Spring System = 10 N/m, m₁ = Two masses are connected by springs in series as shown in Figure 1 below where k₁ = 20 N/m, k₂ 10 kg, and m₂ 5 kg. Solve the system of ODEs in matrix form using the determinant to obtain the characteristic equation (it will make sense to substitute > for w² when you get to your characteristic equation). Determine the eigenvalues (frequencies) and eigenvectors (modes) of oscillation. Assume that no damping is present and that the masses slide without friction. On your diagram, sketch the modes of oscillation (think of how the masses could oscillate with respect to each other). What determines which mode or combination of modes that the masses oscillate at? i.) ii.) iii.) iv.) v.) vi.) vii.) Sketch the system and include a free body diagram. Use a conservation equation to develop your differential equation model (you should end up with two ODEs to describe the motion of both masses). Write your system of ODEs in matrix form. Set up the eigenvalue problem and then determine the eigenvalues. Use the eigenvalues to determine the eigenvectors. Write the general solution for the position of each mass as a function of time. Sketch the modes of vibration using your eigenvectors. www m₁ ww M2

Please help me doing part B all I need help with is too make the derivation of equations of motion, and derivation of the state equations, and that will do for part B if you could help me with this it would make my life alot easier, and no matlab is not necessary for this.

Fundamental.7 deals with the equation of motion. The use of the equation of motion to solve this problem is mandatory. Solution using other approaches (conservation of energy...) will be automatically considered false. For the problem related to Fundamental.7 sketches of the system showing: • the respective velocity and acceleration and the frame of reference considered • the forces acting on the system of considered, in other words, a free body diagram (FBD) are mandatory. Their absences will automatically make the problem false. Z Lft 0 The 6-lb man lies against the cushion for which the coefficient of static friction is 0.7. The angle the cushion has is 56° Determine the smallest angular velocity he rotates about the z-axis, at L=9-ft from G, to ensure the man will not slip.

Scenario Mechatronics is an integrated and interdisciplinary approach to engineering design. A leading supplier and systems integrator of highly dynamic, automated logistics solutions for warehousing, production, picking and distribution operations is looking for a resident engineer to provide electrical, mechanical and software solutions. As the first stage in the interview process, you need to show your understanding of electrical mechanical models and components. Derive an equation relating the input force F, with the output, displacement x for the mechanical system shown * Sketch the response that you would expect for this system and state what happens as c is altered. What type of system response is this V F(t) Derive the relationship between output, the p.d. across the capacitor, and the input v R с Sketch the response that you would expect for this system and explain the importance of the time constant to this response? What type of system response is this?

i just need part 3

From 1.31 1.32 and 1.33 show all work

2- Derive the rule-of-mixtures expression for the composite extensional modulus E₁ assuming the existence of an interphase region. The starting point for the derivation would be the model shown below. For simplicity, assume the interphase, like the matrix, is isotropic with modulus E¹. With an interphase region there is a volume fraction associated with the interphase (i.e.,V;). For this situation: vf + vm + vi = 1 H |w²||wm|

Q5/ A beam with a length L is attached to the wall with a cable as shown. A load W = 400 lb. is attached to the beam. The tension force, T, k in the cable is given by: T = WL√h²+x² hx For a beam with L= 120 in. and h = 50 in. calculate T for x = 10, 30, 50, 70, 90, and 110 in. MATLAB D W

4) Find the transfer function for the system shown below. For full credit, simplify so that the numerator and denominator do not contain fractions. G3 Y(s) R(s) (Σ) 3. G1 G2 Σ G2 H2 H1