
Please answer all sub parts
On average, Americans have lived in 2 places by the time they are 18 years old. Is this average less for college students? The 70 randomly selected college students who answered the survey question had lived in an average of 1.78 places by the time they were 18 years old. The standard deviation for the survey group was 0.8. What can be concluded at the αα = 0.01 level of significance?
- For this study, we should use
- The null and alternative hypotheses would be:
H0:H0:
H1:H1:
- The test statistic = (please show your answer to 3 decimal places.)
- The p-value = (Please show your answer to 4 decimal places.)
- The p-value is αα
- Based on this, we should the null hypothesis.
- Thus, the final conclusion is that ...
- The data suggest that the population
mean is not significantly less than 2 at αα = 0.01, so there is statistically insignificant evidence to conclude that the population mean number of places that college students lived in by the time they were 18 years old is less than 2. - The data suggest that the sample mean is not significantly less than 2 at αα = 0.01, so there is statistically insignificant evidence to conclude that the sample mean number of places that college students lived in by the time they were 18 years old is less than 1.78.
- The data suggest that the populaton mean is significantly less than 2 at αα = 0.01, so there is statistically significant evidence to conclude that the population mean number of places that college students lived in by the time they were 18 years old is less than 2.
- Interpret the p-value in the context of the study.
- There is a 1.22145686% chance that the population mean number of places that college students lived in by the time they were 18 years old is less than 2.
- There is a 1.22145686% chance of a Type I error.
- If the population mean number of places that college students lived in by the time they were 18 years old is 2 and if you survey another 70 college students, then there would be a 1.22145686% chance that the sample mean for these 70 college students would be less than 1.78.
- If the population mean number of places that college students lived in by the time they were 18 years old is 2 and if you survey another 70 college students, then there would be a 1.22145686% chance that the population mean number of places that college students lived in by the time they were 18 years old would be less than 2.
- Interpret the level of significance in the context of the study.
- If the population mean number of places that college students lived in by the time they were 18 years old is less than 2 and if you survey another 70 college students, then there would be a 1% chance that we would end up falsely concluding that the population mean number of places that college students lived in by the time they were 18 years old is equal to 2.
- If the population mean number of places that college students lived in by the time they were 18 years old is 2 and if you survey another 70 college students, then there would be a 1% chance that we would end up falsely concluding that the population mean number of places that college students lived in by the time they were 18 years old is less than 2.
- There is a 1% chance that none of this is real since you have been hooked up to virtual reality since you were born.
- There is a 1% chance that the population mean number of places that college students lived in by the time they were 18 years old is less than 2.
- The data suggest that the population
Since you have posted a question with multiple sub-parts, we will solve first three sub-
parts for you. To get remaining sub-part solved please repost the complete question and
mention the sub-parts to be solved
(1)
Determine the type of the study used.
The type of the study used is determined below as follows:
Since, the value of the population standard deviation is unknown the type of the study used is” single mean t-test”.
(2)
State the hypotheses.
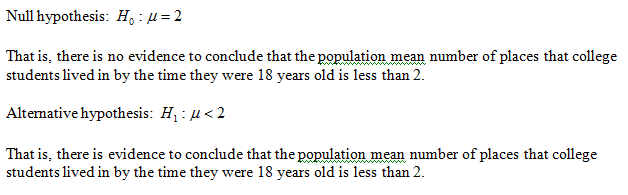
Step by stepSolved in 2 steps with 2 images

- I've shown the excel outline for the question!arrow_forwardWhat does it mean when you have standard deviations of 27 35 and 32 and its effect on the validitity of the data you are accessing to interpret for statistical anaylsis?arrow_forwardThe manufacturer of a particular brand of tires claims they average at least 50,000 miles before needing to be replaced. From past studies of this tire, it is known that the population standard deviation is 8,000 miles. A survey of tire owners was conducted. From the 28 tires surveyed, the mean lifespan was 46500 miles. Using alpha = 0.05, can we prove that the data in inconsistent with the manufacturers claim? We should use a test. What are the correct hypotheses? H0: Ha: Based on the hypotheses, find the following:Test Statistic=p-value= The correct decision is to . The correct conclusion would be:arrow_forward
- On average, Americans have lived in 2 places by the time they are 18 years old. Is this average more for college students? The 66 randomly selected college students who answered the survey question had lived in an average of 2.08 places by the time they were 18 years old. The standard deviation for the survey group was 0.5. What can be concluded at the a = 0.10 level of significance? a. For this study, we should use Select an answer b. The null and alternative hypotheses would be: Но: ? v Select an answer v H1: ? v| Select an answer v c. The test statistic ? v = (please show your answer to 3 decimal places.) d. The p-value = (Please show your answer to 4 decimal places.) %3D e. The p-value is ? v a f. Based on this, we should Select an answer v the null hypothesis. g. Thus, the final conclusion is that ... The data suggest that the populaton mean is significantly more than 2 at a = 0.10, so there is statistically significant evidence to conclude that the population mean number of…arrow_forwardOne way to check on how representative a survey is of the population from which it was drawn is to compare various characteristics of the sample with the population characteristics. A typical variable used for this purpose is age. The GSS 2018 found a mean age of 48.69 and a standard deviation of 17.99 for its sample of 1,495 American adults. Assume that we know from census data that the mean age of all American adults is 37.80. What is the research and the null hypotheses for a two-tailed test of means, calculate the t statistic and test the null hypothesis at the .001 significance level, and what is the decision about the null hypothesis?arrow_forwardPLEASE ONLY SELECT THE ANSWERS AS SHOWN FOR THE MULTIPLE CHOICE, SUCH AS OPTION 1, 2, ETC. 1) In a test of the effectiveness of garlic for lowering cholesterol, 49 subjects were treated with raw garlic. Cholesterol levels were measured before and after the treatment. The changes (before minus after) in their levels of LDL cholesterol (in mg/dL) have a mean of 0.8 and a standard deviation of 1.51. Use a 0.10 significance level to test the claim that with garlic treatment, the mean change in LDL cholesterol is greater than 0. What do the results suggest about the effectiveness of the garlic treatment? Assume that a simple random sample has been selected. Identify the null and alternative hypotheses, test statistic, P-value, and state the final conclusion that addresses the original claim. What are the null and alternative hypotheses? A. H0: μ=0 mg/dL H1: μ<0 mg/dL B. H0: μ>0 mg/dL H1: μ<0 mg/dL C. H0: μ=0 mg/dL H1: μ≠0 mg/dL D. H0: μ=0 mg/dL H1: μ>0 mg/dL…arrow_forward
- I will send the rest of question in a bit because of your guidelinesarrow_forwardDo shoppers at the mall spend more money on average the day after Thanksgiving compared to the day after Christmas? The 52 randomly surveyed shoppers on the day after Thanksgiving spent an average of $122. Their standard deviation was $29. The 59 randomly surveyed shoppers on the day after Christmas spent an average of $117. Their standard deviation was $35. What can be concluded at the αα = 0.05 level of significance? For this study, we should use The null and alternative hypotheses would be: H0:H0: H1:H1: The test statistic = (please show your answer to 3 decimal places.) The p-value = (Please show your answer to 4 decimal places.) The p-value is αα Based on this, we should the null hypothesis.arrow_forward
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman





