
Concept explainers
Nine spheres, each with a mass of 3.2 kg, are distributed evenly along a semicircle of radius r = 2.8 meters, as shown in the diagram above. The diameter of each sphere is much smaller than the radius of the semicircle. For purposes of a coordinate system, the center of the semicircle is at the origin of our coordinate system, and the entire semicircle is in the first and second quadrants (x coordinates range from -r to r meters, while y coordinates range from 0 to r meters.
Find the location of the y coordinate (in meters) of the center of mass of the nine spheres.
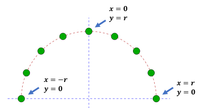
The centre of semicircle is at the origin of our coordinate system
θ
Radius of semicircle (r) =2.8 metre is given.
y0 = 0
y1 = = 2.8 sin (22.5) = 1.0715
y2 = rsin = 2.8 sin ( 2 x 22.5) = 1.98
y3 = rsin = 2.8 sin (3 x 22.5) = 2.58
y = r = 2.8 (given)
Step by stepSolved in 2 steps

- A surveyor measures the distance across a straight river by the following method: Starting directly across from a tree on the opposite bank, he walks x- 110 m along the riverbank to establish a baseline. Then he sights across to the tree. The angle from his baseline to the tree is 0 = 31.2°. How wide is the river? y =arrow_forwardSolve the following problem using analytical techniques: suppose you walk 15.0 m straight west and then 26.5 m straight north. How far are you from your starting point and what is the compass direction of a line connecting your starting point to your final position ? Give the direction in degrees north of west. (Magnitude and direction )arrow_forwardTwo points are given in polar coordinates by (r, ?) = (2.60 m, 50.0°) and (r, ?) = (3.00 m, −42.0°), respectively. What is the distance between them?arrow_forward
- The x and y coordinates of point P are (-8.50 m, -7.00 m). What is the polar angle of point P?arrow_forwardFind the Cartesian coordinates for the point whose distance from the origin is 7 m and the angle measured from the positive horizontal axis is 15°. x=? y=?arrow_forwardA wall clock has a minute hand with a length of 0.46 m and an hour hand with a length of 0.26 m. Take the center of the clock as the origin, and use a Cartesian coordinate system with the positive x axis pointing to 3 o'clock and the positive y axis pointing to 12 o'clock. Write the vector that describes the displacement of a fly if it quickly goes from the tip of the minute hand to the tip of the hour hand at 3:00 P.M. (Let D represents the displacement of the fly.) D = 0.46 |× mî+ -0.26 Let the +y direction be straight up and the +x direction be to the right. Recall the definition of displacement, and remember that positions are vector quantities.arrow_forward
- A plane is flying at a speed of 320 miles per hour on a bearing of N65°E. Its ground speed is 390 miles per hour and its true course, given by the direction angle of the ground speed vector, is 30°. Find the speed, in miles per hour, and the direction angle, in degrees, of the wind. The speed of the wind is miles per hour. (Do not round until the final answer. Then round to the nearest tenth as needed.)arrow_forwardJack goes for a ride on a ferris wheel that has a radius of 37 meters. The center of the ferris wheel is 49 meters above the ground. He starts his ride at the 12 o'clock position and travels counter clockwise. Define a function g that represents Jack's horizontal distance in meters relative to vertical midline of the wheel in terms of the angle (measured in radians) Jack makes with the center.arrow_forwardOn the map, let the +x-axis point east and the +y-axis north. An airplane flies at 880 km/h northwestward direction (i.e., midway between north and west). Can you help me visualize the components of the first velocity, and when the plane flies due south at the same velocity. I understand the answer will be -622.25 for the run, meaning 880*cos(45) for x -axis; the y axis north is 880*sin(45) resulting in 622.5 Please help me visualize the first and second velocity when the plane flies due south at the same velocity? Help me to visualize where one of the velocities due south is zero, and the other is whatever it is. I am afraid I can properly visualize or understand in simple terms what is asked. My point can you help to understand the right angle theorem to the angle.arrow_forward
- Hiker Harry walks 4 km east then 7 km 25o north of west. What is the magnitude and direction of Harry’s position after his hike as measured from his starting point? Give the angle measured relative to east. For example 90° means directly north.arrow_forwardWhat is the magnitude, X (in m2), of the cross product of a vector of magnitude 7.2 m vector pointing east and a vector of magnitude 4.4 m pointing 25° west of north? Enter only the number, not the units belowarrow_forwardYou are on a treasure hunt and your map says "Walk due west for 75.8 paces, then walk 60.0° north of west for 71.5 paces, and finally walk due north for 21.2 paces." What is the magnitude of the component of your displacement in the direction (a) due north and (b) due west? (a) Number i (b) Number C Units Units B W # + A E Startarrow_forward
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON





