Media periodically discuss the issue of heights of winning presidential candidates and heights of their main opponents. The accompanying table lists the heights (cm) from several recent presidential elections. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value ofr. Determine whether there is sufficient evidence to support a claim of linear correlation between the two variables. Should we expect that there would be a correlation? Use a significance level of a = 0.05. Click the icon to view the heights of the candidates. Construct a scatterplot. Choose the correct graph below. OA. 200 160+ 724 POK 160 200 President Height (cm) Q 2 The linear correlation coefficientis r=- (Round to three decimal places as needed.) Determine the null and alternative hypotheses. Ho: P H₁: p (Type integers or decimals. Do not round.) The test statistic is t= (Round to two decimal places as needed.) The P-value is (Round to three decimal places as needed.) Because the P-value of the linear correlation coefficient is Should we expect that there would be a correlation? O B. 200 160+ 160 29 199 200 President Height (cm) ² OA. No, because presidential candidates are nominated for reasons other than height. OB. No, because height is the main reason presidential candidates are nominated. OC. Yes, because height is the main reason presidential candidates are nominated. OD. Yes, because presidential candidates are nominated for reasons other than height. Q G the significance level, there O C. Candidate Heights 200 160 KFT ‒‒‒‒ 160 200 President Height (cm) 2 O D. President 180 181 183 180 179 181 189 178 176 185 190 191 183 189 Opponent 175 179 177 176 183 178 177 182 185 180 172 186 186 172 6 200 HOPK OBE **** ++++ 160 200 President Height (cm) sufficient evidence to support the claim that there is a linear correlation between the heights of winning presidential candiates and the heights of their opponents. X 160+ Q Q G
Media periodically discuss the issue of heights of winning presidential candidates and heights of their main opponents. The accompanying table lists the heights (cm) from several recent presidential elections. Construct a scatterplot, find the value of the linear correlation coefficient r, and find the P-value ofr. Determine whether there is sufficient evidence to support a claim of linear correlation between the two variables. Should we expect that there would be a correlation? Use a significance level of a = 0.05. Click the icon to view the heights of the candidates. Construct a scatterplot. Choose the correct graph below. OA. 200 160+ 724 POK 160 200 President Height (cm) Q 2 The linear correlation coefficientis r=- (Round to three decimal places as needed.) Determine the null and alternative hypotheses. Ho: P H₁: p (Type integers or decimals. Do not round.) The test statistic is t= (Round to two decimal places as needed.) The P-value is (Round to three decimal places as needed.) Because the P-value of the linear correlation coefficient is Should we expect that there would be a correlation? O B. 200 160+ 160 29 199 200 President Height (cm) ² OA. No, because presidential candidates are nominated for reasons other than height. OB. No, because height is the main reason presidential candidates are nominated. OC. Yes, because height is the main reason presidential candidates are nominated. OD. Yes, because presidential candidates are nominated for reasons other than height. Q G the significance level, there O C. Candidate Heights 200 160 KFT ‒‒‒‒ 160 200 President Height (cm) 2 O D. President 180 181 183 180 179 181 189 178 176 185 190 191 183 189 Opponent 175 179 177 176 183 178 177 182 185 180 172 186 186 172 6 200 HOPK OBE **** ++++ 160 200 President Height (cm) sufficient evidence to support the claim that there is a linear correlation between the heights of winning presidential candiates and the heights of their opponents. X 160+ Q Q G
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
Related questions
Question
100%
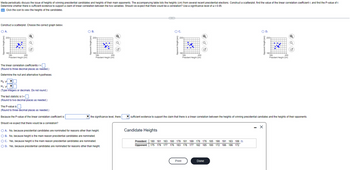
Transcribed Image Text:The text discusses the analysis of the heights of winning presidential candidates compared to their main opponents. The task involves constructing a scatterplot from the given height data to determine any linear correlation between the two variables: the height of the winning candidate and the height of their opponents. The significance level is set at α = 0.05.
### Steps to Perform the Analysis:
1. **Construct a Scatterplot**:
- Choose the correct graph from options A, B, C, or D. Each graph plots the opponent's height (y-axis) against the president’s height (x-axis).
2. **Calculate the Linear Correlation Coefficient (r)**:
- Input the value of \( r \) and round to three decimal places.
3. **Formulate Hypotheses**:
- Null Hypothesis (H₀): \( \rho = 0 \)
- Alternative Hypothesis (H₁): \( \rho \neq 0 \)
4. **Determine the Test Statistic (t)**:
- Input the test statistic value and round to two decimal places.
5. **Identify the P-Value**:
- Input the P-value of the test and round to three decimal places.
6. **Decision Rule**:
- Compare the P-value with the significance level to decide whether there is sufficient evidence for a linear correlation.
7. **Interpretation of Results**:
- Discuss whether the model expects a correlation, considering whether height is a factor in presidential candidate nomination.
### Candidate Heights Data:
- **President Heights (cm)**: 180, 181, 183, 180, 179, 181, 179, 176, 185, 190, 191, 183, 189
- **Opponent Heights (cm)**: 175, 179, 177, 176, 177, 185, 180, 172, 186, 186, 172
### Conclusion Options for Correlation Expectation:
A. No, because presidential candidates are nominated for reasons other than height.
B. No, because height is the main reason presidential candidates are nominated.
C. Yes, because height is the main reason presidential candidates are nominated.
D. Yes, because presidential candidates are nominated for reasons other than height.
Through this exercise, learners will understand how to analyze data for correlation using scatterplots, calculate correlation coefficients, and make inferences using statistical tests.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 3 images
Recommended textbooks for you
MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc
Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning
Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning
MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc
Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning
Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning
Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON
The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman
Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman