Let Q₁ = 1 μC, Q2 = 5 μC, and Q3 = -6 μC. (a) Find the electric field at the location of Q1, due to Q₂ and Q3. N/C (b) Use the electric field you calculated in part (a) to find the force on Q1- N TH (c) Find the electric field at location A, due to all three charges. N/C TE F 1 cm F--- 2₁ Q3
Let Q₁ = 1 μC, Q2 = 5 μC, and Q3 = -6 μC. (a) Find the electric field at the location of Q1, due to Q₂ and Q3. N/C (b) Use the electric field you calculated in part (a) to find the force on Q1- N TH (c) Find the electric field at location A, due to all three charges. N/C TE F 1 cm F--- 2₁ Q3
Related questions
Question
Needs Complete typed solution with 100 % accuracy. Otherwise skip if you can't give complete solution.
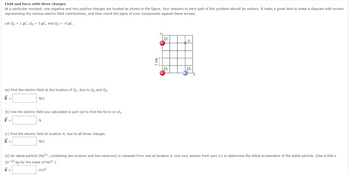
Transcribed Image Text:Field and force with three charges
At a particular moment, one negative and two positive charges are located as shown in the figure. Your answers to each part of this problem should be vectors. It helps a great deal to make a diagram with arrows
representing the various electric field contributions, and then check the signs of your components against these arrows.
Let Q₁ = 1 μC, Q₂ = 5 μC, and Q3 = -6 μC.
(a) Find the electric field at the location of Q₁, due to Q₂ and Q3.
E =
N/C
(b) Use the electric field you calculated in part (a) to find the force on Q₁.
F =
N
(c) Find the electric field at location A, due to all three charges.
E =
N/C
1 cm
F---
Q₁
Q2
A
Q3
(d) An alpha particle (He²+, containing two protons and two neutrons) is released from rest at location A. Use your answer from part (c) to determine the initial acceleration of the alpha particle. (Use 6.646 ×
10-27 kg for the mass of He²+.)
a =
m/s²
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 4 steps with 6 images
