Hm, and let № be anomalous of G. Codes following: 1. embeddings show that if Na normal subgroup of G. then the inchesion map N→→G an embedding of N into G. Specifically, prove that is injective and that it preserves the group structure. 2. (Quotients) Let G/N be the quotient group formed by the cosets of N in G. Prove that the quotient map : G G/N, defined by (9) N. it a surjection and show that it is a homomorphiam. 3. (Comparison) Show that G/N is isomorphic to the image of the map : N G under the condition that (N) normal m G. and uxplain the dillerence between embeddings and quotients in this context. 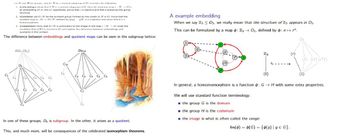The difference between embeddings and quotient maps can be seen in the subgroup lattice: A example embedding When we say 3D3, we really mean that the structure of 23 appears in D3. This can be formalized by a map : Z3D3, defined by : nr. AGL1(Z5) C10 Dic 10 CA Co Co Ca 23 (f) (rf) (r²f) c (0) (1) C2 C2 C2 In one of these groups, D5 is subgroup. In the other, it arises as a quotient. This, and much more, will be consequences of the celebrated isomorphism theorems. In general, a homomomorphism is a function : G H with some extra properties. We will use standard function terminology: ■the group G is the domain the group H is the codomain ■the image is what is often called the range: Im() = (G) = {(9)|gЄG}.

11. Find all normal subgroups of the alternating group .

Let G be the group and H the subgroup given in each of the following exercises of Section 4.4. In each case, is H normal in G? Exercise 3 b. Exercise 4 c. Exercise 5 d. Exercise 6 e. Exercise 7 f. Exercise 8 Section 4.4 Let H be the subgroup e, of the octic group D4. Find the distinct left cosets of H in D4, write out their elements, partition D4 into left cosets of H, and give [D4:H]. Find the distinct right cosets of H in D4, write out their elements, and partition D4 into right cosets of H. Let H be the subgroup e, of the octic group D4. Find the distinct left cosets of H in D4, write out their elements, partition D4 into left cosets of H, and give [D4:H]. Find the distinct right cosets of H in D4, write out their elements, and partition D4 into right cosets of H. Let H be the subgroup e, of the octic group D4. Find the distinct left cosets of H in D4, write out their elements, partition D4 into left cosets of H, and give [D4:H]. Find the distinct right cosets of H in D4, write out their elements, and partition D4 into right cosets of H. Let H be the subgroup (1),(2,3) of S3. Find the distinct left cosets of H in S3, write out their elements, partition S3 into left cosets of H, and give [S3:H]. Find the distinct right cosets of H in S3, write out their elements, and partition S3 into right cosets of H. In Exercises 7 and 8, let G be the multiplicative group of permutation matrices I3,P3,P32,P1,P4,P2 in Example 6 of Section 3.5 Let H be the subgroup of G given by H=I3,P4={ (100010001),(001010100) }. Find the distinct left cosets of H in G, write out their elements, partition G into left cosets of H, and give [G:H]. Find the distinct right cosets of H in G, write out their elements, and partition G into right cosets of H. Let H be the subgroup of G given by H=I3,P3,P32={ (100010001),(010001100),(001100010) }. Find the distinct left cosets of H in G, write out their elements, partition G into left cosets of H, and give [G:H]. Find the distinct right cosets of H in G, write out their elements, and partition G into right cosets of H.

22. If and are both normal subgroups of , prove that is a normal subgroup of .