find the coefficient of kinetic friction between the oblique order and the body?
Q: As shown in the figure below, a box of mass m = 66.0 kg (initially at rest) is pushed a distance d =…
A: Hey dear using the formula for work done we can easily solve this
Q: As shown a worker poling a boat—a very efficient mode of transportation—across a shallow lake. He…
A: Free body diagram F is the reaction force exerted on the boat due to the lake as given by Newton’s…
Q: As shown in the figure below, a box of mass m = 50.0 kg (initially at rest) is pushed a distance d =…
A: Hello. Since your question has multiple sub-parts, we will solve the first three sub-parts for you.…
Q: A student is skateboarding down a ramp that is 6.95 m long and inclined at 24.1° with respect to the…
A:
Q: An incline makes an angle of 30.0 degrees with the horizontal. (Static friction coefficent of the…
A:
Q: vel Flg. 6-23 Problems 16 and 22. 17 In Fig. 6-24, a force P acts on a block weighing 45 N. The…
A: Given data: The weight of the block is W=45 N Initial velocity u=0 m/s. Angle of inclination is…
Q: A 6.53-kg block initially at rest is pulled to the right along a horizontal surface by a constant…
A:
Q: y initial Final m V m d
A:
Q: Two blocks, with masses m and 3m, are attached to the ends of a string with negligible mass that…
A: please see the next step for solution
Q: Problem 15: A cube of mass m is initially at rest at the highest point of an inclined plane which…
A:
Q: A 10 kg-block moves on a smooth horizontal surface with a speed of 10 m/s and slides into another…
A: Given data: Mass = 10 kg Initial speed = 10 m/s Coefficient of friction = 0.25 Need to determine the…
Q: no mass). When the 1.0 kg mass hangs vertically undisturbed it is in contact with a second block…
A:
Q: A skier traveling 13.2m/s reaches the foot of a steady upward 19.70975 incline and glides 20.29696m…
A:
Q: A force P = 873 kN whose line of action passes through points A(1, 1, 4) and B(5, 8, 10). Determine…
A: We write vectors in unit vector notations where units vectors are along x y and z directions. We…
Q: As shown in the figure below, a box of mass m = 66.0 kg (initially at rest) is pushed a distance d =…
A:
Q: A truck is traveling at 11.1m / s down a hill when the brakes on all four wheels lock. The hill…
A: Initial speed = V0 = 11.1 m/sFinal speed = V = 0 m/sAngle = 15 degrees
Q: An applied force of 40.7 N directed at an angle of -18.5 degrees with respect to the +x direction…
A: According to the work-energy theorem, the change in kinetic energy of the mass will be equal to the…
Q: Determine the Value of Total Energy about Point A TEa= Determine the Value of Total Energy about…
A: Mass of the automobile Speed of automobile at point A Speed of automobile at point B For…
Q: If the coefficient of kinetic friction is 0.06 between a 2.26 block and an icy surface, find the…
A:
Q: A 29-N block slides down a 23 degrees inclined plane with an initial velocity of 2.6 m/s. Determine…
A:
Q: H As shown in the figure below, a box of mass m = 69.0 kg (initially at rest) is pushed a distance d…
A: Since you have posted a question with multiple sub-parts, we will solve first three sub-parts for…
Q: A safety mechanism of a lift is simplified by the figure below. A 300 kg block is to slide over an…
A: We will answer the question by identifying the forces in the system. The detailed steps are given…
Q: he designer of a ski resort wishes to have a portion of a beginner's slope on which the…
A:
Q: As shown in the figure below, a box of mass m = 51.0 kg (initially at rest) is pushed a distance d =…
A:
Q: A man pushing a crate of mass m = 92.0 kg at a speed of v = 0.875 m/s encounters a rough horizontal…
A:
Q: As shown In the figure below, a box of mass m = 37.0 kg is sliding along a horizontal frictionless…
A:
Q: As shown in the figure below, a box of mass m = 67.0 kg (initially at rest) is pushed a distance d =…
A: As you have already got the answer to sub-parts (a, b, and c), we will solve the remaining sub-parts…
Q: An object is on earth with a mass of 10.0 kg at the top of a frictionless inclined plane of length…
A: ...
Q: A 1.850 kg block of wood rests on a steel desk. The coefficient of static friction between the block…
A: Mass = 1.850 kg μs = 0.555μk = 0.305 F = 6.19 N
Q: A child of mass 15.0-kg skis from rest straight down a nursery slope. Since the child has no idea…
A:
Q: A SEWAGE WORKER is inside a large underground aqueduct (diameter >> a man's height) of circular…
A:
Q: A crate of mass M is initially at rest at the highest point of an inclined plane, which has a height…
A: mass of crate = m Height (h) = 5.8 m θ = 27ov = 0.35 m/s coefficient of friction on incline =…
Q: As shown in the figure below, a box of mass m = 54.0 kg (initially at rest) is pushed a distance d =…
A:
Q: dAB Vf A bale of cardboard to be recycled is thrown down an incline (0 = 25°) at A with a velocity…
A:
Q: A skier starts from rest at the top of a hill that is inclined at 11.0° with respect to the…
A:
Q: As shown in the figure below, a box of mass m = 54.0 kg (initially at rest) is pushed a distance d =…
A:
Q: A student is skateboarding down a ramp that is 5.02 m long and inclined at 23.6° with respect to the…
A:
Q: A crate of mass mm is initially at rest at the peak of an inclined plane which has a height of…
A:
Q: A blick of mass m is initially at rest at the highest point of an inclined plane, which has a height…
A:
Q: As shown in the figure below, a box of mass m = 67.0 kg (initially at rest) is pushed a distance d =…
A: "As per the guidelines i solved the first three subpart". (a) The work done by the applied force is,…
Q: Problem 4: A crate of mass m is initially at rest at the highest point of an inclined plane, which…
A: Draw the free-body diagram of the crate.
Q: A block, initially at rest, has a mass m and sits on a plane inclined at angle theta. It slides a…
A:
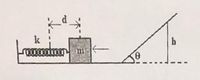
an object with mass m shown in the figure is attached to a spring with a spring constant k in a horizontal arrangement without friction, and the object is compressed as much as d. After the system is released, the object can rise to the height of h in the friction inclined plane. find the coefficient of kinetic friction between the oblique order and the body?
Step by step
Solved in 3 steps
