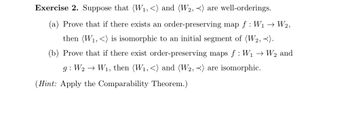
Exercise 2. Suppose that (W₁, <) and (W2, <) are well-orderings. (a) Prove that if there exists an order-preserving map f: W₁W2, then (W₁,<) is isomorphic to an initial segment of (W2, <). (b) Prove that if there exist order-preserving maps f: W₁ → g: W2 → W₁, then (W₁, <) and (W2, <) are isomorphic. (Hint: Apply the Comparability Theorem.) W2 and

Suppose T: V --> W is a linear and surjective map and V and W are of finite dimension. Show that T has a linear right inverse, i.e., there is a linear map S: W --> V so that the composition T of S = Id. Explain your answer.

2. Suppose that G is a lincar map such that G(2,0) = (4, 0) and G(0, 3) = (–3, 9). Find the images of: (a) G(1,0) (b) G(1, 1) (c) G(2, 1)

Set theory

Suppose that T:V→W is a linear map. Prove that T is one-to-one if and only if I maps linearly independen sets in V to linearly independent sets in W.

(1) Given a subset U C V and a linear map T : V → V we say U is T-invariant if T(U) CU. Show that if U1, U2 C V are T-invariant subsets, then so is U1 + U2.

Is R considered symmetric?

1

Let f : X → Y be a map between the sets X and Y. (a) Show that f is injective if and only if there exists a map g : Y → X so that go f = idx. (b) Show that f is surjective if and only if there exists a map h : Y → X so that foh = idy.

1. Let T : R¹ → Rm be a linear transformation, and let {V₁, V2, V3} be a linearly dependent set in R. Explain why the set {T(v₁), T(v2), T(v3)} in Rm must also be linearly dependent. Remark: You argument should not be a “proof by example," that is, the argument should hold for arbitrary linear transformation T and arbitrary linearly dependent set of vectors {v₁, V2, V3} in Rº.